\(\textbf{Art 10 :} \qquad \boxed{{\text{Polar / Distance form of a line}}}\)

Sometimes, it is very convenient to write the equation of a straight line in polar / distance form. Suppose we know that the line passes through the fixed point \(P(h,\,k)\) and is at an inclination of \(\theta :\)

For any point \(Q(x,\,y)\) at a distance r from P along this line, we can write the simple relation

\[\boxed{{\frac{{x - h}}{{\cos \theta }} = \frac{{y - k}}{{\sin \theta }} = r}}\]

This is the required equation of the line. The point \(Q(x,\,y),\) at a distance r from P, has the coordinates

\[Q(x,\,y) \equiv (h + r\cos \theta ,\,k + r\sin \theta ).\]

\[\left\{ \begin{array}{l}{\text{Obviously, there will be another point, say }}Q'(x,y),{\text{ at a distance }}r{\text{ from }}P\\{\text{along this line but on the opposite side of }}Q{\text{; thus }}Q'(x,{\rm{ }}y){\text{ 
 will have the }}\\{\text{coordinates }}Q'(x,\,y) \equiv (h - r\cos \theta ,\,\,\,k - r\sin \theta)\end{array} \right\}\]

Example – 15

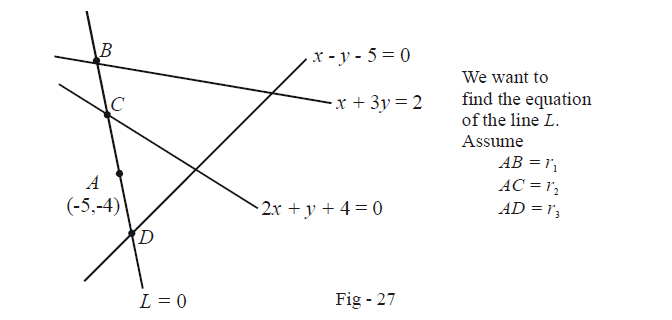
A line through \(A( - 5,\, - 4)\) meets the lines \(x + 3y = 2,\,\,2x + y + 4 = 0\) and \(x - y - 5 = 0\) at the points B, C and D respectively. If

\[\begin{align}{\left( {\frac{{15}}{{AB}}} \right)^2} + {\left( {\frac{{10}}{{AC}}} \right)^2} = {\left( {\frac{6}{{AD}}} \right)^2},\end{align}\]

find the equation of the line.

Solution:

The figure above roughly sketches the situation described in the equation. Let B, C and D be at distances \({r_1},\,{r_2}\) and \({r_3}\) from A along the line \(L = 0,\) whose equation we wish to determine. Assume the inclination of L to be \(\theta .\) Thus, B, C and D have the coordinates (respectively):

\[\begin{align}B \equiv ( - 5 + {r_1}\cos \theta ,\,\,\,\,\, - 4 + {r_1}\sin \theta )\\C \equiv ( - 5 + {r_2}\cos \theta ,\,\,\,\,\, - 4 + {r_2}\sin \theta )\\D \equiv ( - 5 + {r_3}\cos \theta ,\,\,\,\,\, - 4 + {r_3}\sin \theta )\end{align}\]

Since these three points(respectively) satisfy the three given equations, we have :

Point B : \(\begin{align}( - 5 + {r_1}\cos \theta ) + 3( - 4 + {r_1}\sin \theta ) + 2 = 0 \quad \Rightarrow \qquad {r_1} = \frac{{15}}{{\cos \theta + 3\sin \theta }}\end{align}\)

Point C : \(\begin{align}2( - 5 + {r_2}\cos \theta ) + ( - 4 + {r_2}\sin \theta ) + 4 = 0 \quad  \Rightarrow  \qquad {r_2} = \frac{{10}}{{2\cos \theta  + \sin \theta }}\end{align}\)

Point D : \(\begin{align}( - 5 + {r_3}\cos \theta ) - ( - 4 + {r_3}\sin \theta ) - 5 = 0 \quad  \Rightarrow  \qquad {r_3} = \frac{6}{{\cos \theta  - \sin \theta }}\end{align}\)

It is given that

\[\begin{align}&{\left( {\frac{{15}}{{AB}}} \right)^2} + {\left( {\frac{{10}}{{AC}}} \right)^2} = {\left( {\frac{6}{{AD}}} \right)^2}\\
\text{i.e;}\qquad \qquad \qquad  &{\left( {\frac{{15}}{{{r_1}}}} \right)^2} + {\left( {\frac{{10}}{{{r_2}}}} \right)^2} = {\left( {\frac{6}{{{r_3}}}} \right)^2}\\ \Rightarrow  \qquad &{(\cos \theta  + 3\sin \theta )^2} + {(2\cos \theta  + \sin \theta )^2} = {(\cos \theta  - \sin \theta )^2}\\ \Rightarrow  \qquad &4{\cos ^2}\theta  + 9{\sin ^2}\theta  + 12\sin \theta \cos \theta  = 0\\ \Rightarrow  \qquad &{(2\cos \theta  + 3\sin \theta )^2} = 0\\ \Rightarrow  \qquad &\tan \theta  = \frac{{ - 2}}{3}\\ \Rightarrow  \qquad &m = \frac{{ - 2}}{3}\end{align}\]

Thus, we obtain the slope of L as \(\begin{align}\frac{{ - 2}}{3}. \end{align}\) The equation of L can now be easily written :

\[\begin{align}&L:y - ( - 4) = \frac{{ - 2}}{3}(x - ( - 5))\\ \Rightarrow  \qquad &L:2x + 3y + 22 = 0\end{align}\]

TRY YOURSELF - I

Q1.  A variable straight line drawn through the intersection of the lines\(\begin{align}\frac{x}{a} + \frac{y}{b} = 1\;\;and\;\;\frac{x}{b} + \frac{y}{a} = 1\end{align}\) and meets the axes in A and B. Show that the locus of the mid-point of AB is \(2xy(a + b) = ab(x + y)\)

Q2. The line \(bx + ay = ab\) cuts the axes in A and B. Another variable line cuts the axes in C and D such that \(OA + OB = OC + OD\) where O is the origin. Prove that the locus of the point of intersection of the lines AD and BC is the line\(\;x + y = a + b\)

Q3.  A point P moves so that the square of its distance from (3, –2) is equal to its distance from the line \(5x - 12y = 13\) Find the locus of P.

Q4.  A line intersects the x-axis in A(7, 0) and the y-axis in B(0, –5). A variable line perpendicular to AB intersects the x-axis in P and the y-axis in Q. If AQ and BP intersect in R, find the locus of R.

Q5. If the sum of the distances of a point from two perpendicular lines in a plane is 1, prove that its locus is a square.

Q6.  A vertex of an equilateral triangle is (2, 3) and the opposite side is \(\;x + y = 2\).  Find the equations of the other sides.

Q7.  A ray of light along the line \(x - 2y - 3 = 0\) is incident upon the mirror-line \(3x - 2y - 5 = 0\) Find the equation of the reflected ray.

Q8. If the vertices of a triangle have integral coordinates, show that it cannot be equilateral.

Q9. Show using coordinate geometry that the angle bisectors of the sides of a triangle are concurrent.

Q10. The sides of a triangle are \(4x + 3y + 7 = 0\;,\;5x + 12y - 27 = 0\;\;and\;\;3x + 4y + 8 = 0\) and By explicitly evaluating the medians in this triangle, show that they are concurrent.

Q11. A rod APB of constant length meets the axes in A and B. If AP = b and PB = a and the rod slides between the axes, show that the locus of P is\(\;{b^2}{x^2} + {a^2}{y^2} = {a^2}{b^2}\)

Q12. If p is the length of the perpendicular from the origin to the line whose intercepts on the axes are a and b, show that \(\begin{align}\frac{1}{{{p^2}}} = \frac{1}{{{a^2}}} + \frac{1}{{{b^2}}}\end{align}\)

Q13. The lines \(3x + 4y - 8 = 0\;and\;5x + 12y + 3 = 0\) intersect in A. Find the equations of the lines passing through which intersect the given lines at B and C, such that \(AB = AC\).

Q14. The equal sides AB and AC of an isosceles triangle ABC are produced to the points P and Q such that \(BP.CQ = A{B^2}\) Prove that the line PQ always passes through a fixed point.

Q15. One side of a square is inclined to the x-axis at an angle and one of its extremities is at the origin; prove that the equations to its diagonals are

\[\begin{array}{l}
&y(\cos \alpha  - \sin \alpha ) = x(\sin \alpha  + \cos \alpha )\\\\
and\qquad &y(\sin \alpha  + \cos \alpha ) + x(\cos \alpha  - \sin \alpha ) = a
\end{array}\]

where a is the length of the side of the sqaure.