In Fig. 6.11, the value of ∠A + ∠B + ∠C + ∠D + ∠E + ∠F is
a. 190°
b. 540°
c. 360°
d. 180°
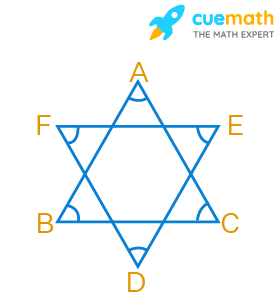
Solution:
Given, the figure represents two triangles in the form of a star.
We have to find the value of ∠A + ∠B + ∠C + ∠D + ∠E + ∠F.
The figure contains two triangles
(i) triangle ABC
(ii) triangle DEF
By angle sum property of a triangle,
We know that the sum of all the three interior angles of the triangle is equal to 180 degrees.
Considering triangle ABC,
∠A + ∠B + ∠C = 180° ------------------------------ (1)
Considering triangle DEF,
∠D + ∠E + ∠F = 180° ------------------------------ (2)
Adding (1) and (2),
∠A + ∠B + ∠C + ∠D + ∠E + ∠F = 180° + 180°
= 360°
Therefore, the required value is 360°.
✦ Try This: In ΔABC, AD bisects ∠BAC and AD = DC. If ∠ADB=100°, then find ∠ABD

☛ Also Check: NCERT Solutions for Class 7 Maths Chapter 6
NCERT Exemplar Class 7 Maths Chapter 6 Problem 22
In Fig. 6.11, the value of ∠A + ∠B + ∠C + ∠D + ∠E + ∠F is: a. 190°, b. 540°, c. 360°, d. 180°
Summary:
In Fig. 6.11, the value of ∠A + ∠B + ∠C + ∠D + ∠E + ∠F is 360°
☛ Related Questions:
- In Fig. 6.12, PQ = PR, RS = RQ and ST || QR. If the exterior angle RPU is 140°, then the measure of . . . .
- In Fig. 6.13, ∠BAC = 90°, AD ⊥ BC and ∠BAD = 50°, then ∠ACD is: a. 50°, b. 40°, c. 70°, d. 60°
- If one angle of a triangle is equal to the sum of the other two angles, the triangle is: a. Obtuse, . . . .
visual curriculum