What is the ratio of the sector area to the area of the entire circle?

Solution:

Area of sector = (𝜋r²) (θ / 360°)

Area of circle = 𝜋r²

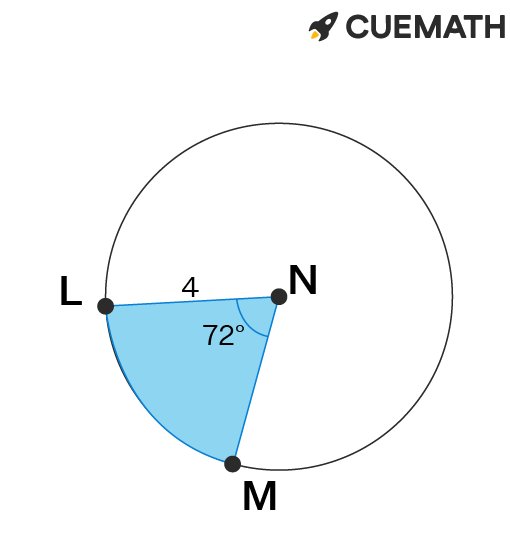
From the figure, 

Radius of circle, r = 4

Angle of sector, θ = 72°

We know that

Ratio of area of sector to area of circle = [(𝜋r²)(θ/360°)] / (𝜋r²)

By further simplification

= (θ/360°)

So we get

= 72°/360°

= 1/5

Therefore, the required ratio is 1: 5

---

What is the ratio of the sector area to the area of the entire circle?

Summary:

The ratio of the sector area to the area of the entire circle is 1: 5.