Arithmetic Mean Formula
The arithmetic mean formula calculates the mean or average of the numbers and is used to measure the central tendency of the data. It can be also be defined as the sum of all the given observations to the total number of observations. Let us study the arithmetic mean formula using solved examples.
What is Arithmetic Mean Formula?
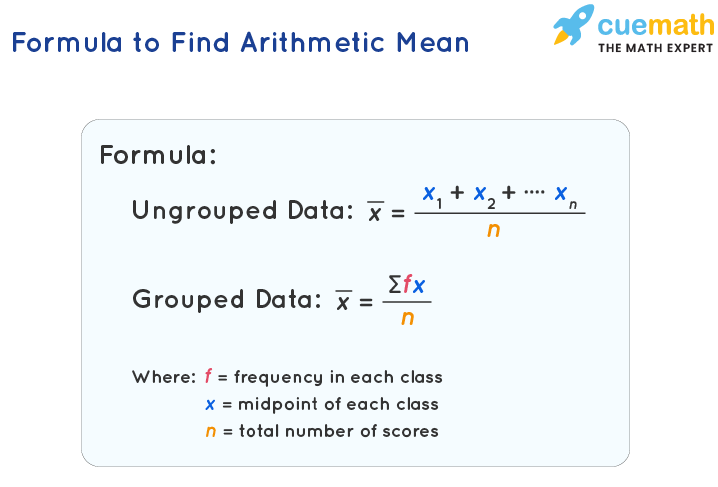
To calculate the arithmetic mean of given observations, you just simply add all the given observations and divide the resultant sum by the total number of observations. The arithmetic mean formula to calculate the mean set of observations is given as:
Arithmetic Mean Formula
Arithmetic mean is the sum of all observations divided by a number of observations.
Arithmetic mean formula = {Sum of Observation}÷{Total numbers of Observations}
Arithmetic mean formula = \(X=\Sigma \frac{\rm{X_i}}{\rm{n}}\), where i varies from 1 to n.

Examples on Arithmetic Mean Formula
Example 1: The marks obtained by 8 students in a class test are 10, 19, 12, 21, 18, 20, 11, and 19. What is the arithmetic mean of the marks obtained by the students?
Solution:
To find: Arithmetic mean of the marks obtained by the students
Using the arithmetic mean formula,
Arithmetic mean = {Sum of Observation} ÷ {Total numbers of Observations}
Arithmetic mean = (10 + 19 + 12 + 21 + 18 + 20 + 11 + 19) ÷ 8
= 16.25
Answer: Arithmetic mean of the marks obtained by the students = 16.25.
Example 2: The heights of five students are 164 cm, 134 cm, 155 cm, 156 cm, and,172 cm respectively. Find the mean height of the students.
Solution:
To find: Mean height of the students
Using the arithmetic mean formula,
Arithmetic mean = {Sum of Observation}/{Total numbers of Observations}
Arithmetic mean = (164 + 134 + 155 + 156 + 172)/5
= 781/5 = 156.2 cm
Answer: Mean height of the students = 156.2 cm.
Example 3: The mean monthly salary of 5 workers of a group is $1400. One more worker whose monthly salary is $1550 has joined the group. Find the arithmetic mean of the monthly salary of 6 workers of the group.
Solution: Here, n = 5, x̄ =1400
Using the arithmetic mean formula,
x̄ = ∑xi/n
∴∑xi = x̄ × n
∑xi = 1400 × 5 = 7000
Total salary of 5 workers = $7000
Total salary of 6 workers = $7000 + 1550 = $8550
Average salary of 6 workers = 8550/6 = 1425
Answer: ∴ Average monthly salary of 6 workers = $1425
FAQs on Arithmetic Mean Formula
What is Arithmetic Mean Formula in Statistics?
The arithmetic mean formula in statistics is defined as the sum of all observations divided by a number of observations. General arithmetic mean formula = {Sum of Observation}÷{Total numbers of Observations}.
Arithmetic mean formula in statistics = \\(X=\Sigma \frac{\rm{X_i}}{\rm{n}}\), where i varies from 1 to n.
How To Calculate the Arithmetic Mean Using Arithmetic Mean Formula?
If the set of 'n' number of observations is given then the arithmetic mean can be easily calculated by using a general arithmetic mean formula that is, Arithmetic Mean = {Sum of Observation} ÷ {Total numbers of Observations}.
How To Use the Arithmetic Mean Formula?
The general arithmetic mean formula is mathematically expressed as Arithmetic Mean = {Sum of Observation} ÷ {Total numbers of Observations}. Let us consider an example to understand how to use arithmetic mean formula.
Example: Find the arithmetic mean of (1, 2, 3, 4, 5).
Solution: Total number of observation = 5
Arithmetic mean formula = {Sum of Observation} ÷ {Total numbers of Observations}
Arithmetic Mean = (1 + 2 + 3 + 4 + 5) ÷ 5 = 15/5 = 3
Arithmetic Mean of (1, 2, 3, 4, 5) is 3
What Will Be the Arithmetic Mean Formula for n Observations?
Arithmetic mean formula for 'n' observations is expressed as, Arithmetic mean of n observations = {Sum of 'n' Observation} ÷ {Total numbers of 'n' Observations}
visual curriculum