The angles of a triangle are in the ratio 2 : 3 : 4. Find the angles of the triangle
Solution:
Given, the angles of a triangle are in the ratio 2 : 3 : 4
We have to find the smallest angle of the triangle.
We know that the sum of all three interior angles of a triangle is always equal to 180°
Let us consider a triangle ABC
∠A + ∠B + ∠C = 180°
Given, ∠A = 2x
∠B = 3x
∠C = 4x
So, 2x + 3x + 4x = 180°
5x + 4x = 180°
9x = 180°
x = 180°/9
x = 20°
Substituting the value of x to find the measure of each angle,
∠A = 2(20) = 40°
∠B = 3(20) = 60°
∠C = 4(20) = 80°
Therefore, the angles are 40°, 60° and 80°
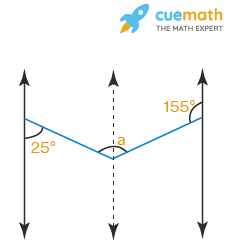
✦ Try This: In the given figure, find the measure of angle A.
☛ Also Check: NCERT Solutions for Class 9 Maths Chapter 6
NCERT Exemplar Class 9 Maths Exercise 6.3 Problem 8
The angles of a triangle are in the ratio 2 : 3 : 4. Find the angles of the triangle
Summary:
The angles of a triangle are in the ratio 2 : 3 : 4. The angles of the triangle are 40°, 60° and 80°. Since all the angles are less than 90 degrees, the given triangle is an acute angled triangle
☛ Related Questions:
- A triangle ABC is right angled at A. L is a point on BC such that AL ⊥ BC. Prove that ∠ BAL = ∠ ACB
- Two lines are respectively perpendicular to two parallel lines. Show that they are parallel to each . . . .
- In Fig. 6.15, m and n are two plane mirrors perpendicular to each other. Show that incident ray CA i . . . .
visual curriculum