Triangles

A triangle is a closed shape with 3 angles, 3 sides, and 3 vertices. A triangle with three vertices says P, Q, and R is represented as △PQR. It is also termed a three-sided polygon or trigon. In this mini-lesson, we will explore everything about triangles, which are commonly seen around us. If you observe the shape of signboards and your favorite sandwiches it forms the shape of a triangle.

A triangle consists of various parts. It has 3 angles, 3 sides, 3 vertices. Let us learn the concept with the help of a triangle figure given below. Look at the triangle PQR.

In the above image:

• The three angles are, ∠PQR, ∠QRP, and ∠RPQ.
• The three sides are side PQ, side QR, and side RP.
• The three vertices are P, Q, and R

According to two major elements, triangles can be classified as :

• On the basis of angles
• On the basis of the measurement of their sides.

Let us understand the classification of triangles with the help of the table given below. The table gives information about the difference between 6 different types of triangles on the basis of angles and sides.

Note: The sum of all the angles of the triangle is equal to 180°.

In geometry, for every two-dimensional shape, there are always two basic measurements we need to find out, i.e., the area and perimeter of that shape. Similarly, the triangle has two basic formulas which help us to determine its area and perimeter. Let us discuss the formulas in detail.

Perimeter of Triangle

The perimeter of a triangle is the sum of all three sides of the triangle. Consider △ABC.

In the above figure, the three sides of the triangle are represented as AB= c, AC = b, and CB = a respectively.
The perimeter of a triangle is the sum of all three sides AB + BC + CA.
Perimeter of Triangle formula = a + b + c units

Half of the perimeter of the triangle is termed as Semi Perimeter of Triangle. It is given as (a + b + c)/2 units.

Area of a Triangle

The area of a triangle is the space covered by the triangle. It is half the product of its base and altitude (height). It is always measured in square units, as it is two-dimensional. Look at the given triangle ABC.

Area of ΔABC = 1/2 × AD × BC square units.

Here, BC is the base and AD is the height of the triangle.

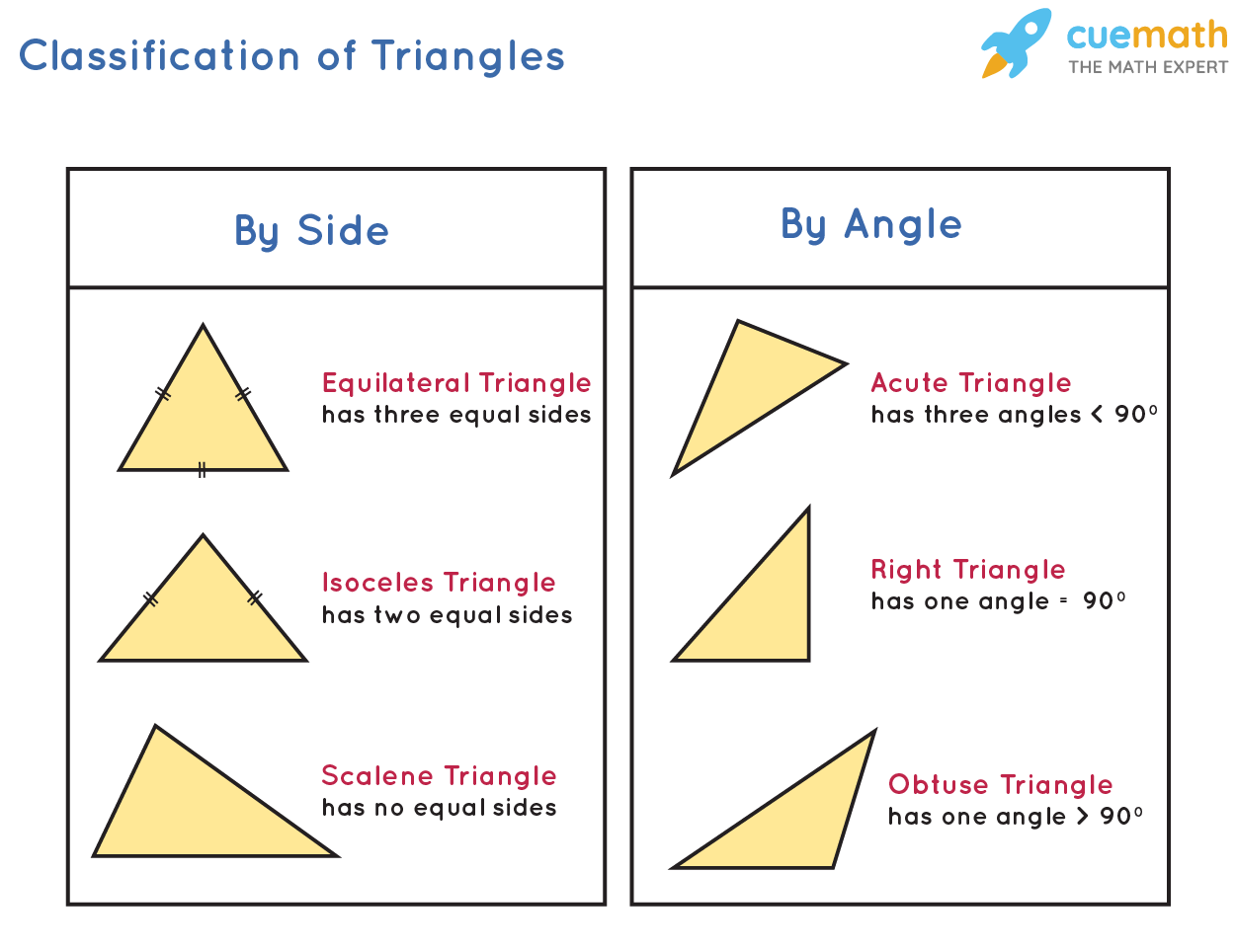
Important Notes

• A triangle cannot have a measurement or value of all the angles less than 60°.
• A triangle is a 3-sided closed shape.
• There are two important formulas related to triangles, i.e., Herons formula and Pythagoras theorem.
• The sum of the angles of a triangle adds up to 180° and given as ∠1 + ∠2 + ∠3 = 180°. 

Related Articles on Triangles

Check out these interesting articles to know more about triangles and topics related to triangles.

• Properties of Triangle
• Scalene Triangle
• Equilateral Triangle
• Right Angled Triangle
• Triangle Calculator
• Scalene Triangle Formula
• Right Triangle Calculator
• Isosceles Triangle Perimeter Formula
• Area of Isosceles Triangle
• Area of Equilateral Triangle
• Area of Right Triangle
• Area of Scalene Triangle
• Acute Triangle

Are Isosceles Triangles Always Acute?

No, an isosceles triangle can be an acute angle, right angle, or obtuse-angled triangle depending upon the measure of the angles it has.

What is the Area of Scalene Triangle?

The area of the scalene triangle is used to find the area occupied by the scalene triangle within its sides. The area of the scalene triangle is half of the product of the base to the height of the scalene triangle. Thus, the area of the scalene triangle, with a base "b" and height "h" is given as "1/2 × b × h". 

What is the Formula Used for Finding the Area of a Right Triangle?

The formula used for finding the area of a right triangle of base (b) and height (h) is 1/2 × base × height (or) 1/2 × b × h.

What is an Equilateral Triangle?

An equilateral triangle is a regular polygon (trigon) with three equal sides. Equilateral triangles also have three equal angles which measure 60 degrees each and sum up to 180 degrees. 

What is an Isosceles Triangle?

In a triangle, if the length of only two sides is equal and the measure of corresponding opposite angles is also equal, then the triangle is said to be an isosceles triangle. 

What is a Right Triangle in Geometry?

A right triangle is a triangle with one angle measurement as 90 degrees (right angle). In geometry we have three different names for all the three sides of a right triangle which are listed below:

• The hypotenuse (longest side or the side opposite to 90° angle.)
• The base
• The perpendicular (altitude).