Profit and Loss
Profit and loss are the terms used to identify whether a deal is profitable or not. We use these terms very often in our daily lives. If the selling price is greater than the cost price, then the difference between the selling price and cost price is called profit. If the selling price is less than the cost price, then the difference between the cost price and the selling price is called loss. The price at which a product is purchased is called its cost price. The price at which a product is sold is called its selling price. Let us learn more about profit and loss in this article.
| 1. | Profit and Loss Related Terms |
| 2. | Profit and Loss Formulas |
| 3. | Profit and Loss Percentage |
| 4. | FAQs on Profit and Loss |
Profit and Loss Related Terms
When a person buys an article for a certain price and then sells it for a different price, he makes a profit or incurs a loss. There are various terms that are associated with the entire process of making a transaction. For example, cost price of the article (C.P.), selling price (S.P.), discount, marked price, profit, and loss. Let us understand the meaning of these terms one by one.
Cost Price
The price at which an article is purchased is called its cost price. For example, if Neil bought an umbrella for $8, this is the cost price of the umbrella. It is abbreviated as C.P.
Selling Price
The price at which an article is sold is known as the selling price of the article. For example, if Neil sold the same umbrella for $10, then $10 is considered the selling price of the umbrella. It is abbreviated as S.P.
Profit
When, in a transaction, the selling price is greater than the cost price, it means we earn a profit. Using the above example, the profit that Neil earned is $2. It is calculated with the help of the formula: Profit = Selling price - Cost price. In the above example, the Cost price of the umbrella was $8 and the Selling price of the umbrella was $10, so the profit that he made can be calculated by using the formula: Profit = Selling price - Cost price. Substituting the values, we get, Profit = 10 - 8 = 2. Therefore, he makes a profit of $2.
Loss
When, in a transaction, the cost price is greater than the selling price, it means we incur a loss. For example, if a bag is bought for $20 and it is sold for $17, it means we incurred a loss of $3 in this transaction. Loss is calculated with the help of the formula: Loss = Cost price - Selling price. Taking the same example, the Cost price of the bag is $20 and the Selling price is $17, so the loss can be calculated with the formula: Loss = Cost price - Selling price. Substituting the values, we get, Loss = 20 - 17 = 3. Therefore, there is a loss of $3 in the transaction.
Marked Price
Marked price is the price set by the seller on the label of the article. It is a price at which the seller offers a discount. After the discount is applied on the Marked price, it is sold at a reduced price known as the selling price.
Example: Sandra goes shopping at a store where everything is at a 50% discount. The price tag on a dress is $120. This means that the Marked Price of the dress before discount = $120.
Discount
To cope with the competition in business and boost the sale of goods, shopkeepers offer discounts to customers. The rebate or the offer given by the shopkeepers to lure the customers is called a discount. Discount is always calculated on the Marked price of the article.
Discount = Marked Price - Selling Price
Discount (%) = (Discount/Marked Price) × 100
If the marked price of an article is $600, and there is a 40% discount on it, this means that the customer can buy the article at the following price:
40% discount on marked price = (40/100) × 600
Discount ($)= 24000/100 = $240
Therefore, Selling Price = Marked Price - Discount ($) = $600 − $240 = $360
Profit and Loss Formulas
Now, let us learn the formulas for calculating profit and loss.
Profit Formula
If the selling price of an article is greater than its cost price, there is a gain in the transaction. The basic formula used for calculating the profit is: Profit = Selling Price - Cost Price.
Loss Formula
If the selling price of an article is lesser than the cost price, there is a loss in the transaction. The basic formula used for calculating the loss is: Loss = Cost Price - Selling Price
Let us use these formulas and find the profit or loss in a few scenarios.
| Business | CP | SP | PROFIT/LOSS |
|---|---|---|---|
| A shopkeeper bought an article at $30 and sold it at $25. | $30 | $25 | Loss of $5 |
| A book was bought at $35 sold at $40. | $35 | $40 | Profit of $5 |
Profit and Loss Percentage
The profit percentage (%) or loss percentage (%) is calculated with the help of the following formulas, which show that the profit or loss in a transaction is always calculated on its Cost Price:
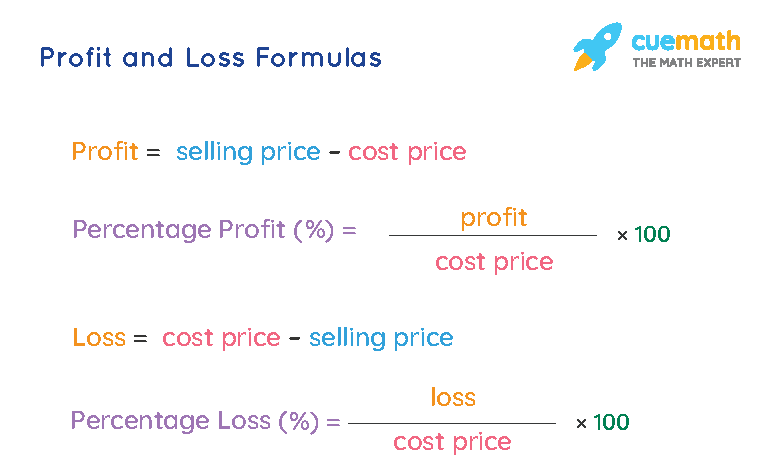
Example: If the CP of a commodity = $800 and SP = $900, then let's find the profit (%).
Profit = SP - CP= 900 − 800 = $100
Profit (%) = (Profit/CP) × 100
= (100/800) × 100
= 12.5%
Important Notes
Given below are some of the important notes related to profit and loss that we studied in this article. Have a look!
- Profit = SP - CP
- Loss = CP - SP
- Profit (%) = {Profit/CP} × 100
- Loss (%) = {Loss/CP} × 100
- Discount = Marked Price - Selling Price
- Discount (%) = (Discount/MP) × 100
Related Articles on Profit and Loss
Check out these interesting articles related to profit and loss. Click to know more!
FAQs on Profit and Loss
What is Profit and Loss in Math?
If an article is sold at a price higher than the price for which it was bought, there is a profit in the transaction. In other words, if Selling Price > Cost Price, there is a profit. If an article is sold at a price lesser than the price for which it was bought, there is a loss. It means, if Selling Price < Cost Price, there is a loss in the transaction.
How is Profit and Loss Calculated?
In order to calculate profit and loss, the cost price and the selling price of the product should be known. The basic formulas that are used to find the profit and loss are given below:
Profit = Selling Price - Cost Price
Loss = Cost Price - Selling Price
What is the Profit and Loss Percentage Formula?
In a transaction, after the profit and loss is calculated, it is converted in the form of a percentage. It should be remembered that the amount of profit or loss incurred is based on the Cost price. The formulas that are used to calculate the profit and loss percentage are given below:
- Profit percentage (P%) = (Profit /Cost Price) × 100
- Loss percentage (L%) = (Loss / Cost price) × 100
What is the Formula for Profit?
The formula used to calculate the profit in a transaction is: Profit = Selling Price - Cost Price.
What is the Formula for Loss?
The formula used to calculate the loss in a transaction is: Loss = Cost Price - Selling Price.
What is the Formula for Profit%?
The profit percentage is always calculated on the cost price. Therefore, the formula for Profit (%) is = (Profit/Cost Price) × 100.
What is the Formula for Loss%?
The loss percentage is always calculated on the cost price. Therefore, the formula for Loss (%) is = (Loss/Cost Price) × 100
What is Cost Price and Selling Price?
The price for which you buy an article is its cost price, and the price for which you sell an article is its selling price.
What does it Mean by Marked Price?
The price set by the seller before offering the discount on a commodity is called the marked price.