Simple Interest
Simple interest is a method to calculate the amount of interest charged on a sum at a given rate and for a given period of time. In simple interest, the principal amount is always the same, unlike compound interest where we add the interest to the principal to find the principal for the new principal for the next year.
In this lesson, you will be introduced to the concept of borrowing money and the simple interest that is derived from borrowing. You will also be introduced to terms such as principal, amount, rate of interest, and time period. Through these terms, you can calculate simple interest using the simple interest formula.
| 1. | What is Simple Interest? |
| 2. | Simple Interest Formula |
| 3. | How to Find Simple Interest? |
| 4. | What Types of Loans use Simple Interest? |
| 5. | Simple Interest vs Compound Interest |
| 6. | FAQs on Simple Interest |
What is Simple Interest?
Simple interest is a method of interest that always applies to the original principal amount, with the same rate of interest for every time cycle. When we invest our money in any bank, the bank provides us interest on our amount. The interest applied by the banks is of many types and one of them is simple interest. Now, before going deeper into the concept of simple interest, let's first understand what is the meaning of a loan.
A loan is an amount that a person borrows from a bank or a financial authority to fulfil their needs. Loan examples include home loan, car loan, education loan, and personal loan. A loan amount is required to be returned by the person to the authorities on time with an extra amount, which is usually the interest you pay on the loan.
Simple Interest Formula
Simple interest is calculated with the following formula: S.I. = (P × R × T)/100, where P = Principal, R = Rate of Interest in % per annum, and T = Time, usually calculated as the number of years. The rate of interest is in percentage R% (and is to be written as R/100, thus 100 in the formula). To understand more about this formula, click here.
- Principal: The principal is the amount that was initially borrowed (loan) from the bank or invested. The principal is denoted by P.
- Rate: Rate is the rate of interest at which the principal amount is given to someone for a certain time, the rate of interest can be 5%, 10%, or 13%, etc. The rate of interest is denoted by R.
- Time: Time is the duration for which the principal amount is given to someone. Time is denoted by T.
The above formula can be further solved for any variable, P, R, or T. For example, by dividing both sides of the SI formula S.I. = (P × R × T)/100 by R × T, we get P = (100 × S.I.)/(R × T). Similarly, we can solve for either R or T.
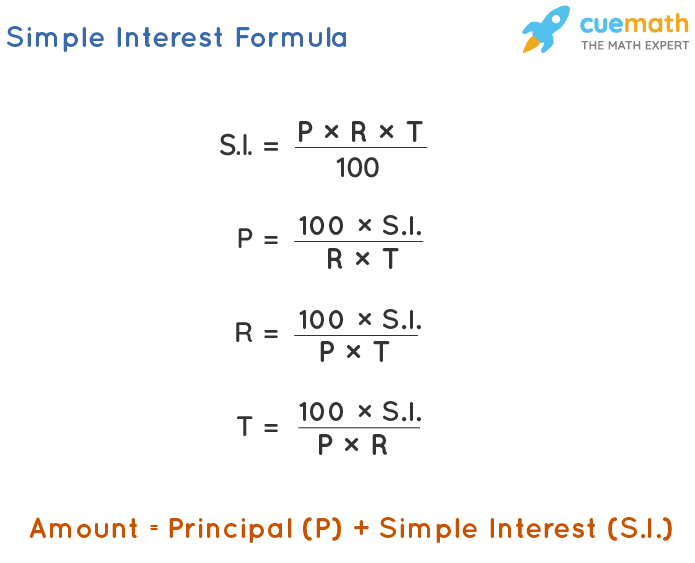
Sometimes, the simple interest formula is written as just SI = PRT where R is the rate of interest as a decimal. i.e., if the rate of interest is 5% then R can be written as 5/100 = 0.05.
Amount: When a person takes a loan from a bank, he/she has to return the principal borrowed plus the interest amount, and this total returned is called the Amount.
Amount = Principal + Simple Interest
A = P + S.I.
A = P + PRT
A = P(1 + RT)
How to Find Simple Interest?
Simple interest is found by using the formula SI = (PRT)/100 where P is the principal, R is the rate of interest, and T is the duration. The values of P, R, and T have to be substituted in this formula to calculate the simple interest. Here is an example to understand the process better for same P and R values but for different T values.
Example:
Michael's father had borrowed personal loan of $1,000 from the bank and the rate of interest was 5%. What would the simple interest be if the amount is borrowed for 1 year? Similarly, calculate the simple interest if the amount is borrowed for 2 years, 3 years, and 10 years? Also, calculate the amount that has to be returned in each of these cases.
Solution:
Principal amount = $1,000, personal loan interest rate = 5% = 5/100. (Add a sentence here describing the given information in the question.)
| Duration |
Simple Interest |
|---|---|
| 1 Year | S.I = (1000 × 5 × 1)/100 = 50 |
| 2 Year | S.I = (1000 × 5 × 2)/100 = 100 |
| 3 Year | S.I = (1000 × 5 × 3)/100 = 150 |
| 10 Year | S.I = (1000 × 5 × 10)/100 = 500 |
Now, we can also prepare a table for the above question adding the amount to be returned after the given time period.
| Simple Interest | Amount | |
|---|---|---|
| 1 Year | S.I = (1000 × 5 × 1)/100 = 50 | A = 1000 + 50 = 1050 |
| 2 Year | S.I = (1000 × 5 × 2)/100 = 100 | A = 1000 + 100 = 1100 |
| 3 Year | S.I = (1000 × 5 × 3)/100 = 150 | A = 1000 + 150 = 1150 |
| 10 Year | S.I = (1000 × 5 × 10)/100 = 500 | A = 1000 + 500 = 1500 |
What Types of Loans use Simple Interest?
Most banks these days apply compound interest on loans because in this way banks get more money as interest from their customers, but this method is more complex and hard to explain to the customers. On the other hand, calculations become easy when banks apply simple interest methods. Simple interest is much more useful when a customer wants a loan for a short period of time, for example, 1 month, 2 months, or 6 months.
When someone goes for a short-term loan using simple interest, the interest applies on a daily or weekly basis instead of a yearly basis. Consider that you borrowed $10,000 on simple interest at a 10% interest rate per year, so this 10% a year rate is divided into a rate per day which is equal to 10/365 = 0.027%. So you have to pay $2.73 a day extra on $10,000.
Simple Interest vs Compound Interest
Simple interest and compound interest are two ways to calculate interest on a loan amount. It is believed that compound interest is more difficult to calculate than simple interest because of some basic differences in both. Let's understand the difference between simple interest and compound interest through the table given below:
| Simple Interest | Compound Interest |
|---|---|
| Simple interest is calculated on the original principal amount every time. | Compound interest is calculated on the accumulated sum of principal and interest. |
| It is calculated using the following formula: S.I.= P × R × T | It is calculated using the following formula: C.I.= P × (1 +R)T - P |
| It is equal for every year on a certain principal. | It is different for every span of the time period as it is calculated on the amount and not the principal. |
Important Notes on Simple Interest:
- If the rate of interest as a percentage is used then the SI formula is (PRT)/100. But if the rate of interest is used as a decimal (i.e., if we have already divided the rate by 100) then the SI formula is just PRT.
- The rate of interest is the interest on every $100 for a fixed time period.
- Interest is always more in the case of compound interest as compared to simple interest.
- The formula or methods to calculate compound interest is derived from simple interest calculation methods.
Think Tank:
- If the interest paid by Micheal is one-fifth of the total amount he has to pay after a certain time period, what is the time period if the rate of interest was 25%?
- The interest on a sum lent at the rate of 10% per annum for a period of 20 months is equal to 1.5 times interest on $3,000 at the rate of 5% per annum in 3 years. What is the sum.
☛ Related Topics:
Simple Interest Examples
-
Example 1: Robert purchased a car worth $48,000, he borrowed the money from the bank at 10% per annum for a period of 4 years. How much amount he has to pay after the period.
Solution:
The principal value for the car is $48,000, the rate of simple interest is 10% and the time period given is 4 years.
Using the formula for amount, A= P(1 +RT), A= 48000 × (1 + 10/100 × 4)
A= 48000 × (1 + 2/5)
A= 48000 × 7/5
A= $67200
Answer: Therefore, Robert has to pay $67,200.
-
Example 2: If Maria borrowed a sum of $46,500 for a period of 21 months at 20% per annum, then find the simple interest that she will need to pay.
Solution:
The principal amount is $46,500 and the rate of interest is 20% = 20/100. The time period given is 21 months = 21/12 years. Using the simple interest formula,
SI = P × R × T
SI = 46500 × 20/100 × 21/12
SI = $16275.
Answer: Therefore, Maria is going to pay $16,275.
-
Example 3: How much money was invested at 5% annual simple interest for 4 years to earn $3500?
Solution:
Assume that principal value is P.
Rate of interest is, R = 5% = 0.05.
Time is, T = 4 years.
Amount is, A = 3500.
Using the simple interest formula of amount,
A = P (1 + RT)
3500 = P (1 + 0.05 · 4)
3500 = 1.2 P
Dividing both sides by P,
P = 3500/1.2 = 2916.67
Answer: The invested money = $ 2916.67.

FAQs on Simple Interest
What is the Definition of Simple Interest?
Simple interest is a type of interest that is calculated only on the initial amount borrowed/invested, without considering any interest charged/earned in previous periods. It is a fixed percentage of the principal amount that is charged or earned over a specific period of time.
What is the Use of Simple Interest?
Simple interest is used in cases where the amount that is to be returned requires a short period of time. So, monthly amortization, mortgages, savings calculation, and education loans use simple interest.
What are Compound Interest and Simple Interest Formulas?
For a given principal P, time T, and rate of interest R%,
- simple interest formula is PRT.
- compound interest formula is P(1 + R)T - P.
What are the Types of Simple Interests?
Simple interest is of two types ordinary simple interest and exact simple interest. In ordinary simple interest, a year is considered of 365 days while calculating the interest while in exact simple interest, a year is considered 366 days if it is a leap year. Both methods use the same formula to calculate simple interest.
Are Home Loans Simple or Compound Interest?
Home loans take a long time to repay, so the interest added by the lender is usually compound interest.
Where to Find Simple Interest Calculator?
To find the simple interest calculator (SI calculator), click here. This calculator allows us to enter the values of principal, rate of interest, and time duration (in years/months/days) and finds the simple interest showing step-by-step solution.
Are Car Loans Simple or Compound Interest?
Car loans or auto loans use simple interest to calculate the interest. The borrower agrees to pay the money back, plus a flat percentage of the amount borrowed. But in case the borrower fails to repay the amount on time, the company or the lender may start charging compound interest.
What is the Difference between Simple and Compound Interest?
Simple interest is the interest paid only on the principal, whereas, compound interest is the interest paid on both principal and interest compounded in regular intervals.
How to Calculate Simple Interest?
Simple Interest is calculated using the following formula: SI = P × R × T, where P = Principal, R = Rate of Interest, and T = Time period. Here, the rate is given in percentage (r%) is written as r/100. And the principal is the sum of money that remains constant for every year in the case of simple interest.
How do I Calculate Simple Interest Monthly?
To calculate simple interest monthly, we have to divide the yearly interest calculated by 12. So, the formula for calculating monthly simple interest becomes (P × R × T) / (100 × 12).
What is Simple Interest Rate Formula?
Using the simple interest formula, SI = PRT/100. To find the rate R from this, we just solve this equation for R. Then we get R = (SI × 100) / (P × T).
visual curriculum