Integration
Integration is a way of uniting the part to find a whole. In the integral calculus, we find a function whose differential is given. Thus integration is the inverse of differentiation. Integration is used to define and calculate the area of the region bounded by the graph of functions. The area of the curved shape is approximated by tracing the number of sides of the polygon inscribed in it. This process known as the method of exhaustion was later adopted as integration. We obtain two forms of integrals, indefinite and definite integrals. Differentiation and integration are the fundamental tools in calculus that are used to solve problems in math and physics. The principles of integration were formulated by Leibniz. Let's move further and learn about integration, its properties, and some of its powerful techniques.
| 1. | What is Integration? |
| 2. | Integration an Inverse Process of Differentiation |
| 3. | Rules of Integration |
| 4. | Methods of Integration |
| 5. | Integration of Rational Algebraic Functions |
| 6. | FAQs on Integration |
What is Integration?
Integration is the process of finding the area of the region under the curve. This is done by drawing as many small rectangles covering up the area and summing up their areas. The sum approaches a limit that is equal to the region under the curve of a function. Integration is the process of finding the antiderivative of a function. If a function is integrable and if its integral over the domain is finite, with the limits specified, then it is the definite integration.

If d/dx(F(x) = f(x), then ∫ f(x) dx = F(x) +C. These are indefinite integrals. For example, let f(x) = x3 be a function. The derivative of f(x) is f’(x) = 3x2 and the antiderivative of 3x2 is f(x) = x3
| Function F(x) | Derivative F'(x) = f(x) | Antiderivative of f(x) |
| x3 + 0 | 3x2 | x3 + ? |
| x3 + 2 | 3x2 | x3 + ? |
| x3 - 4 | 3x2 | x3 + ? |
Thus we find that the derivatives of F(x) = f(x), however, the anti-derivatives of f(x) are not unique. The anti-derivative of f(x) is a family of infinitely many functions. In fact, there exist infinite integrals of this function because the derivative of any real constant C is zero and we can write as ∫ cos x. dx = sin x + C. It is the rule of integration to add an arbitrary constant C from the set of real numbers. Thus we conclude that, If \(\dfrac{dy}{dx}=f(x)\), then we write \(y=\int f(x) dx\) which is read as "Integral of f with respect to x."
Theorem: If F(x) is a particular anti-derivative of f(x) on an interval I, then every anti-derivative of f(x) on I is given by ∫ f(x) dx = F(x) + C.
- Here, ∫ f(x) dx represents the whole class of integral.
- C is the arbitrary constant, and all the antiderivatives of f(x) on I can be obtained by assigning a particular value to C.
- Here f(x) is the integrand,
- The variable x in dx is called the integrator and the whole process of finding the integral is called the integration. The ∫ sign stands for the sum.

Integration an Inverse Process of Differentiation
We are given a derivative of a function and are asked to find its primitive, that is, the original function. Such a process is called anti-differentiation or integration. If we are given the derivative of a function, the process of finding the original function is called integration. The derivatives and the integrals are opposite to each other. Consider a function f(x)= sin x. The derivative of f(x) is f'(x) = cos x. We say that the function cos x is the derived function of sin x. Similarly, we say that sin x is the anti-derivative of cos x.
Rules of Integration
We already know the formulas of derivatives of some important functions. Here are derivatives and their corresponding standard integrals of a few functions given as integration formulas.
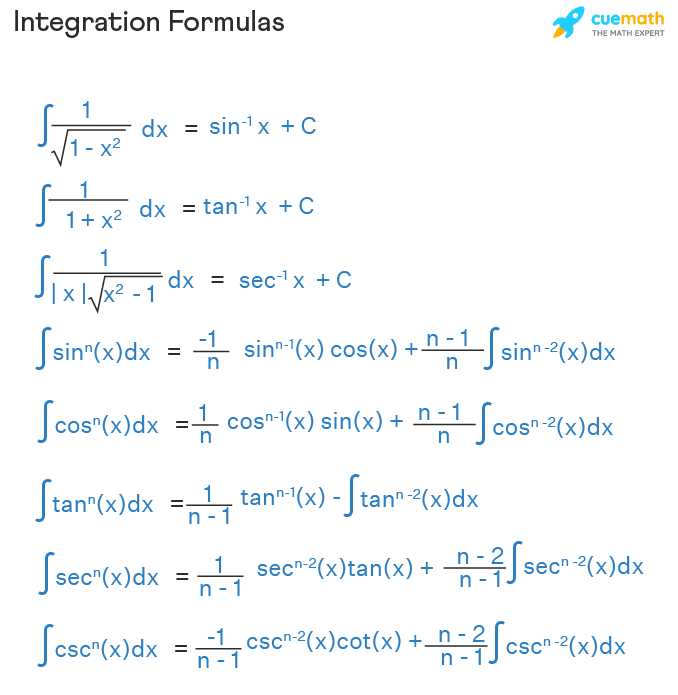
There are certain rules defined for finding integrals. They include:
Sum and Difference Rules:
- ∫ [f(x)+g(x)] dx = ∫ f(x) dx + ∫ g(x) dx
- ∫ [f(x)-g(x)] dx =∫ f(x) dx - ∫ g(x) dx
Power Rule: ∫ xn dx = (xn+1)/ (n+1)+ C. (Where n ≠ -1)
Exponential Rules:
- ∫ ex dx = ex + C
- ∫ ax dx = ax /ln(a) + C
- ∫ ln(x) dx = x ln(x) -x + C
Constant Multiplication Rule:
- ∫ a dx = ax + C, where a is the constant.
Reciprocal Rule:
- ∫ (1/x) dx = ln(x)+ C
Properties of Integration
A few properties of indefinite integrals are:
- ∫ [f(x)±g(x)] dx =∫ f(x) dx ± ∫ g(x) dx
- ∫ k f(x) dx = k ∫ f(x) dx, where k is any real number.
- ∫ f(x) dx = ∫ g(x) dx, if ∫ [f(x)-g(x)] dx = 0
- The combination of first two properties arise to \(\int [k_1 f_1(x) dx + k_2 f_2 (x) dx + .........k_n f(x)dx]\\\\= k_1\int f_1(x) dx + k_2 \int f_2 (x) dx + .... +k_n \int f(x) dx\)
Methods of Integration
Sometimes, the inspection is not enough to find the integral of some functions. There are additional methods to reduce the function in the standard form to find its integral. Prominent methods are discussed below.
The methods of integration are:
- Decomposition method
- Integration by Substitution
- Integration using Partial Fractions
- Integration by Parts
Method 1: Integration by Decomposition
The functions can be decomposed into a sum or difference of functions, whose individual integrals are known. The given integrand will be algebraic, trigonometric or exponential or a combination of these functions.
Suppose we need to integrate (x2 -x +1)/x3 dx, we decompose the function as :
∫ (x2 -x +1)/x3 dx = ∫ (x2 /x3 - x /x3 +1/x3 )
= ∫ (1/x)dx - ∫ (1/x2) dx + ∫ (1/x3)dx
Applying the reciprocal rule and the power rule, we get
∫ (x2 -x +1)/x3 dx = log|x| + 1/x - 1/2x2 + C
Method 2: Integration by Substitution
The integration by substitution method lets us change the variable of integration so that the integrand is integrated in an easy manner.
Suppose, we have to find y =∫ f(x) dx.
Let x=g(t). Then, \(\dfrac{dx}{dt}=g'(t)\).
So, y= ∫ f(x) dx can be written as y= ∫ f(g(t)) g'(t).
For example, let's find the integral of f(x) = sin(mx) using substitution.
Let mx = t. Then, \(m\dfrac{dx}{dt}=1\).
\(\begin{align}y&=\int \sin{mx}dx\\&=\dfrac{1}{m}\int \sin{t}dt\\&=-\dfrac{1}{m} \cos{t}+C\\&=-\dfrac{1}{m} \cos{mx}+C\end{align}\)
y=∫ sin(mx)dx can be written as ∫ f(g(t)) g'(t)dt
Note: The substitution for the variable of integration can also use trignonometric identities. A few important standard results are:
- ∫ tan x dx = log|secx| +C
- ∫ cot x dx = log|sin x| +C
- ∫cosec x dx = log|cosec x -cot x| +C
- ∫ sec x dx = log|secx + tan x| +C
Method 3: Integration using Partial Fractions
Suppose we have to find \(y=\int \dfrac{P(x)}{Q(x)} dx\) where \(\dfrac{P(x)}{Q(x)}\) is an improper rational function. We reduce it in such a way that \(\dfrac{P(x)}{Q(x)}=T(x)+\dfrac{P_{1}(x)}{Q(x)}\). Here, T(x) is polynomial in x and \(\dfrac{P_{1}(x)}{Q(x)}\) is proper rational function. The following table shows some rational functions and their corresponding form of partial fractions.

For example, let's find the integral of \(f(x)=\dfrac{1}{(x+1)(x+2)}\) using integration by partial fractions.
By using partial fraction we have \(\dfrac{1}{(x+1)(x+2)}=\dfrac{A}{x+1}+\dfrac{B}{x+2} \cdots (1)\).
We will determine the values of A and B.
On comparing in equation (1), we get 1=A(x+2)+B(x+1).
From this, we have a set of two linear equations.
A+B=0 and 2A+B =1
On solving these equations we get, A=1 and B=-1.
So, equation (1) can be written as \(\dfrac{1}{(x+1)(x+2)}=\dfrac{1}{x+1}-\dfrac{1}{x+2}\).
Now, solving the integral
\(\begin{align}\int \left(\dfrac{1}{(x+1)(x+2)}\right)dx\\=\int \left(\dfrac{1}{x+1}-\dfrac{1}{x+2}\right)dx\\=\log{|x+1|}-\log{|x+2|}+C\\=\log{\left|\dfrac{x+1}{x+2}\right|}+C\end{align}\)
Method 4: Integration by Parts
This Integration rule is used to find the integral of two functions.
By product rule of derivatives, we have \(\dfrac{d}{dx}(uv)=u\dfrac{dv}{dx}+v\dfrac{du}{dx}\;\;\;\;\;\;\; \cdots (1)\)
Integration on both sides of equation (1), we get \(\int u\dfrac{dv}{dx}dx=uv-\int v\dfrac{du}{dx} dx\;\;\;\;\;\;\; \cdots (2)\)
Equation (2) can be written as \(uv=\int u\dfrac{dv}{dx}dx+\int v\dfrac{du}{dx} dx\)
Let u=f(x) and \(\dfrac{dv}{dx}=g(x)\).
Then, we have \(\dfrac{du}{dx}=f'(x)\) and v = ∫ g(x)dx.
So, Equation (2) becomes
\(\begin{align}\int f(x) g(x)dx\\=f(x) \int g(x)dx-\int [f'(x) \int g(x)dx]dx\end{align}\)
For example, let's find the integral of \(xe^{x}\) using integration by parts.
\(\begin{align}\int xe^{x}dx&=x \int e^{x}dx-\int \left(\dfrac{dx}{dx}\int e^{x}dx\right]dx\\&=x e^{x}-\int [e^{x})dx\\&=x e^{x}-e^{x}+C\end{align}\)
A few important standard results(Bernoulli's formula):
- ∫ eax sin bx dx = eax /(a2 + b2)[a sin bx - b cos bx] + C
- ∫ eax cos bx dx = eax /(a2 + b2)[a cos bx + b sin bx] + C
Integration of Rational Algebraic Functions
To integrate the rational algebraic functions whose numerator and denominator contain some positive integral powers of x with the constant coefficients, we use integration by partial fractions and arrive at a few standard results that could be directly applied as integration formulas.
- ∫1/ (a2 - x2) dx = (1/2a) log|(a+x)/(a-x)| +C
- ∫1/ (x2 - a2) dx = (1/2a) log|(x-a)/(x+a)| +C
- ∫1/ √(x2 - a2) dx = log |x + √(x2 - a2)|+C
- ∫ 1/ √(x2 + a2) dx = log |x + √(x2 + a2)|+C
- ∫ 1/ √(a2 - x2) dx = sin-1 (x/a) +C
- ∫1/ (a2 + x2) dx = (1/a) tan -1 (x/a) + C
Important notes
- Integration is an inverse process of differentiation.
- Always add the constant of integration after determining the integral of the function.
- If two functions, say f(x) and g(x) have same derivatives, then |f(x)-g(x)|= C, where C is some constant.
☛ Also Check:
Examples of Integration
-
Example 1: Integrate the function f(x)=2x sin(x2+1) with respect to x.
Solution:
Observe that the derivative of x2+1 is 2x.
So, we will proceed with integration by substitution.
Let x2+1=z
Then, 2x dx = dz
\(\begin{align}\int f(x) dx&=\int 2x \sin{(x^2+1)}dx\\&=\int \sin{z}dz\\&=-\cos{z}+C\\&=-\cos{(x^{2}+1)}+C\end{align}\)
∴\(\int 2x \sin{(x^2+1)}dx=-\cos{(x^{2}+1)}+C\)
-
Example 2: Find the integral \(\int \dfrac{x^2+1}{x^2-5x+6}dx\).
Solution:
Observe that the function \(\dfrac{x^2+1}{x^2-5x+6}\) is an improper rational function.
Thus we apply integration using partial fractions.On dividing this function, we get 5x-5 as the remainder.
So, by long division, this can be written as \(\dfrac{x^2+1}{x^2-5x+6}=1+\dfrac{5x-5}{x^2-5x+6}dx\).
By factorization, we have \(x^2-5x+6=(x-2)(x-3)\).
So, \(1+\dfrac{5x-5}{x^2-5x+6}=1+\dfrac{5x-5}{(x-2)(x-3)}\)
Let's proceed with partial fraction on \(\dfrac{5x-5}{(x-2)(x-3)}\).
\(\begin{align}\dfrac{5x-5}{(x-2)(x-3)}=\dfrac{A}{x-2}+\dfrac{B}{x-3}\end{align}\)
On comparing, we get, \(5x-5=A(x-3)+B(x-2)\).
From this, we have a set of two linear equations.
A+B =5 --------->(1)
3A+2B =5 --------->(2)
On solving these equations (1) and (2) we get, A= -5 and B =10
\(1+\dfrac{5x-5}{x^2-5x+6}=1-\dfrac{5}{x-2}+\dfrac{10}{x-3}\).
Integrate on both sides of equation,
\(\begin{align}I&=\int \left(1+\dfrac{5x-5}{x^2-5x+6}\right)dx\\&=\int \left(1-\dfrac{5}{x-2}+\dfrac{10}{x-3}\right)dx\\&=\int dx-5\int \dfrac{dx}{x-2}+10\int \dfrac{dx}{x-3}\\&=x-5\log{|x-2|}+10\log{|x-3|}+C\end{align}\)
Answer: ∴ \(x-5\log{|x-2|}+10\log{|x-3|}+C\)
-
Example 3: If \(\dfrac{d}{dx}f(x)=4x^3-\dfrac{3}{x^4}\) and \(f(2)=0\), find the function \(f(x)\).
Integration on both sides of the equation \(\dfrac{d}{dx}f(x)=4x^3-\dfrac{3}{x^4}\).
\(\begin{align}\int \dfrac{d}{dx}f(x) dx&=\int \left(4x^3-\dfrac{3}{x^4}\right)dx\\f(x)&=4\left(\dfrac{x^4}{4}\right)-\dfrac{3x^{-3}}{-3}+C\\f(x)&=x^4+\dfrac{1}{x^{3}}+C\end{align}\)
Use the condition f(2)=0 after integration, to find the value of C.
\(\begin{align}f(2)&=0\\2^4+\dfrac{1}{2^{3}}+C&=0\\16+\dfrac{1}{8}+C&=0\\C&=-\dfrac{129}{8}\end{align}\)
Answer: ∴ the function is \(f(x)=x^4+\dfrac{1}{x^{3}}-\dfrac{129}{8}\).
-
Example 4: Calculate ∫ cos2 x dx
Using the trigonometric identity, we have:
\(\int \cos^{2}{x}dx=\int\left(\dfrac{1+\cos{(2x)}}{2}\right)\).
On integration, we get\(\begin{align}\int \cos^{2}{x}dx&=\int\left(\dfrac{1+\cos{(2x)}}{2}\right)dx\\&=\dfrac{1}{2}\int dx+\dfrac{1}{2}\int \cos{(2x)}dx\\&=\dfrac{x}{2}+\dfrac{1}{4}\sin{(2x)}+C\end{align}\)
Answer: ∴ ∫ cos2 x dx = \dfrac{x}{2}+\dfrac{1}{4}\sin{(2x)}+C\)
go to slidego to slidego to slidego to slide

FAQs on Integration
What is Integration?
The process of finding the antiderivatives of functions also known as integrals is called integration. It is the technique of finding a function, g(x), the derivative of which d/dx(g(x)), is equal to the function f(x). It is represented as ∫ f(x) and it is called the indefinite integral of the function.∫ f(x)dx is the summation of the product of the function and its displacement along x.
What is the Use of Integration?
The definite integrals in integration are used to find the quantities like area, volume, etc., that can be interpreted as the area below the curve. The antiderivatives are found to help in calculating the definite integrals.
What is The Integration of 1?
The integration of 1 is (x+C). The integration of a constant, ie., ∫ a. dx = ax + C, where a is the constant. Here ∫1. dx = x + C
What are The Methods of Integration of a Function?
There are many methods to integrate a function. A few standard integrals are just finding the antiderivatives, for which the basic integration formulas are used. There are a few methods to be followed like substitution method, integration by parts, and integration using partial fractions. These are discussed here in this article.
What Are The Rules of Integration?
There are many rules of integration that help us find the integrals. the power rule, the sum and difference rules, the exponential rule, the reciprocal rule, the constant rule, the substitution rule, and the rule of integration by parts are the prominent ones.
What is The Integration of √x?
As per the power rule of integration, we know ∫ xn dx = (xn+1)/ (n+1)+ C.
∴ ∫ x½ . dx = (x½+1)/ (½+1)+ C.
= (x3/2)/ (3/2)+ C = (2/3) x3/2
How Integration is Used in Real Life?
The applications of integration in real life are mentioned below.
- In electrical engineering, we need a cable to connect two substations that are miles apart from each other. Integration helps us to find the exact length of the cable.
- In physics, integration is used to find the center of mass, the center of gravity, the velocity of an object, etc.
- In epidemiology, integral calculus is used to study the spread of an infectious disease.
Why is integration important?
Calculus is centered on the concepts of derivatives and integration. In mathematics, we use integration to find the areas, volumes, displacement, etc. In fact, the concept of integration in calculus gave birth to integral calculus.
visual curriculum