Area of Parallelogram
The area of a parallelogram is defined as the region or space covered by a parallelogram in a two-dimensional plane. It is calculated by multiplying the base of the parallelogram by its height. i.e, area, A = base × height.
A parallelogram is a special kind of quadrilateral. If a quadrilateral has two pairs of parallel opposite sides, then it is called a parallelogram. Finding the area of parallelograms has very important applications in real life such as agriculture, construction, physics, etc. Let us learn how to find the area of a parallelogram.
| 1. | What is the Area of Parallelogram? |
| 2. | Area of Parallelogram Formula |
| 3. | How to Calculate the Area of Parallelogram? |
| 4. | Area of Parallelogram in Vector Form |
| 5. | FAQs on Area of Parallelogram |
What is the Area of Parallelogram?
The area of a parallelogram refers to the total number of unit squares that can fit into it and it is measured in square units (like cm2, m2, in2, etc). It is the region enclosed or encompassed by a parallelogram in two-dimensional space. Let us recall the definition of a parallelogram. A parallelogram is a four-sided, 2-dimensional figure with two pairs of parallel opposite sides.
We come across many geometric shapes other than rectangles and squares in our daily lives. Since few properties of a rectangle and a parallelogram are somewhat similar, the area of the rectangle is similar to the area of a parallelogram.
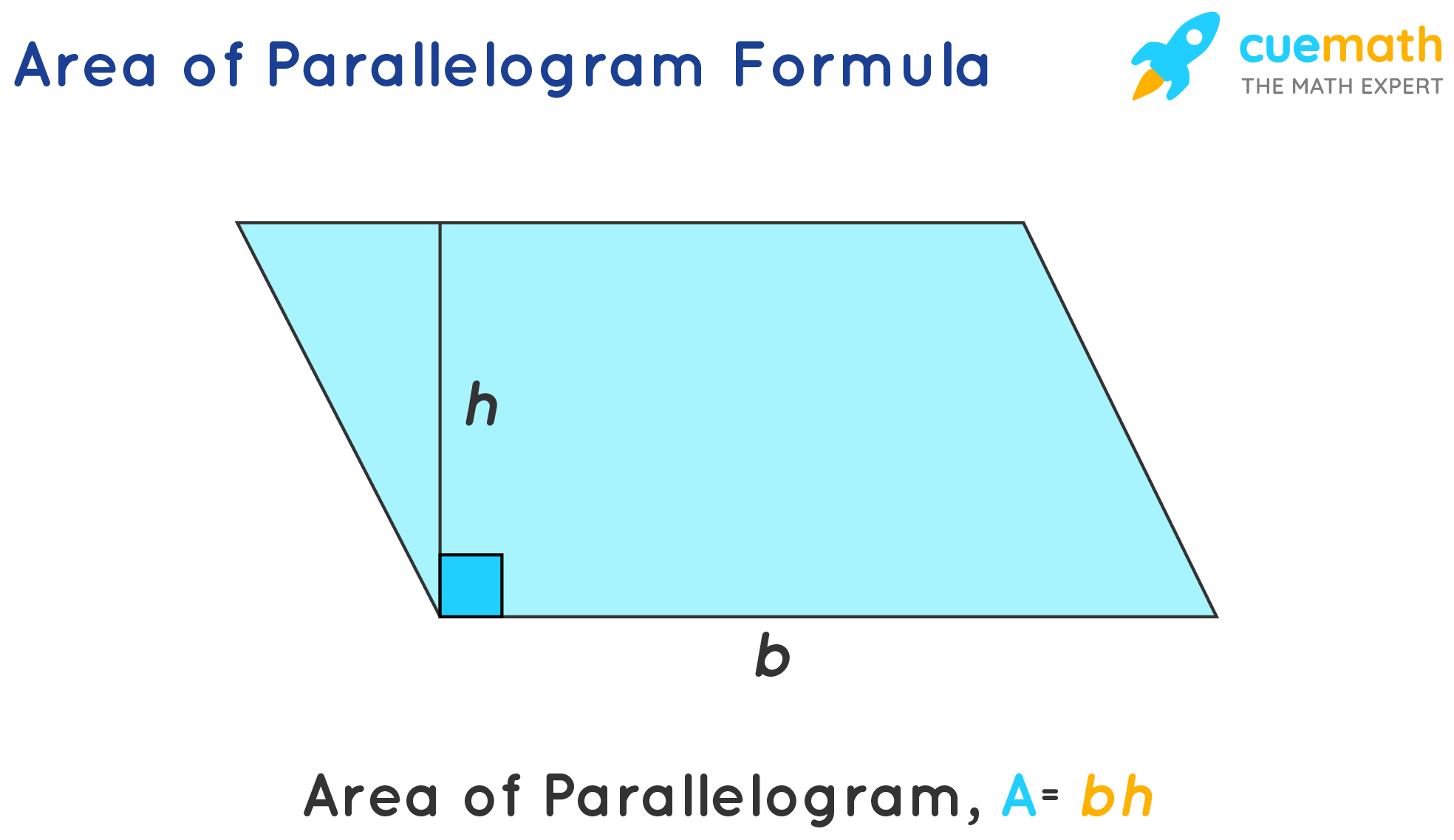
Area of a Parallelogram Formula
The area of a parallelogram can be calculated by multiplying its base by the altitude. The base and altitude of a parallelogram are perpendicular to each other as shown in the following figure. The formula to calculate the area of a parallelogram can thus be given as,
Area of parallelogram = b × h square units
where,
- b is the length of the base
- h is the height or altitude
Let us analyze the above formula using an example. Assume that PQRS is a parallelogram. Using grid paper, let us find its area by counting the squares.

Now, we will calculate the area of parallelogram by two methods: (i) By counting squares (ii) By using formula
Area of Parallelogram by Counting Squares:
From the above figure:
total number of complete squares = 16
total number of half squares = 8
Area of the parallelogram = 16 + (1/2) × 8 = 16 + 4 = 20 unit2
Area of Parallelogram by Using Formula:
Also, we observe in the figure that ST ⊥ PQ. By counting the squares, we get:
Side, PQ = 5 units
Corresponding height, ST = 4 units
Area of the parallelogram = Side × height = 5 × 4 = 20 unit2
Note that we got the same answer using both methods. Thus, the area of the given parallelogram is base times the altitude.
Let's do an activity to understand the area of a parallelogram.
- Step I: Draw a parallelogram (PQRS) with altitude (SE) on a cardboard and cut it.
- Step II: Cut the triangular portion (PSE).
- Step III: Paste the remaining portion (EQRS) on a white chart.
- Step IV: Paste the triangular portion (PSE) on the white chart joining sides RQ and SP.

After doing this activity, we observed that the area of a rectangle is equal to the area of a parallelogram. Also, the base and height of the parallelogram are equal to the length and breadth of the rectangle respectively.
Area of Parallelogram = Base × Height (which is nothing but length × width of the rectangle).
How to Calculate the Area of a Parallelogram?
The parallelogram area can be calculated in each of the following cases:
- when its base and height are known.
- if the length of the adjacent sides along with the angle between them is known.
- if its two diagonals along with any of their intersecting angles are known
Parallelogram Area Using Height
Suppose 'a' and 'b' are the set of parallel sides of a parallelogram and 'h' is the height (which is the perpendicular distance between 'a' and 'b'), then the area of a parallelogram is given by:
Area = Base × Height
A = b × h [square units]
Example: If the base of a parallelogram is equal to 10 cm and the height is 8 cm, then find its area.
Solution: Given, length of base = 10 cm and height = 8 cm
As per the formula, Area = 10 × 8 = 80 cm2
Parallelogram Area Using Lengths of Sides
The area of a parallelogram can also be calculated without the height if the length of adjacent sides and the angle between them are known to us. We can simply use the area of the triangle formula from the trigonometry concept for this case.
Area = ab sin (θ)
where,
- a and b = length of adjacent sides, and,
- θ = angle between a and b
Example: The angle between any two sides of a parallelogram is 90 degrees. If the length of the two adjacent sides is 4 units and 6 units respectively, then find the area.
Solution:
Let a = 4 units and b = 6 units
θ = 90 degrees
Using area of parallelogram formula,
Area = ab sin (θ)
⇒ A = 4 × 6 sin (90º)
⇒ A = 24 sin 90º
⇒ A = 24 × 1 = 24 sq.units.
Note: If the angle between the sides of a parallelogram is 90 degrees, then the parallelogram becomes a rectangle.
Parallelogram Area Using Diagonals
The area of any given parallelogram can also be calculated using the length of its diagonals. There are two diagonals for a parallelogram, intersecting each other at certain angles. Suppose, this angle is given by x, then the area of the parallelogram is given by:
Area = ½ × d1 × d2 sin (x)
where,
- d1 and d2 = Length of diagonals of the parallelogram, and
- x = Angle between the diagonals.
Area of Parallelogram in Vector Form
The area of the parallelogram can be calculated using different formulas even when either the sides or the diagonals are given in vector form.
- The area of a parallelogram whose adjacent sides are the vectors a and b is |a × b|.
- The area of a parallelogram whose diagonals are given by the vectors d1 and d2 is 1/2 |(d1 × d2)|.
In these two formulas, "×" stands for the "cross product of vectors". Let us now see how these two formulas are equivalent.
Consider a parallelogram ABCD as shown in the figure below,

Area of parallelogram in vector form using the adjacent sides is, |a × b|, where, a and b are vectors representing two adjacent sides.
By the triangle law of vectors,
a + b = d1 → i) and,
b + (-a) = d2
or, b - a = d2 → ii)
⇒ d1 × d2 = (a + b) (b - a)
= a × (b - a) + b × (b - a)
= a × b - a × a + b × b - b × a
Since a × a = 0, and b × b = 0
⇒ a × b - 0 + 0 - b × a
Since a × b = - b × a,
d1 × d2 = a × b - (-(a × b))
d1 × d2 = 2(a × b)
1/2 (d1 × d2) = a × b
Therefore, the area of the parallelogram when diagonals are given in vector form = 1/2 |(d1 × d2)|, where, d1 and d2 are diagonals.
Thinking Out Of the Box!
- Can a kite be called a parallelogram?
- What elements of a trapezoid should be changed to make it a parallelogram?
- Can there be a concave parallelogram?
- Can you find the area of the parallelogram without knowing its height?
☛ Related Topics:
Area of Parallelogram Examples
-
Example 1: The adjacent sides of a parallelogram are 10 in and 6 in. The altitude corresponding to side 10 in is 5 in. Find the area and the length of the altitude corresponding to the side 6 in.

Solution:
Let ABCD be a parallelogram where DE ⊥ AB, AF ⊥ BC
Using the area of parallelogram formula,
Area of Parallelogram ABCD = (10) × (5) = 50 in2
Length of BC = 6 in
Length of Altitude AF = (50 ÷ 6) = 8.3 in
Answer: Area of the given parallelogram = 50 in2; Altitude = 8.3 in
-
Example 2: Calculate the area of a solar sheet that is in the shape of a parallelogram, given that, the base measures 20 in, and the altitude measures 8 in.
Solution:
Using the area of parallelogram formula,
Area of the solar sheet = B × H = (20) × (8) = 160 in2
Answer: Area of solar sheet = 160 in2
-
Example 3: The area of a playground which is in the shape of a parallelogram is 2500 in2, with one side measuring 250 in. Find the corresponding altitude.
Solution:
Area of the playground = 2500 in2
Side of a playground = 250 in
By using the area of the parallelogram formula,
Area = Side × Altitude
2500 = 250 × Altitude
Altitude = 2500/250 = 10 in
Answer: The corresponding altitude of the playground measures 10 in.

FAQs on Area of Parallelogram
What is the Area of a Parallelogram in Math?
The area of a parallelogram is defined as the region enclosed by it in two-dimensional space. It is measured in square units like cm2, m2, in2, etc. The area of a parallelogram is calculated by the formula, A = b × h where:
- A = area of parallelogram
- b = base
- h = height
What is the Area of a Parallelogram Formula?
The area of a parallelogram can be calculated by finding the product of its base with the altitude. The base and altitude of a parallelogram are always perpendicular to each other. The formula to calculate the area of a parallelogram is given as Area of parallelogram = (base × height square units.
How to Find Area of a Parallelogram Without Height?
The area of a parallelogram can be calculated without the height when the length of adjacent sides and the angle between them is known. The formula to find the area for this case is given as, area = ab sin (θ), where 'a' and 'b' are lengths of adjacent sides, and, θ is the angle between them.
Also, the area can be calculated when the diagonals and their intersecting angle are given, using the formula, Area = ½ × d1 × d2 sin (y), where 'd1' and 'd2' are lengths of diagonals of the parallelogram, and 'y' is the angle between them.
How to Find Area of Parallelogram With Vectors?
The area of a parallelogram can be calculated when the adjacent sides or diagonals are given in vector form. The formula to find area using vector adjacent sides is given as, |a × b|, where a and b are adjacent side vectors. Also, the area of parallelogram formula using diagonals in vector form is, area = 1/2 |(d1 × d2)|, where d1 and d2 are diagonal vectors.
How to Calculate the Area of Parallelogram Whose Adjacent Sides are Given?
To find the area of a parallelogram when the lengths of adjacent sides are given, we need the angle between them. The formula to find the area for this case is given as, area = ab sin (θ), where 'a' and 'b' are lengths of adjacent sides, and, θ is the angle between the adjacent sides of the parallelogram.
How to Calculate Area of Parallelogram Using Calculator?
To determine the area of a parallelogram the easiest and fastest method is to use the area of a parallelogram calculator. It is a free online tool that helps you to calculate the area of a parallelogram with the help of the given dimensions. Try now Cuemath's area of parallelogram calculator, enter the value of height and base of the parallelogram and get the parallelogram's area within a few seconds.
What is the Area of a Parallelogram When Diagonals are Given?
The area of a parallelogram can be calculated when the diagonals and their intersecting angle are known. The formula is given as, area = ½ × d1 × d2 sin (x), where 'd1' and 'd2' are lengths of diagonals of the parallelogram, and 'x' is the angle between them.
visual curriculum