Trapezoid
The trapezoid is fascinating because it is defined based on the geography you belong to. If you’re visiting the United Kingdom on an exchange trip and ask a student to draw a trapezoid for you, then they will draw it like a trapezium. A trapezoid is also called a trapezium in some parts of the world and it is a type of quadrilateral with one pair of opposite sides parallel to each other.
| 1. | What is Trapezoid? |
| 2. | Properties of Trapezoid |
| 3. | Types of Trapezoids |
| 4. | Trapezoid Formula |
| 5. | FAQs on Trapezoid |
What is Trapezoid?
A trapezoid is also known as a trapezium is a four-sided polygon or a quadrilateral. It has one set of opposite sides which are parallel and a set of non-parallel sides. The parallel sides are known as the bases and the non-parallel sides are known as the legs of the trapezoid.
Definition of Trapezoid
A trapezoid is a four-sided closed 2D figure which has an area and its perimeter. Two sides of the shape are parallel to each other and they are termed as the bases of the trapezoid. The non-parallel sides are known as the legs or lateral sides of a trapezoid. The shortest distance between two parallel sides is known as the altitude. Since the opposite sides are parallel to each other, calculating the area of a trapezoid is simple.
Properties of Trapezoid
These are the properties of a trapezoid that make it stand out from other quadrilaterals:
- The bases (the top and bottom) are parallel to each other
- Opposite sides of a trapezoid (isosceles) are of the same length
- Angles next to each other sum up to 180°
- The median is parallel to both the bases
- Median's length is the average of both the bases i.e. (a +b)/2
- If both pairs of the opposite sides are parallel in a trapezoid, it is considered a parallelogram
- If both pairs of the opposite sides are parallel, all sides are of equal length, and at right angles to each other, then a trapezoid can be considered as a square
- If both pairs of the opposite sides are parallel, its opposite sides are of equal length and at right angles to each other, then a trapezoid can be considered as a rectangle
Types of Trapezoids
There are three types of trapezoids, and those are given below:
- Isosceles Trapezoid
- Scalene Trapezoid
- Right Trapezoid
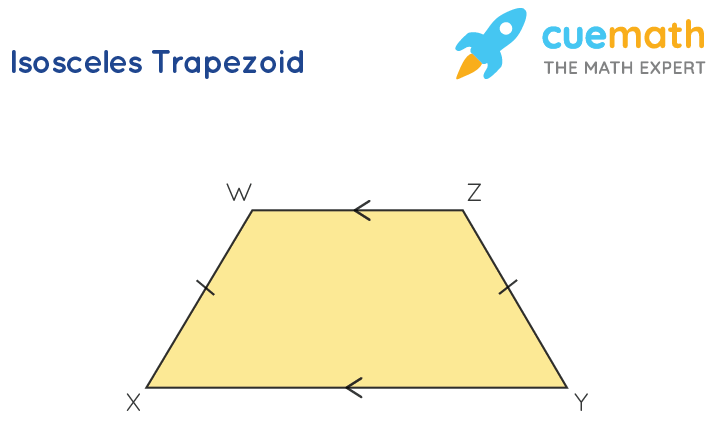
Isosceles Trapezoid
If the legs or non-parallel sides of the trapezoid are equal in length, then it is called an isosceles trapezoid. The angles of the parallel sides (base) in the isosceles trapezoid are equal to each other. An isosceles trapezoid has a line of symmetry and both the diagonals are equal in length.
In the below isosceles trapezoid XYZW, XY and WZ are called the bases of the trapezoid. WX and YZ are called the legs of the trapezoid since they are not parallel to each other.
Scalene Trapezoid
When neither the sides nor the angles of the trapezoid are equal, then it is a scalene trapezoid. In the below scalene trapezoid, all four sides i.e. AB, BC, CD, and DA are of different lengths. The bases i.e. DC and AB are parallel to each other but are of different lengths.
Right Trapezoid
A right trapezoid also called the right-angled trapezoid, has a pair of right angles. These kinds of trapezoids are used to estimate the areas under the curve. In the below right trapezoid or right-angled trapezoid, there are two right angles one at D and the other one at A. One pair of opposite sides i.e. DC and AB are parallel to each other.
Trapezoid Formula
There are two main trapezoid formulas, they are:
- Area of a Trapezoid
- Perimeter of a Trapezoid
Area of Trapezoid
The area of the trapezoid is calculated by measuring the average of the parallel sides and multiplying it with its height. To find the area of a trapezoid, the lengths of two of its parallel sides is to be known and the distance (height) between them. It is the number of unit squares that can be fit inside the shape and it is measured in square units such as cm2, m2, in2, etc. The formula of the area (A) of a trapezoid is calculated on the bases i.e. a and b and whose height is h which is the perpendicular distance between a and b.
Hence, the area of a trapezoid is calculated by the following formula:
Area =[(AB + CD)/2] × h
A = [(a + b)/2] × h
Where,
- AB and CD = parallel sides
- a = shorter base
- b = longer base
- h = height or altitude
Perimeter of Trapezoid
The perimeter of a trapezoid is defined as the total length of the boundary of the shape, i.e. the sum of all its sides. Since the trapezoid is a two-dimensional shape, thus, the perimeter will also lie in a two-dimensional plane only. Consider a trapezoid ABCD as shown below with side measures a,b,c, and d. Let's look into the Trapezoid Formula. The perimeter of the trapezoid formula is calculated by finding the sum of all the sides i.e, AB + BC + CD + DA
Perimeter of a Trapezoid = Sum of all the sides = a + b + c + d
where, a, b,c, and d are the sides of the trapezoid.
☛Related Topics on Trapezoid
Listed below are a few topics that are related to a trapezoid.
Trapezoid Examples
-
Example 1: If the area of the trapezoid is 128 inches and the lengths of the bases are 12 inches and 20 inches, what will be the height of the trapezoid?
Solution:
Let us assume that the bases are a and b, and the height of the trapezoid is h. Using the given information,
We have to find h which is the distance or height between the bases. Let us substitute all these values in the area of a trapezoid formula:
A = [(a + b)/2] × h
128 = [(20 + 12)/2] × h
256 = 32 × h
h = 8 inches
Therefore, the height of the trapezoid is 8 inches.
-
Example 2: Sarah has been asked to find the area of a trapezoid with sides 13 units, 8 units, 17 units, and 8 units. Can you help her?
Solution:
Let us assume that a and b are the bases, and h is the height of the given trapezoid.
The above trapezoid can be represented as follows:
13 + x + x = 17
13 + 2x =17
2x = 4
Therefore x =2
Now, applying Pythagoras' Theorem to one of the triangles,
82 = 22 + h2
64 = 4 + h2
h2 = 60
h=√60
h=√4×15
h=2√15
Finally, we will use the area of a trapezoid formula to find its area:
A = [(a + b)/2] × h
A = [(13 + 17)/2] × 2√15
A = (60√15)/2
A=30√15 sq units
Therefore, the area of the given trapezoid is 30√15 sq units
FAQs on Trapezoid
What is a Trapezoid?
A trapezoid is a four-sided closed 2D shape that has an area and a perimeter. It is also called a Trapezium. The sides of a trapezoid are parallel to each other and they are termed as the bases of the trapezoid. The non-parallel sides are known as the legs or lateral sides of a trapezoid. The shortest distance between two parallel sides is known as the altitude.
What are the Types of Trapezoid?
The trapezoids are classified on the basis of the nature of their sides. The main types of trapezoids are listed below:
- Isosceles Trapezoid
- Scalene Trapezoid
- Right Trapezoid
What are the Basic Properties of Trapezoid?
There are many properties of a trapezoid few of them are listed below:
- The top and the bottom bases of a trapezoid are parallel.
- An isosceles trapezoid consists of equal lengths of opposite sides.
- Angles next to each other sum up to 180°.
How to Find the Area of a Trapezoid?
The area of a trapezoid is calculated by calculating the average of the two parallel sides and multiplying it by its height.
Area = [(a + b)/2] × h, where a and b are the lengths of the bases and h represents the height.
What is the Equation for a Trapezoid?
There are two equations for a trapezoid. One equation calculates its area; the other is its perimeter. The perimeter of a trapezoid PQRS is given as, Perimeter = PQ + QR + RS + PS. The area of a trapezoid = [(a + b)/2] x h, where a and b are the lengths of the bases and h represents the height.
Is Trapezoid a Quadrilateral?
Since the trapezoid has four sides, it automatically becomes a quadrilateral. It has two sides that are parallel, and two sides that aren’t.
What are the Three Attributes of a Trapezoid?
The three main attributes of a trapezoid are as follows
- Its base angles and diagonals are equal if the trapezoid is isosceles
- The intersection point of the diagonals is collinear (in the same line) to the midpoints of the two opposite sides
- Opposite sides of an isosceles trapezoid are congruent
How do you Find the Missing Side of a Trapezoid?
The missing side of the trapezoid can be determined from the information given to you. In case, you have the area, and the length of the bases, you can find the length of the height. You can also determine the length of the missing side if you know the perimeter and the length of the other three sides of the trapezoid.
Are the Diagonals of a Trapezoid Equal?
Trapezoids are of three kinds - Isosceles, Scalene, and Right. In the case of the isosceles trapezoid, the diagonals are equal since the non-parallel or the legs of the trapezoid are equal in length. In the case of scalene and right trapezoid, diagonals are not equal.
How To Calculate Height of Trapezoid Using Trapezoid Formula?
Area of trapezoid formula, A = [(a + b)/2] × h
To calculate the height of the trapezoid we can mold the area of the trapezoid formula as
h = 2A/(a+b). Where 'a' is the shorter base, 'b' is the longer base, and 'h' is the distance between the two bases, and A is the area of a trapezoid.
What Are the Two Basic Trapezoids Formulas?
The two basic trapezoid formulas are:
The perimeter of a Trapezoid is Sum of all the sides. Expressed as P = a + b + c + d. Where, a, b,c, and d are the sides of the trapezoid.
Area of trapezoid formula, A = [(a + b)/2] × h.