Remainder Theorem
The remainder theorem is a fundamental concept in algebra and is used to find the remainder when a polynomial is divided by a linear expression. We know that the remainder by dividing one polynomial by other can be found by long division of polynomials. Instead, we can use the remainder theorem to find the remainder easily. This has many important applications:
- It is used to find the remainder when a polynomial is divided by another linear polynomial.
- It helps in the factorization of polynomials.
- It helps in determining the zeros of a polynomial.
But the remainder theorem has some limitations. This theorem works only when the divisor is linear. Let us go into depth about the remainder theorem by working on some examples.
What is the Remainder Theorem?
The remainder theorem states that when a polynomial p(x) is divided by a linear polynomial (x - a), then the remainder is equal to p(a). The remainder theorem enables us to calculate the remainder of the division of any polynomial by a linear polynomial, without actually carrying out the steps of the long division.
Note that the degree of the remainder polynomial is always 1 less than the degree of the divisor polynomial. Using this fact, when any polynomial is divided by a linear polynomial (whose degree is 1), the remainder must be a constant (whose degree is 0).
Remainder Theorem Statement and Proof
According to the remainder theorem, when a polynomial p(x) (whose degree is greater than or equal to 1) is divided by a linear polynomial x - a, the remainder is given by r = p(a). i.e., to find the remainder, follow the steps below:
- Find the zero of the linear polynomial by setting it to zero. i.e., x - a = 0 ⇒ x = a.
- Then just substitute it in the given polynomial. The result would give the remainder.
Here is the remainder theorem formula depending on the type of divisor (linear polynomial).
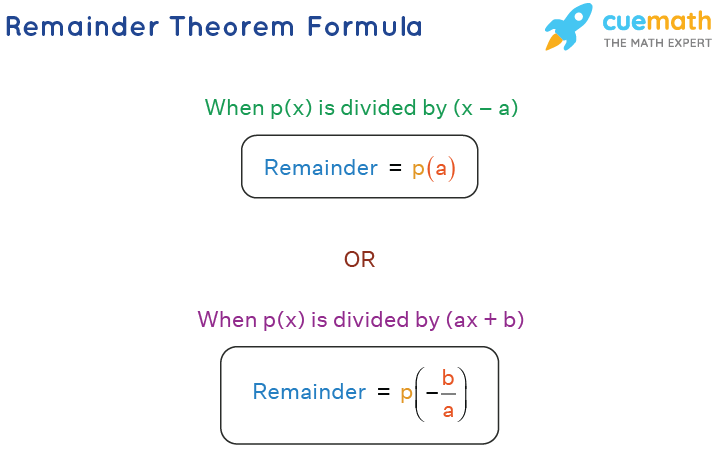
Similarly, we can extend the remainder theorem for different types of linear polynomials as follows:
- The remainder when p(x) is divided by x - a is p(a) (∵ x - a = 0 ⇒ x = a).
- The remainder when p(x) is divided by ax + b is p(-b/a) (∵ ax + b = 0 ⇒ x = -b/a).
- The remainder when p(x) is divided by ax - b is p(b/a) (∵ ax - b = 0 ⇒ x = b/a).
- The remainder when p(x) is divided by bx - a is p(a/b) (∵ bx - a = 0 ⇒ x = a/b).
Proof of Remainder Theorem
Let us assume that q(x) and 'r' are the quotient and the remainder respectively when a polynomial p(x) is divided by a linear polynomial (x - a). By division algorithm, Dividend = (Divisor × Quotient) + Remainder.
Using this, p(x) = (x - a) · q(x) + r.
Substitute x = a
p(a) = (a - a) · q(a) + r
p(a) = (0) · q(a) + r
p(a) = r
i.e. the remainder = p(a).
Hence, proved.
Remainder Theorem for Polynomials
The remainder can be easily found when a polynomial is divided by a linear polynomial. To find it, just substitute the zero of the linear polynomial in the dividend polynomial. To see how it works in the case of polynomials, let us consider the following example with two polynomials:
Dividend, p(x) : 6x4 - x3 + 2x2 - 7x + 2
Divisor : 2x + 3
Let us find the remainder in two ways:
- using the long division
- using the remainder theorem
Let us observe whether both answers are the same.
By using the long division of polynomials:

On dividing polynomials, the remainder that we have got is, r = 203/4
Now, let’s find the remainder by using the remainder theorem. i.e., first we find the zero of the linear polynomial (2x + 3 = 0 ⇒ x = -3/2) and then substitute it in the given polynomial. Then we get:
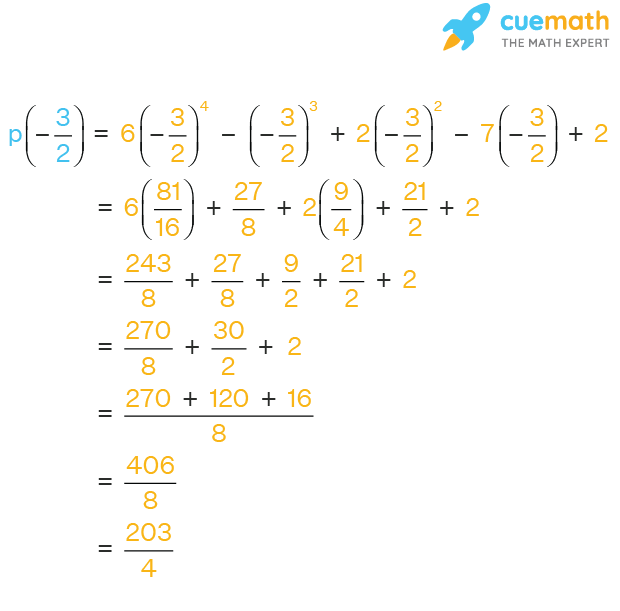
We have got the remainder to be 203/4 and the answer matched with the remainder found using the long division. Hence, the remainder theorem works wonders for polynomials.
Differences Between the Remainder Theorem and Factor Theorem
Here are the main differences between the remainder and factor theorems.
| Remainder Theorem | Factor Theorem | |
|---|---|---|
| Definition | The remainder theorem states that the remainder when p(x) is divided by (x - a) is p(a). | The factor theorem states that (x - a) is a factor of p(x) if and only if p(a) = 0. |
| Application | It is used to find the remainder. | It is used to decide whether a linear polynomial is a factor of the given polynomial or not. |
| Example | From the above example, the remainder when 6x4 - x3 + 2x2 - 7x + 2 is divided by 2x + 3 is 203/4. | Since the remainder in this example is NOT 0, 2x + 3 is NOT a factor of 6x4 - x3 + 2x2 - 7x + 2. |
The remainder and factor theorems together are used to solve/factorize polynomials.
Important Notes on Remainder Theorem:
- The remainder theorem says "when a polynomial p(x) is divided by a linear polynomial whose zero is x = k, the remainder is given by p(k)".
- The basic formula to check the division is: Dividend = (Divisor × Quotient) + Remainder.
- The remainder theorem does not work when the divisor is not linear.
- Also, it does not help to find the quotient.
☛ Related Articles:
Remainder Theorem Examples
-
Example 1: Find the remainder when p(x): 3x5 − x4 + x3 − 4x2 + 2 is divided by q(x): x − 1.
Solution:
We will substitute the zero of q(x) into the polynomial p(x) to find the remainder r. The zero of q(x) is x = 1.
So by remainder theorem, r = p(1)
= 3(1)5 - (1)4 + (1)3 - 4(1)2 + 2
= 3 - 1 + 1 - 4 + 2
= 1
Answer: 1
-
Example 2: Consider the following polynomial: p(x): x6 − 2x5 + x4 + 2x3 + x2 − 36. Evaluate the value of this polynomial when x is equal to 2. What can you infer from your answer?
Solution:
We have
p(2) = (2)6 − 2(2)5 + (2)2 + 2(2)3 + (2)2 − 36
= 64 – 64 + 16 + 16 + 4 − 36
= 0
By remainder theorem, p(2) is the remainder when p(x) is divided by (x - 2). Since p(2) = 0 ⇒ 0. Thus, we can conclude that (x - 2) is a factor of p(x).
Answer: p(2) = 0 and (x - 2) is a factor of p(x).
-
Example 3: Consider the following two polynomials:
a(x) : x3 − x2 + x − 1
b(x) : 2x + 1
Find the remainder polynomial when a(x) is divided by b(x) using two methods.
Solution:
Finding remainder by long division:
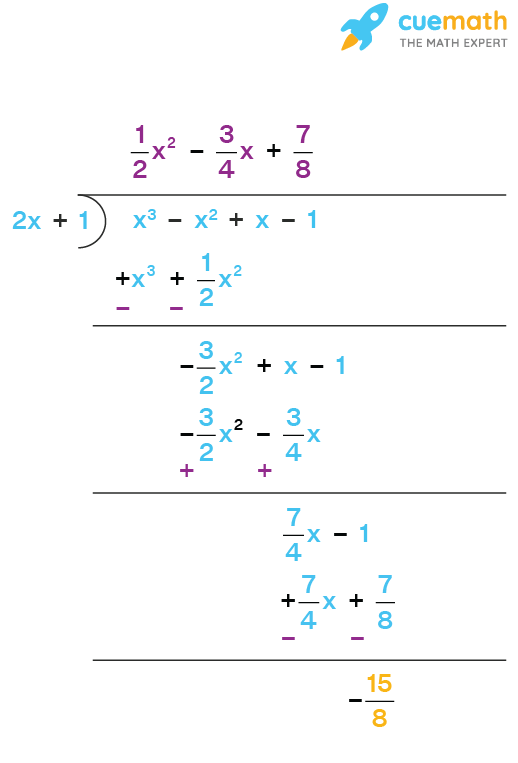
Thus, we have
q(x): 1/2x2 - 3/4x + 7/8
r = -15/8
Finding remainder by remainder theorem:
Putting x = -1/2 in x3 − x2 + x − 1,
we have,
=(-1/2)3- (-1/2)2 + (-1/2) -1
=(-1/8) - (1/4) - (1/2) -1
= -7/8 - 1
Remainder = -15/8
Note: The quotient cannot be found by using the remainder theorem.
Answer: The remainder obtained in both methods is -15/8.

FAQs on Remainder Theorem
State and Prove Remainder Theorem.
The remainder theorem states that when a polynomial p(x) is divided by (x - a), then the remainder = f(a). This can be proved by Euclid’s Division Lemma. By using this, if q(x) is the quotient and 'r' is the remainder, then p(x) = q(x) (x - a) + r. Substitute x = a on both sides, then we get p(a) = r, and hence the remainder theorem is proved.
How do You Use the Remainder Theorem?
To find the remainder when p(x) is divided by a linear polynomial (ax - b),
- Set ax - b = 0 and find x. Here, x = b/a.
- Substitute it in p(x). Then remainder = p (b/a).
What Are the Applications of the Remainder Theorem Formula?
The factor theorem is the main application of the remainder theorem formula. To prove the factor theorem, we need the remainder theorem. The factor theorem says if the remainder obtained by dividing p(x) by (x - r) is 0, then (x - r) is a factor of p(x).
How Does Polynomial Remainder Theorem Work?
To understand how the remainder theorem works, let us consider a general case. Let a(x) be the dividend polynomial and b(x) the linear divisor polynomial, and let q(x) be the quotient and r the constant remainder. Thus, we have a(x) = b(x) q(x) + r. Let us denote the zero of the linear polynomial b(x) by k. This means that b(k) = 0. If we plug in x as k in the starred relation above, we have a(k) = b(k) q(k) + r. Note that doing this is allowed since the starred relation holds true for every value of x. In fact, it is a polynomial identity. Since b(k)=0 we are left with a(k)=r. In other words, the remainder is equal to the value of a(x) when x is equal to k. Precisely what we stumbled upon!
This is exactly what the remainder theorem is: When a polynomial a(x) is divided by a linear polynomial b(x) whose zero is x equal to k, the remainder is given by r=a(k).
Are the Factor Theorem and the Remainder Theorem Same?
No, they are different. The factor theorem just ensures whether the given linear polynomial is a factor of the given polynomial, whereas the remainder theorem find the remainder when a polynomial is divided by another polynomial that is linear. To find more differences, click here.
What is the Use of the Remainder Theorem Class 9?
The remainder theorem in Class 9 is used to find the remainder when a polynomial p(x) is divided by (ax + b). The remainder theorem is further extended to prove the factor theorem where we can determine whether (ax + b) is a factor of p(x) or not. If the remainder is 0, then (ax + b) is a factor of a polynomial p(x), otherwise, it is not.
Who Invented the Remainder Theorem?
Chinese mathematician Sun Zi invented the remainder theorem. The complete remainder theorem was given by Qin Jiushao in 1247.
What if the Remainder Is Zero?
If the remainder is zero, then the remaining quotient and the divisor are the factors of the given expression.
What is the Remainder Theorem Formula?
The general formula for polynomial remainder theorem is represented as, when p(x) is divided by (ax + b) then the remainder = p (-b/a).
What is the Use of the Factor Theorem?
Factor theorem is used in math to find the factors of a given polynomial equation. Let's say hypothetically, if f(x) is a polynomial, putting f(k) = 0, then (x – k) is a factor of f(x).
visual curriculum