Division of Fractions
Division means sharing an item equally. We have learned about the division of whole numbers, now let us see how to divide fractions. A fraction has two parts - a numerator and a denominator. Dividing fractions is almost the same as multiplying them. For the division of fractions, we multiply the first fraction by the reciprocal (inverse) of the second fraction. Let us learn more about the division of fractions in this article.
How to Divide Fractions?
We know that division is a method of sharing equally and putting into equal groups. We divide a whole number by the divisor to get the quotient. Now, when we do division of a fraction by another fraction, it is the same as multiplying the fraction by the reciprocal of the second fraction. The reciprocal of a fraction is a simple way of interchanging the fraction's numerator and denominator. Observe the following figure to learn a simple rule of dividing fractions.
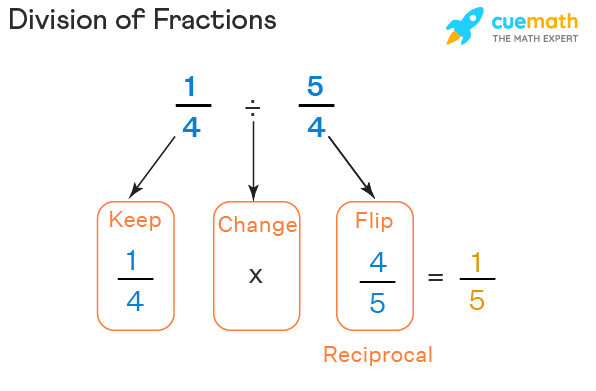
In the subsequent sections, we will learn the division of fractions with fractions, whole numbers, decimals, and mixed numbers. In every case, we will be using the same rule of dividing fractions as given above. Let's begin!
Dividing Fractions by Fractions
We just learned how to divide fractions by taking the reciprocal. Now, let us see the method of dividing fractions by fractions with an example. Have a look at the formula of the division of a fraction by fraction given below. If x/y is divided by a/b, this implies,
x/y ÷ a/b
⇒ x/y × b/a (reciprocal of a/b is b/a)
⇒ xb/ya
Now, if we need to divide: 5/8 ÷ 15/16, we will substitute the values of the given numerators and denominators.
5/8 ÷ 15/16 = 5/8 × 16/15 = 2/3
∴ The value of 5/8 ÷ 15/16 = 2/3.
Division of Fractions with Whole Numbers
For the division of fractions with whole numbers, we need to multiply the denominator of the given fraction with the given whole number. In the general form, if x/y is the fraction and a is the whole number, then x/y ÷ a = x/y × 1/a = x/ya.
Let us take an example and divide 2/3 with 4.
2/3 ÷ 4 = 2/3 × 1/4
= 1/6
Therefore, 2/3 ÷ 4 gives us 1/6. This is how we divide fractions with whole numbers.
Dividing Fractions with Decimals
We know that decimal numbers themselves are a fraction to base 10. We can represent the decimal in the fractional form and then perform the division. For dividing fractions with decimals, follow the steps given below:
- Convert the given decimal to a fraction.
- Divide both the fractions.
Consider the example, 4/5 ÷ 0.5. Here, 0.5 can be written in fractional form as 5/10 or 1/2. Now, divide 4/5 by 1/2. This implies, 4/5 ÷ 1/2 = 4/5 × 2/1 = 8/5. This is how we perform the division of fractions with decimals. Now let us learn how to divide fractions with mixed numbers.
Division of Fractions and Mixed Numbers
We have learned how to convert mixed fractions to improper fractions. For the division of fractions with mixed numbers, we have to convert the mixed fraction to an improper fraction first and then divide them as we divide two fractions. Consider the following example.
3/4 ÷ \(1\dfrac{1}{2}\)
So, the first step is to convert \(1\dfrac{1}{2}\) to an improper fraction. \(1\dfrac{1}{2}\) is the same as 3/2. Now, it can be solved in the following way:
3/4 ÷ 3/2
⇒ 3/4 × 2/3
⇒ 6/12 = 1/2
Therefore, 3/4 ÷ \(1\dfrac{1}{2}\) = 1/2. If you want to divide a mixed number with a fraction, first convert the mixed number to an improper fraction and follow the same steps as shown above.
Division of Fractions Related Articles
Check these interesting articles related to the concept of division of fractions in math.
FAQs on Dividing Fractions
What does Division of Fractions Mean?
The division of fractions means breaking down a fraction into further parts. For example, if you take half (1/2) of a pizza and you further divide it into 2 equal parts, then each portion will be 1/4th of the whole pizza. Mathematically, we can express this reasoning as 1/2 ÷ 2 = 1/4.
What is Multiplication and Division of Fractions?
The multiplication of fractions means to add a fraction to itself repeatedly a specific number of times. The following steps are used to multiply fractions:
- Step 1: Multiply the numerators of both the fractions.
- Step 2: Multiply the denominators of both the fractions.
- Step 3: Simplify the fraction obtained after multiplication.
On the other hand, the division of fractions means to do equal grouping or equal sharing of a fraction. Dividing fractions is related to multiplication, as while dividing two fractions, we multiply the reciprocal of the second fraction to the first.
How to Visualize Division of Fractions?
To visualize the division of fractions, take a piece of paper and fold it into two equal parts. Cut 1/2 of the paper with scissors. Now, you will be left with 1/2 of the paper. Now, again divide that 1/2 portion into 2 equal parts. After this, you will be left with 1/4th of the paper. That is the answer of 1/2 ÷ 2. This is how you can visualize the concept of dividing fractions.
What is the Rule for Dividing Fractions?
The basic rule of dividing fractions is to keep, change, and flip. It means we have to keep the first fraction as it is, change the division sign to the multiplication sign, and flip the second fraction to its reciprocal. By following this simple rule, you can divide any two fractions.
What are the Steps to Dividing Fractions?
The following steps have to be followed in order to divide fractions:
- Step 1: Take the reciprocal of the second fraction.
- Step 2: Multiply it with the first fraction.
- Step 3: Reduce the resultant fraction to its lowest terms.
How to Teach Division of Fractions?
Division of fractions can be taught in many ways such as, by using models or applying the concept of multiplication of fractions. Some of the ways to teach how to divide fractions are listed below:
- Take circular or rectangular fraction models to demonstrate the concept of division of fractions to your learners.
- Use worksheets including pictures and word problems.
- Use materials from day-to-day lives like beans, leaves, pebbles, etc to show learners how to divide fractions.
How to Divide a Number by a Fraction?
To divide a whole number by a fraction, we multiply the whole number with the reciprocal of the given fraction. To divide a fraction by a fraction, we multiply the reciprocal of the second fraction with the first fraction.
How to do Division of Fractions with Whole Numbers?
Dividing fractions with whole numbers is a three-step process:
- Step 1: Keep the fraction as it is. For example, 3/4 ÷ 6.
- Step 2: Flip the whole number, which will make it a fraction of the format 1/a. In this case, 6 will become 1/6.
- Step 3: Change the sign into multiplication. We will get 3/4 × 1/6 = 3/24 = 1/8.
How to do Division of Fractions With Mixed Numbers?
For the division of fractions and mixed numbers, the following steps are used:
- Step 1: Keep the fraction as it is.
- Step 2: Convert the mixed number into an improper fraction and flip the second fraction.
- Step 3: Change the sign into multiplication between the fractions. Multiply and simplify them.