Whole Numbers
Whole numbers are a set of numbers including all natural numbers and 0. They are a part of real numbers that do not include fractions, decimals, or negative numbers. Counting numbers are also considered as whole numbers. Let us learn everything about whole numbers, the whole numbers definition, along with whole number examples in this article.
| 1. | What are Whole Numbers? |
| 2. | Natural Numbers and Whole Numbers |
| 3. | Whole Numbers on Number Line |
| 4. | Properties of Whole Numbers |
| 5. | FAQs on Whole Numbers |
What are Whole Numbers?
Natural numbers along with zero (0) are referred to as whole numbers. We know that natural numbers refer to a set of counting numbers starting from 1, 2, 3, 4 and so on. In simple words, whole numbers are a set of numbers without fractions, decimals, or even negative integers. It is a collection of positive integers and zero. Or we can say that whole numbers are the set of non-negative integers. The primary difference between natural and whole numbers is the presence of zero in the whole numbers set.
Whole Number Definition
Whole Numbers are those counting positive numbers that start from 0 and go on till infinity. For example, 0, 1, 2, 3, 4, and so on.
Set of Whole Numbers
The set of whole numbers includes the set of natural numbers along with the number 0. The set of whole numbers in mathematics is given as {0, 1, 2, 3, ...} which is denoted by the symbol W.
W = {0, 1, 2, 3, 4, …}
Here are some facts about whole numbers, which will help you understand them better:
- All natural numbers are whole numbers.
- All counting numbers are whole numbers.
- All positive integers including zero are whole numbers.
- All whole numbers are real numbers.
- Every whole number is a rational number.
Whole Number Symbol
The symbol used to represent whole numbers is the alphabet ‘W’ in the uppercase, W = 0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, …
Smallest Whole Number
0 is the smallest whole number because whole numbers start from 0 (from the definition of whole numbers). Zero is a number that lies between the positive and negative numbers on a number line. Although zero carries no value, it is used as a placeholder. So, zero is neither a positive number nor a negative number.
List of Whole Numbers
The list of whole numbers starts from 0, 1, 2, 3, 4, 5, and goes on till infinity.
Natural Numbers and Whole Numbers
From the above definitions, we can understand that every whole number other than 0 is a natural number. Also, every natural number is a whole number. So, the set of natural numbers is a part of the set of whole numbers or a subset of whole numbers.
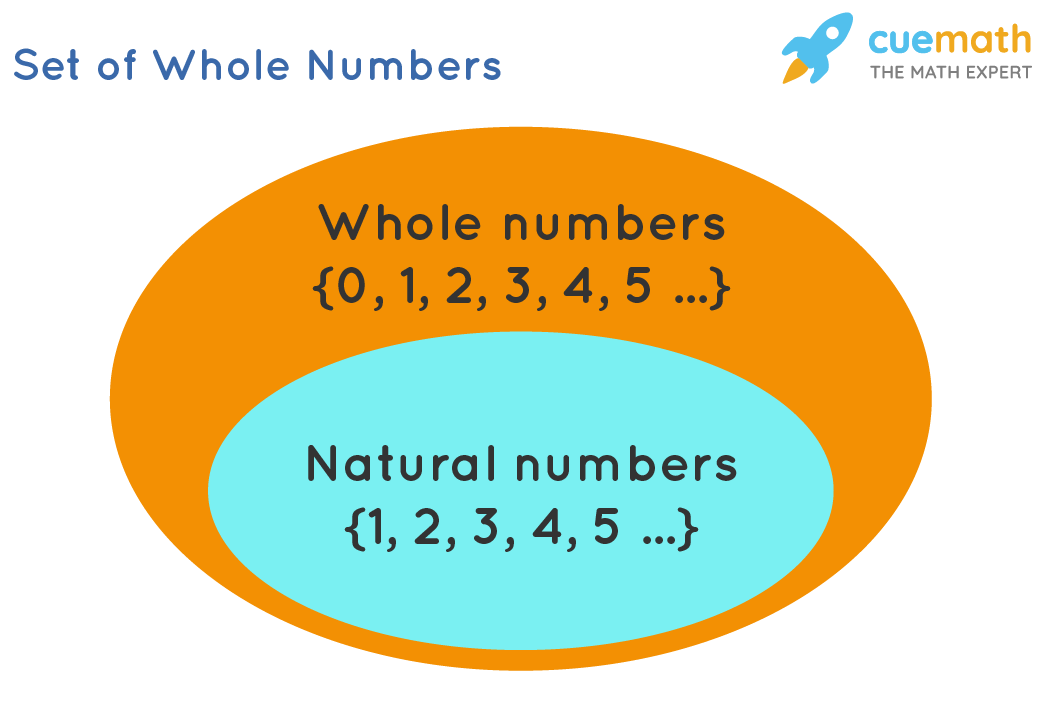
Difference Between Whole numbers and Natural Numbers
Let us understand the difference between natural numbers and whole numbers with the help of the table given below:
| Whole Number | Natural Number |
|---|---|
| The set of whole numbers is, W = {0,1,2,3,...}. | The set of natural numbers is, N = {1,2,3,...}. |
| The smallest whole number is 0. | The smallest natural number is 1. |
| Every natural number is a whole number. | Every whole number is a natural number, except 0. |
Whole Numbers on Number Line
The set of natural numbers and the set of whole numbers can be shown on the number line as given below. All the positive integers or the integers on the right-hand side of 0 represent the natural numbers, whereas all the positive integers along with zero, altogether represent the whole numbers. Both sets of numbers can be represented on the number line as follows:

Properties of Whole Numbers
The basic operations on whole numbers: addition, subtraction, multiplication, and division, lead to four main properties of whole numbers that are listed below:
- Closure Property
- Associative Property
- Commutative Property
- Distributive Property
Closure Property
The sum and product of two whole numbers is always a whole number. For example, 7 + 3 = 10 (whole number), 7 × 2 = 14 (whole number).
Associative Property
The sum or product of any three whole numbers remains the same even if the grouping of numbers is changed. For example, when we add the following numbers we get the same sum: 10 + (7 + 12) = (10 + 7) + 12 = 29. Similarly, when we multiply the following numbers we get the same product no matter how the numbers are grouped: 3 × (2 × 4) = (3 × 2) × 4 = 24.
Commutative Property
The sum and the product of two whole numbers remain the same even after interchanging the order of the numbers. This property states that a change in the order of addition does not change the value of the sum. Let 'a' and 'b' be two whole numbers. According to the commutative property a + b = b + a. For example, a = 10 and b = 19 ⇒ 10 + 19 = 19 + 10 = 29 . This property also holds true for multiplication, but not for subtraction and division. For example: 7 × 9 = 63 and 9 × 7 = 63.
Additive Identity
When a whole number is added to 0, its value remains unchanged, i.e., if a is a whole number then a + 0 = 0 + a = a. For example, 3 + 0 = 3 + 0 = 3.
Multiplicative Identity
When a whole number is multiplied by 1, its value remains unchanged, i.e., if a is a whole number then a × 1 = a = 1 × a. For example. 4 × 1 = 1 × 4 = 4.
Distributive Property
This property states that the multiplication of a whole number is distributed over the sum or difference of the whole numbers. It means that when two numbers, for example, a and b are multiplied with the same number c, and are then added, then the sum of a and b can be multiplied by c to get the same answer. This property can be represented as: a × (b + c) = (a × b) + (a × c). Let a = 10, b = 20 and c = 7 ⇒ 10 × (20 + 7) = 270 and (10 × 20) + (10 × 7) = 200 + 70 = 270. The same property is true for subtraction as well. For example, we have a × (b − c) = (a × b) − (a × c). Let a = 10, b = 20 and c = 7 ⇒ 10 × (20 − 7) = 130 and (10 × 20) − (10 × 7) = 200 − 70 = 130.
Multiplication by Zero
When a whole number is multiplied by 0, the result is always 0, i.e., a × 0 = 0 × a = 0. For example, 4 × 0 = 0.
Division by Zero
The division of a whole number by 0 is not defined, i.e., if a is a whole number then a/0 is not defined.
For more information about the properties of whole numbers, click on the link provided.
Important Points related to Whole numbers
- 0 is a whole number and not a natural number.
- The first five whole numbers are 0, 1, 2, 3, 4.
- The smallest whole number is 0.
- Whole numbers do not include negative numbers, fractions, and decimals.
☛ Related Topics
- Rational Numbers
- Prime Numbers
- Composite Numbers
- Even Numbers
- Odd Numbers
- Real Numbers
- Natural Numbers
- Irrational Numbers
- Counting Numbers
- Cardinal Numbers
Cuemath is one of the world's leading math learning platforms that offers LIVE 1-to-1 online math classes for grades K-12. Our mission is to transform the way children learn math, to help them excel in school and competitive exams. Our expert tutors conduct 2 or more live classes per week, at a pace that matches the child's learning needs.
Whole Numbers Examples
-
Example 1: Identify the whole numbers among the following numbers ( -1, 0, 3, 1/2, 5).
Solution:
The set of whole numbers in mathematics is: {0, 1, 2, 3, ...}. Therefore, among the given numbers, the whole numbers are, (0, 3, and 5).
-
Example 2: State true or false with reference to whole numbers.
a.) 0 is a whole number.
b.) Every natural number is a whole number.
c.) Every whole number is a rational number.
d.) Every whole number is an integer.
e.) Every number is a whole number.
Solution:
a.) True, 0 is a whole number.
b.) True, every natural number is a whole number.
c.) True, every whole number is a rational number.
d.) True, every whole number is an integer.
e.) False, every number may not necessarily be a whole number. Whole numbers are a set of numbers that include only natural numbers and 0. They are a part of real numbers that do not include fractions, decimals, or negative numbers.
-
Example 3: Fill in the blanks with reference to whole numbers.
a.) The set of whole numbers is represented as _____.
b.) When a whole number is multiplied by 0, the result is always __
c.) Find the mean of the first five whole numbers _
d.) _ is the whole number which is not a natural number.
Solution:
a.) The set of whole numbers is represented as, W = {0,1,2,3,...}
b.) When a whole number is multiplied by 0, the result is always 0.
c.) The mean of the first five whole numbers is 2. This is because the first five whole numbers are 0, 1, 2, 3, and 4. So, (0 + 1 + 2 + 3 + 4) ÷ 5 = 10/5 = 2
d.) 0 is the whole number which is not a natural number.

FAQs on Whole Numbers
What are Whole Numbers in Math?
Whole numbers, in math, include positive integers and 0. In other words, it is a set of natural numbers and 0. Decimals, fractions, and negative integers are not a part of whole numbers.
Can Whole Numbers be Negative?
The set of whole numbers in mathematics is represented as {0, 1, 2, 3, ...}. Hence, whole numbers cannot be negative. The smallest whole number is 0.
What is the Difference Between Natural and Whole Numbers?
Natural numbers are numbers used for counting. The set of natural numbers in mathematics is expressed as, {1, 2, 3, ...}. Whereas the whole numbers are the set of natural numbers and 0, which is expressed as, W = {0, 1, 2, 3, ...}. This shows that natural numbers start from 1, 2, 3 4, and so on, whereas whole numbers include zero (0) and they start from 0, 1, 2, 3, 4, and so on.
Where do Whole Numbers Start from?
The whole numbers start from 0 and go up to infinity. For example, 0, 1, 2, 3, 4, 5, and so on. So, in this way, the smallest whole number is 0.
What is the Symbol for the Set of Whole Numbers?
W is the symbol of the whole numbers set. Mathematically, it can be represented as W = {0, 1, 2, 3, 4, 5, 6, 7, ...}.
What are Some Examples of Whole Numbers?
The set of whole numbers is: {0, 1, 2, 3, ...}. Any value that is included in this set is a whole number example. Some examples are 0, 15, 53, etc.
Which Whole Number is not a Natural Number?
0 (Zero) is a whole number which is not a natural number. This is the number that differentiates natural numbers from whole numbers.
How are Integers Different from Whole Numbers?
Integers are different from whole numbers because integers include positive numbers, negative numbers and zero. A few examples of integers are -5, 0, -34, 89, and so on. However, whole numbers do not include negative numbers, they just include positive numbers and zero. A few examples of whole numbers are 98, 34, 0, 5, and so on.
How to Multiply Fractions with Whole Numbers?
Fractions can be multiplied with whole numbers using the same method of multiplication of fractions. The only change is that the whole number needs to be written in the form of a fraction by making the whole number the numerator and writing 1 as its denominator. For example, if we need to multiply 4 × 1/8, we just need to write 4 as 4/1 and then multiply it normally. This will be 4/1 × 1/8 = 1/2
How are Whole Numbers used in Everyday Life?
Whole numbers are used in our everyday lives. For example, if we need to count the number of apples in our basket, or, if we need to count the number of notebooks we have, or, if we need to know the number of students in our class, we need whole numbers.
What is the Smallest Whole Number?
The smallest whole number is 0 because whole numbers are positive counting numbers that start from 0 and go on till infinity.
What is the Meaning of a Whole Number?
A whole number means a number that does not include any fractions, negative numbers or decimals. It includes complete or whole numbers like 4, 67, 12, and so on. This is the reason for which these are called whole numbers.
What are the First Five Whole Numbers?
The first five whole numbers can be listed as 0, 1, 2, 3, and 4 because whole numbers start from 0 and go on till infinity.
visual curriculum