Integers
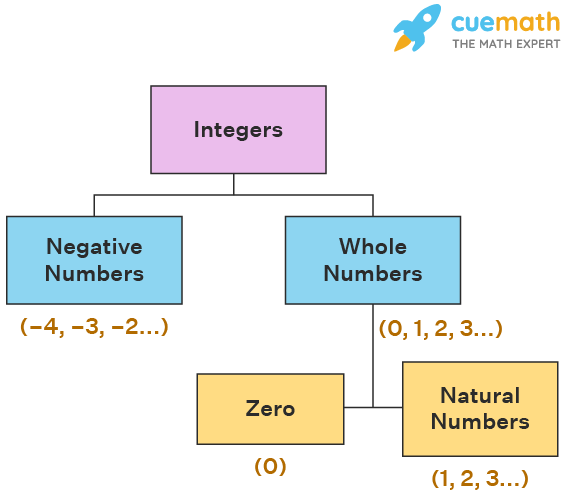
Integers include positive numbers, negative numbers, and zero. 'Integer' is a Latin word which means 'whole' or 'intact'. This means integers do not include fractions or decimals. Let us learn more about integers, the definition of integers, meaning of integers, and the properties of integers in this article.
What are Integers?
Integers include all whole numbers and negative numbers. This means if we include negative numbers along with whole numbers, we form a set of integers.
Integers Definition
An integer is a number with no decimal or fractional part and it includes negative and positive numbers, including zero. A few examples of integers are: -5, 0, 1, 5, 8, 97, and 3,043. A set of integers, which is represented as Z, includes:
- Positive Numbers: A number is positive if it is greater than zero. Example: 1, 2, 3, . . .
- Negative Numbers: A number is negative if it is less than zero. Example: -1, -2, -3, . . .
- Zero is defined as neither a negative number nor a positive number. It is a whole number.
Set of Integers
The set of integers is represented by the letter Z and it is written as shown below:
Z = {... -7, -6, -5, -4, -3, -2, -1, 0, 1, 2, 3, ...}
Observe the figure given below to understand the definition of integers.
Integers on a Number Line
A number line is a visual representation of numbers on a straight line. This line is used for the comparison of numbers that are placed at equal intervals on an infinite line that extends on both sides, horizontally. Just like other numbers, the set of integers can also be represented on a number line.

Graphing Integers on a Number Line
Positive and negative integers can be visually represented on a number line. Integers on a number line help in performing arithmetic operations. The basic points to keep in mind while placing integers on a number line are as follows:
- The number on the right side is always greater than the number on the left side.
- Positive numbers are placed on the right side of 0, because they are greater than 0.
- Negative numbers are placed on the left side of 0, because they are smaller than 0.
- Zero, which is not positive or negative, is usually kept in the middle.
Integer Operations
The four basic arithmetic operations associated with integers are:
- Addition of Integers
- Subtraction of Integers
- Multiplication of Integers
- Division of Integers
There are some rules for performing these operations of integers. Before we start learning these methods of integer operations, we need to remember a few things.
- If there is no sign in front of a number, it means that the number is positive. For example, 5 means +5.
- The absolute value of an integer is a positive number, i.e., |−2| = 2 and |2| = 2.
Addition of Integers
Adding integers is the process of finding the sum of two or more integers where the value might increase or decrease depending on the integer being positive or negative. The different rules and the possible cases for the addition of integers are given in the following section.
Rules of Integers in Addition
While adding two integers, we use the following rules:
- When both integers have the same signs: Add the absolute values of integers, and give the same sign as that of the given integers to the result.
- When one integer is positive and the other is negative: Find the difference of the absolute values of the numbers and then give the sign of the larger of these numbers to the result.
Example: Add the given integers: 2 + (-5)
Solution:
Here, the absolute values of 2 and (-5) are 2 and 5 respectively.
Their difference (larger number - smaller number) is 5 - 2 = 3
Now, among 2 and 5, 5 is the larger number and its original sign “-”.
Hence, the result gets a negative sign, "-”.
Therefore, 2 + (-5) = -3
Example: Add the given integers: (-2) + 5
Solution:
Here, the absolute values of (-2) and 5 are 2 and 5 respectively. Their difference (larger number - smaller number) is 5 - 2 = 3. Now, among 2 and 5, 5 is the larger number and its original sign “+”. Hence, the result will be a positive value. Therefore,(-2) + 5 = 3
We can also solve the above problem using a number line. The rules for the addition of integers on the number line are as follows.
- Always start from 0.
- Move to the right side, if the second number is positive.
- Move to the left side, if the second number is negative.
Example: Find the value of 5 + (-10) using a number line.
Solution:
In the given problem, the first number is 5 which is positive. So, we start from 0 and move 5 units to the right side.
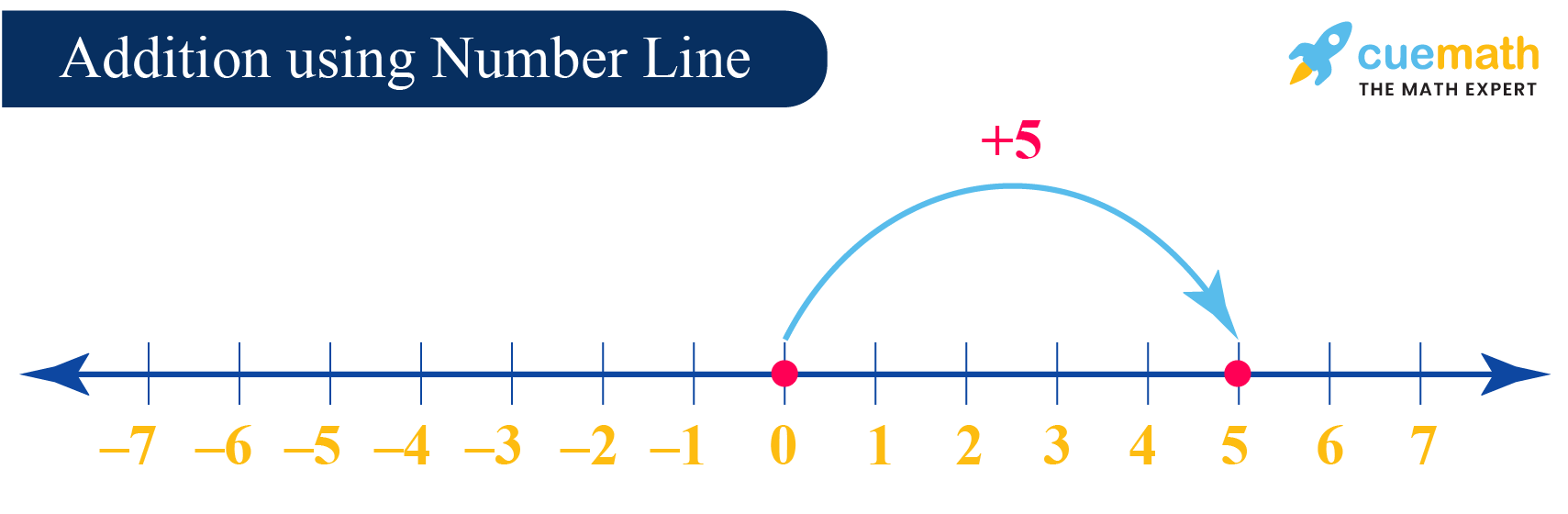
The next number in the given problem is -10, which is negative. We move 10 units to the left side from 5.

Finally, we reach at -5. Therefore, the value of 5 + (-10) = -5
Subtraction of Integers
Subtracting integers is the process of finding the difference between two or more integers where the final value might increase or decrease depending on the integer being positive or negative. The different rules and the possible cases for the subtraction of integers are given in the following section.
Rules of Integers in Subtraction
In order to carry out the subtraction of two integers, we use the following rules:
- Convert the operation into an addition problem by changing the sign of the subtrahend.
- Apply the same rules of addition of integers and solve the problem thus obtained in the above step.
Example: Subtract the given integers: 7 - 10
Solution: 7 - 10 can be written as (+ 7) - (+)10
- Convert the given expression into an addition problem and change the sign of the subtrahend, so, we get: 7 + (-10)
- Now, the rules for this operation will be the same as for the addition of two integers.
- Here, the absolute values of 7 and (-10) are 7 and 10 respectively.
- Their difference (larger number - smaller number) is 10 - 7 = 3.
- Now, among 7 and 10, 10 is the larger number and its original sign “-”.
- Hence, the result gets a negative sign, "-”.
- Therefore, 7 - 10 = -3
Multiplication of Integers
For the multiplication of integers, we use the following rules given in the table. The different rules and the possible cases for the multiplication of integers are given in the following section.
Rules of Integers in Multiplication
In order to carry out the multiplication of two integers, we use the following rules:
| Product of Signs | Result | Example |
|---|---|---|
| (+) × (+) | + | 2 × 3 = 6 |
| (+) × (-) | - | 2 × (-3) = -6 |
| (-) × (+) | - | (-2) × 3 = -6 |
| (-) × (-) | + | (-2) × (-3) = 6 |
Example: Multiply (-6) × 3
Solution: Using the rules of multiplication of integers, when we multiply a positive and negative integer, the product has a negative sign.
Therefore, (-6) × 3 = -18
Division of Integers
Division of integers means equal grouping or dividing an integer into a specific number of groups. For the division of integers, we use the rules given in the following table. The different rules and the possible cases for the division of integers are given in the following section
Rules of Integers in Division
In order to carry out the division of two integers, we use the following rules.
| Division of Signs | Result | Example |
|---|---|---|
| (+) ÷ (+) | + | 12 ÷ 3 = 4 |
| (+) ÷ (-) | - | 12 ÷ (-3) = -4 |
| (-) ÷ (+) | - | (-12) ÷ 3 = -4 |
| (-) ÷ (-) | + | (-12) ÷ (-3) = 4 |
Example: Divide (-15) ÷ 3
Solution: Using the rules of division of integers, when we divide a negative integer by a positive integer, the quotient has a negative sign.
Therefore, (-15) ÷ 3 = -5
Integers Worksheets
Download integers worksheets, including addition and subtraction of integers, adding and subtracting multiple integers, and multiplication and division of integers.
- Integers Worksheets for Grade 7
- Integers Worksheets for Grade 6
- Adding and Subtracting Integers Worksheet Grade 5
- Adding and Subtracting Integers Worksheet Grade 7
Properties of Integers
The main properties of Integers are as follows:
- Closure Property
- Associative Property
- Commutative Property
- Distributive Property
- Additive Inverse Property
- Multiplicative Inverse Property
- Identity Property
Closure Property
The closure property states that the set is closed for any particular mathematical operation. Z is closed under addition, subtraction, multiplication, and division of integers. For any two integers, a and b:
- a + b ∈ Z
- a - b ∈ Z
- a × b ∈ Z
- a/b ∈ Z
Associative Property
According to the associative property, changing the grouping of two integers does not change the result of the operation. It is to be noted that the associative property applies only to the addition and multiplication of two integers.
For any two integers, a and b:
- a + (b + c) = (a + b) + c
- a × (b × c) = (a × b) × c
Commutative Property
According to the commutative property, changing the position of the operands in an operation does not affect the result. It is to be noted that the commutative property is applicable only to the addition and multiplication of integers.
For any two integers, a and b:
- a + b = b + a
- a × b = b × a
Distributive Property
The distributive property states that for any expression of the form a (b + c), which means a × (b + c), operand a can be distributed among the operands b and c as: (a × b) + (a × c) that is,
a × (b + c) = (a × b) + (a × c)
Additive Inverse Property
The additive inverse property states that the addition operation between any positive integer and its negative value will give the result as zero (0).
For any integer, a:
a + (-a) = 0
Multiplicative Inverse Property
The multiplicative inverse property states that the multiplication operation between any integer and its reciprocal will give the result as one (1).
For any integer, a: a × 1/a = 1
Identity Property
Integers follow the identity property for addition and multiplication operations. The additive identity property states that when zero is added to an integer, it results in the integer itself. This means, a + 0 = a
Similarly, the multiplicative identity states that when 1 is multiplied to any integer, it results in the integer itself. This means, a × 1 = a
☛ Related Articles
- Addition and Subtraction of Integers
- Multiplication and Division of Integers
- Positive and Negative Integers Worksheets
- Integer Formulas
- Consecutive Integers Formula
- Sum of Integers Formula
- Multiplying Integers Calculator
- Subtracting Integers Calculator
- Dividing Integers Calculator
Cuemath is one of the world's leading math learning platforms that offers LIVE 1-to-1 online math classes for grades K-12. Our mission is to transform the way children learn math, to help them excel in school and competitive exams. Our expert tutors conduct 2 or more live classes per week, at a pace that matches the child's learning needs.
Integers Examples
-
Example 1: Can you identify the property of the integer used in the following expression? 3 + (7 + 2) = (3 + 7) + 2
Solution:
The given expression, 3 + (7 + 2) = (3 + 7) + 2, shows the associative property of integers, which says that changing the grouping of two integers does not change the result of the operation because 3 + (7 + 2) = (3 + 7) + 2 = 12.
-
Example 2: Add the following integers: (-9) + (-5)
Solution:
According to the rules of integers in addition, when both the integers have the same signs, we add the absolute values of integers and give the same sign as that of the given integers to the result. The absolute values of the given integers is 9 and 5. So we will add 9 + 5 = 14 and the sign of the sum will be negative. Therefore, (-9) + (-5) = -14
-
Example 3: State true or false with reasons:
a.) Every integer is a natural number.
b.) Every integer is a whole number.
c.) Every integer is a rational number.
Solution:
a.) False, every integer is not a natural number because integers include negative numbers and zero, however, natural numbers are positive counting numbers that start from 1, 2, 3, and so on.
b.)False, every integer is not a whole number because integers include negative numbers, however, whole numbers are positive numbers that start from 0, 1, 2, 3, 4, and so on.
c.) True, every integer is a rational number because every integer can be expressed in the form of p/q such that q is not equal to 0.

FAQs on Integers
What are Integers in Math?
An integer is a number that includes negative and positive numbers, including zero. It does not include any decimal or fractional part. A few examples of integers are: -5, 0, 1, 5, 8, 97, and 3,043.
What are the Different Types of Integers?
There are generally three types of integers:
- Positive Integers: An integer is positive if it is greater than zero. Example 1, 2, 3 . . .
- Negative Integers: An integer is negative if it is less than zero. Example -1, -2, -3 . . .
- Zero is defined as neither a negative integer nor a positive integer.
Can a Negative Number be an Integer?
Yes, a negative number can also be an integer, but it should not have a decimal or fractional part. For example: Negative numbers: -2, -234, -71, etc., are all integers.
What are Consecutive Integers?
The integers that follow each other in order are called consecutive integers. For example, 2, 3, 4, and 5 are four consecutive integers.
What is the Rule for Adding a Positive and Negative Integer?
According to the rules of integers in addition, when one integer is positive and the other is negative, we find the difference of the absolute values of the numbers and then give the sign of the larger number to the result. For example, if we need to add 6 + (-4), we will find the difference of the absolute values of the given integers, that is, the difference between 6 and 4 is 2, and the result will have a positive sign because the larger number (6) has a positive sign.
What are the Properties of Integers?
Various arithmetic operations can be performed on integers, like addition, subtraction, multiplication and division. The major properties of integers associated with these different operations are as follows and the detailed explanation of these properties is given above on this page:
- Closure Property
- Associative Property
- Commutative Property
- Distributive Property
- Additive Inverse Property
- Multiplicative Inverse Property
- Identity Property
What are the Applications of Integers?
The application of positive and negative numbers in the real world is different. While positive numbers are commonly used everywhere, the negative integers are used in measuring temperature which can also have a negative value, that is, the temperature of a city can be -4°C or -10°C. The negative and positive numbers and zero in the scale denote different temperature readings. Bank credit and debit statements also use integers to represent the negative or positive values in transactions.
Why is the Set of Integers called Z?
The set of integers is called Z because the 'Z' stands for Zahlen, a German word which means numbers.
What is a Negative Integer?
A negative integer is an integer that is less than zero and has a negative sign before it. For example, -56, -12, -3, and so on are negative integers.
What is a Positive Integer?
An integer is said to be positive when it is greater than zero and it has a positive sign or no sign before it. For example, 34, 78, 9, and so on are positive integers.
visual curriculum