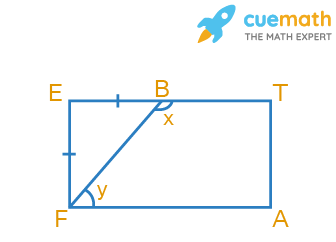
A playground is in the form of a rectangle ATEF. Two players are standing at the points F and B where EF = EB. Find the values of x and y.
A playground is in the form of a rectangle ATEF. Two players are standing at the points F and B where EF = EB. Find the values of x and y

Solution:

Given, ATEF is a rectangle.

Two players are standing at the points F and B where EF = EB.

We have to find the values of x and y.

We observe that EFB is an isosceles triangle since EF = FB.

By angle sum property of a triangle,

∠EFB + ∠EBF + ∠FEB = 180°

We know that each angle of a rectangle is equal to 90°.

So, ∠EFB + ∠EBF + 90° = 180°

We know that the angles opposite to the equal sides are equal.

∠EFB + ∠EFB = 180° - 90°

2∠EFB = 90°

∠EFB = 45°

Also, ∠EBF = 45°

We know that the linear pair of angles are supplementary.

So, ∠EBF + x = 180°

45° + x = 180°

x = 180° - 45°

x = 135°

Similarly, ∠EFB + y = 90°

45° + y = 90°

y = 90° - 45°

y = 45°

Therefore, the values of x and y are 135° and 45°.

✦ Try This: Each side of a rhombus is 10 cm. If one of its diagonals is 16 cm, find the length of the other diagonal.

☛ Also Check: NCERT Solutions for Class 8 Maths

NCERT Exemplar Class 8 Maths Chapter 5 Problem 156

A playground is in the form of a rectangle ATEF. Two players are standing at the points F and B where EF = EB. Find the values of x and y.

Summary:

A playground is in the form of a rectangle ATEF. Two players are standing at the points F and B where EF = EB. The values of x and y are 135° and 45°.

☛ Related Questions:

Math worksheets and
visual curriculum