Gradient Definition
In this mini-lesson, we shall explore the world of the gradient, by finding answers to questions like what is a gradient, what is a directional derivative, and understanding the properties of gradients with examples.
Let us understand how the gradient of line changes with a change in inclination of the line.
In this visualizer, move point B, and observe the change in the gradient of the line.
On moving the point B in the above simulation, the following three changes can be observed.
(a) coordinates of the point B
(b) the angle of the line
(c) the slope of the line
Lesson Plan
What Is the Definition of Gradient?
The gradient is the inclination of a line. The gradient is often referred to as the slope (m) of the line. The gradient or slope of a line inclined at an angle \(\theta \) is equal to the tangent of the angle \(\theta \).

\[ m = tan\theta \]
The gradient can be calculated geometrically for any two points \((x_1, y_1)\), \((x_2, y_2)\) on a line.
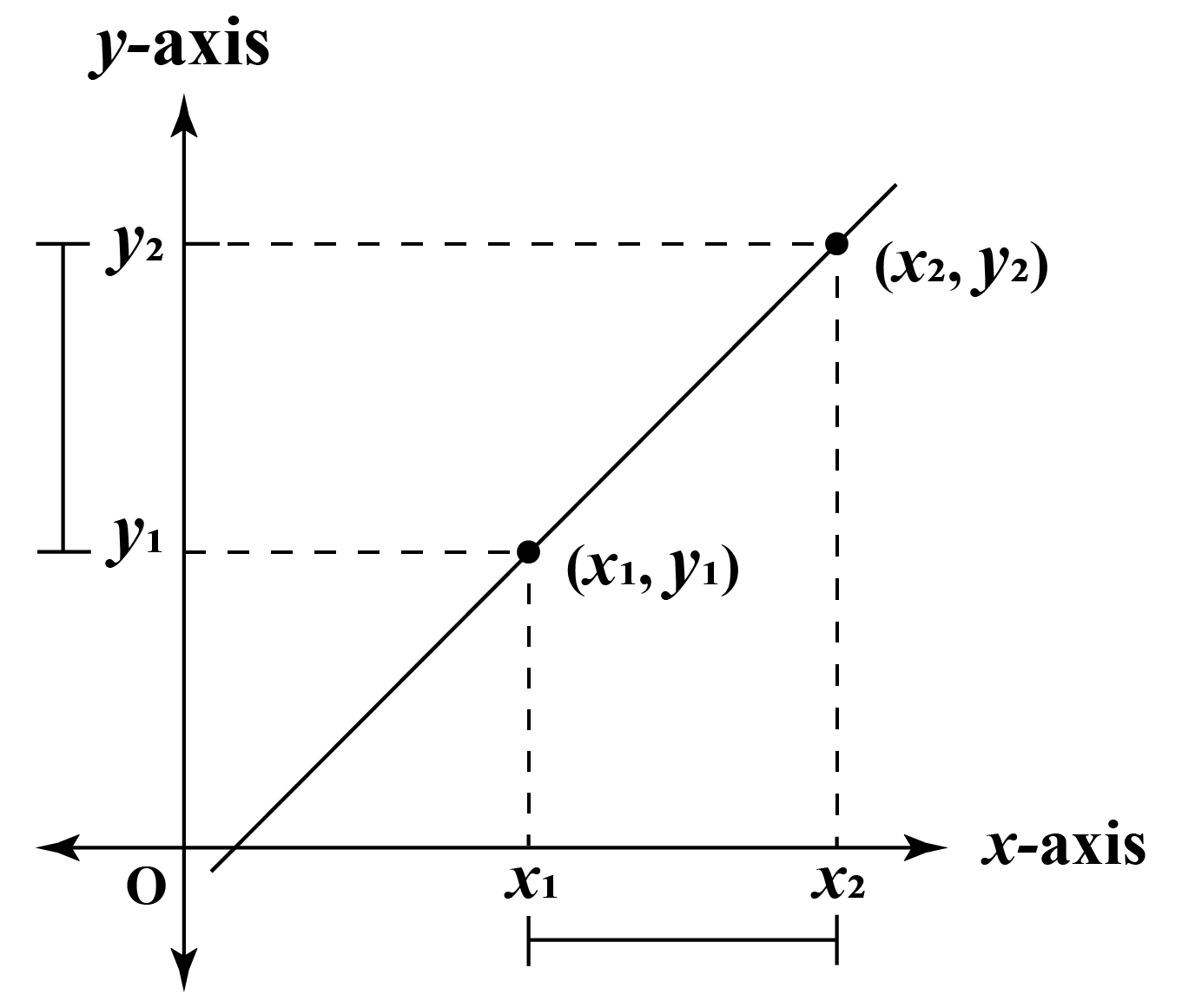
\[ Slope =\frac{y_2 - y_1}{x_2 - x_1} \]
Further, the gradient is a quantity, which helps to understand the variation in one quantity, with respect to another quantity.
For a function f(x) the gradient is calculated from its first derivative, \(\frac{d}{dx}.f(x) \).
Summarizing the above sentences, we have:
\[m = tan\theta = \frac{y_2 - y_1}{x_2 - x_1} = \frac{d}{dx}.f(x) \]
Examples
- The gradient of a line inclined at an angle of \(45^0 \) is \(m = tan 45^0 = 1 \)
- The gradient of a line joining the points(0, 3) and (1, 5) is \(m = \frac{5 - 3}{1 - 0} = \frac{2}{1} = 2 \)
- The gradient of the tangent to the curve \(y = x^2 + x + 3 \) is \(\frac{d}{dx}.y = \frac{d}{dx}.(x^2 + x + 3)\) =\( 2x + 1 + 0 = 2x + 1 \)

- A few quick formulae to calculate the derivatives is as follows: \(\frac{d}{dx}.k(constant) = 0\) ;\(~\frac{d}{dx}.x = 1\) ;\(~ \frac{d}{dx}.x^2 = 2x\);\(~ \frac{d}{dx}.x^3 = 3x^2\);\(~ \frac{d}{dx}.x^n = nx^n-1 \)
- The principal values of \(\theta \) for the Tangent function is as follows: \(tan0^0 = 0\);\(~ tan30^0 = \frac{1}{\sqrt 3}\); \(~tan45^0 = 1\);\(~ tan60^0 =\sqrt3\);\(~ tan90^0 = undefined\)
Properties of Gradient
The following properties of a gradient help to understand the orientation of the line.
- Gradients can have a positive value or a negative value.
- The gradient of a horizontal line is zero and hence the gradient of the x-axis is zero.
- The gradient of a vertical line is undefined and hence the gradient of the y-axis is undefined.
- The gradient of a curve at any point is equal to the gradient of its tangent at that point on the curve.
- The gradient of two parallel lines is equal. \(m_1 = m_2 \)
- The product of the gradients of two perpendicular lines is -1. \(m_1.m_2 = -1 \)
What Is a Directional Derivative?
For a line drawn in an n-dimensional space, the gradient of the line with reference to a specific dimension is called its directional derivative.
The concept of the partial derivative is helpful to find the directional derivative. And it is represented as \(\frac{\delta y}{\delta x} \)
Let us consider an equation y = 5x + 4z + 3xz + 11
This represents the equation of a line in a 3-dimensional array.
Here the partial derivative with reference to x gives the directional derivative in the direction of x-axis. In this expression, z is treated as a constant.
\[\begin{align} \frac{\delta y}{\delta x} &= \frac{\delta}{\delta x}(5x + 4z + 3xz + 11) \\ \frac{\delta y}{\delta x} &= \frac{\delta}{\delta x}(5x) + \frac{\delta}{\delta x}(4z) + \frac{\delta}{\delta x}(3xz)
+ \frac{\delta}{\delta x}(11) \\\frac{\delta y}{\delta x} &= 5(1) + 0 + 3(1)z + 0 \\\frac{\delta y}{\delta x} &=5 + 3z\end{align} \]
\(\therefore \) 5 + 3z is the directional derivative of the equation of the line with respect to the x-axis.

The equation y = mx + c is referred as slope-intercept form. Here, "m" is the slope, and "c" is the y-intercept of the line.
Find the slope and the y-intercept of a line having an equation 4x -9y + 5 = 0.
Solved Examples
| Example 1 |
Suzane is trying to climb up a ladder, which is inclined at an angle of \(60^0 \). What is the gradient of the ladder?
Solution
It is given that the inclination is \(60^0 \).
Gradient of the ladder is its slope (m).
\[\begin{align} m &= tan\theta \\ m &=tan60^0 \\ m &=\sqrt3 \end{align} \]
| \(\therefore \) The gradient of the ladder is \(\sqrt 3 \) |
| Example 2 |
Albert marks two points (4, 3) and (6, 7) on a graph paper and draws a line passing through these points. Find the gradient of the line.
Solution
The given points are \((x_1, y_1) \) = (4, 3) and \((x_2, y_2) \) = (6, 7)
The gradient is the slope(m) of the line joining these points.
\[\begin{align} m &=\frac{y_2 – y_1}{x_2 – x_1} \\ m &=\frac{(7 – 3)}{(6 – 4)} \\m &= \frac{4}{2} \\ m &= 2 \end{align} \]
| \(\therefore \) The gradient is 2 |
| Example 3 |
A line is drawn to touch the curve \(f(x) = x^3 + 2x^2 -5x + 8 \)at the point (1, 6). Find the gradient of this line.
Solution
The equation of the curve is \(f(x) = x^3 + 2x^2 -5x + 8 \)
The line touching this curve is the tangent.
The gradient of the tangent can be found by finding the first derivative of the equation of the curve.
\[\begin{align} \frac{dy}{dx} &= \frac{d}{dx} f(x) \\ \frac{dy}{dx} &= \frac{d}{dx} ( x^3 + 2x^2 -5x + 8) \\ \frac{dy}{dx} &= 3x^2 +4x -5 \end{align} \]
The above derivative is the slope of the tangent of the curve at the referred point.
The slope of the tangent at the point (1,6) is as follows:
\[ \begin{align} m &= 3*(1)^2 + 4(1) – 5 \\ m &= 3 + 4 – 5 \\ m &= 7 – 5 \\ m &= 2 \end{align} \]
| \(\therefore \) The gradient of the tangent is 2 |
| Example 4 |
Cheryl draws two parallel lines and the equation of one line is 2x – y + 5 = 0. Find the gradient of the other line.
Solution
The given equation of the line is 2x – y = 5
Further, the gradient of the two parallel lines is equal.
Let us find the gradient of this line.
\[\begin{align} 2x -y + 5 &= 0 \\ -y &= -2x -5 \\ y &= 2x + 5 \end{align} \]
Comparing this with the slope-intercept form of the equation y = mx + c we have m = 2
The gradient of this line is 2
Hence the required gradient of the parallel line is m = 2
| \(\therefore \) The gradient of the parallel line is 2 |
| Example 5 |
The teacher asks Sam to draw a set of perpendicular lines and to write the slope of one line as 2. Help Sam to find the slope of the other line.
Solution
The slope of the given line is \(m_1 \) = 2
The product of slopes of two perpendicular lines is -1
\[\begin{align} m_1.m_2 &= -1 \\ 2 \times m_2 &= -1 \\ m_2 &= \frac{-1}{2}\end{align} \]
| \(\therefore \) The slope of the line is \(\frac{-1}{2}\) |
Interactive Questions on Gradient
Here are a few activities for you to practice. Select/Type your answer and click the "Check Answer" button to see the result.
Let's Summarize
The mini-lesson targeted the fascinating concept of gradient. The math journey around gradient started with the basics of the gradient and went on to creatively crafting a fresh concept involving formulas and equations. Done in a way that not only it is relatable and easy to grasp, but also will stay with them forever.
About Cuemath
At Cuemath, our team of math experts is dedicated to making learning fun for our favorite readers, the students!
Through an interactive and engaging learning-teaching-learning approach, the teachers explore all angles of a topic.
Be it worksheets, online classes, doubt sessions, or any other form of relation, it’s the logical thinking and smart learning approach that we, at Cuemath, believe in.
Frequently Asked Questions (FAQs)
1. What is the definition of the gradient?
The gradient is the inclination of a line. It is measured in terms of the angle the line makes with the reference x-axis. Also, the two points on the line or the equation of the line are helpful to find the gradient.
\[m = tan\theta = \frac{y_2 - y_1}{x_2 - x_1} = \frac{d}{dx}.f(x) \]
2. What is the gradient of a matrix?
For a matrix containing different functions(equations) as its elements, the derivative of these elements represented in a matrix form is called the gradient of a matrix.
3. What is the purpose of a gradient in mathematics?
In mathematics, the gradient is useful to know the angle between two lines. Generally, one of the lines is considered to be the horizontal line parallel to the x-axis or the x-axis and the angle it makes with the other line is referred to as the gradient of that line.
If the angle between the lines is \(\theta \) then the gradient \(m = tan\theta \).