Permutation and Combination

Permutation and combination form the principles of counting and they are applied in various situations. A permutation is a count of the different arrangements which can be made from the given set of things. In permutation the details matter, as the order or sequence is important. Writing the names of three countries {USA, Brazil, Australia} or {Australia, USA, Brazil) or { Brazil, Australia, USA} is different and this sequence in which the names of the countries are written is important. In combinations, the name of three countries is just a single group, and the sequence or order does not matter. Let us learn more about permutation and combination in the below content.

Permutation and combination are the methods employed in counting how many outcomes are possible in various situations. Permutations are understood as arrangements and combinations are understood as selections. As per the fundamental principle of counting, there are the sum rules and the product rules to employ counting easily.

Suppose there are 14 boys and 9 girls. If a boy or a girl has to be selected to be the monitor of the class, the teacher can select 1 out of 14 boys or 1 out of 9 girls. She can do it in 14 + 9 = 23 ways(using the sum rule of counting). Let us look at another scenario. Suppose Sam usually takes one main course and a drink. Today he has the choice of burger, pizza, hot dog, watermelon juice, and orange juice. What are all the possible combinations that he can try? There are 3 snack choices and 2 drink choices. We multiply to find the combinations. 3 × 2 = 6. Thus Sam can try 6 combinations using the product rule of counting. This can be shown using tree diagrams as illustrated below.

In order to understand permutation and combination, the concept of factorials has to be recalled. The product of the first n natural numbers is n! The number of ways of arranging n unlike objects is n!.

Permutations

A permutation is an arrangement in a definite order of a number of objects taken some or all at a time. Let us take 10 numbers: 0, 1, 2, 3, 4, 5, 6, 7, 8 and 9. The number of different 4-digit-PIN which can be formed using these 10 numbers is 5040. P(10,4) = 5040. This is a simple example of permutations. The permutations of 4 numbers taken from 10 numbers equal to the factorial of 10 divided by the factorial of the difference of 10 and 4. The permutations is easily calculated using \(^nP_r = \frac{n!}{(n - r)!}\).

Combinations

A combination is all about grouping. The number of different groups which can be formed from the available things can be calculated using combinations. Let us try to understand this with a simple example. A team of 2 is formed from 5 students(William, James, Noah, Logan, and Oliver). This the combination of 'r' persons from the available 'n' persons is given as \(^nC_r = \frac{n!}{r!.(n - r)!}\) The combinations can happen in the following 10 ways by which the team of 2 could be formed.

• William James
• William Noah
• William Logan
• William Oliver
• James Noah
• James Logan
• James Oliver
• Logan Noah
• Logan Oliver
• Oliver Noah

This is a simple example of combinations. C(5,2) = 10.

Permutation and combination formulas are helpful to find the permutation and combination of r objects taken from n objects. The concept of permutations is used to find the different arrangements and the concept of combinations is used to find the different groups. For the given values of n and r, the permutations are always greater than the combinations.

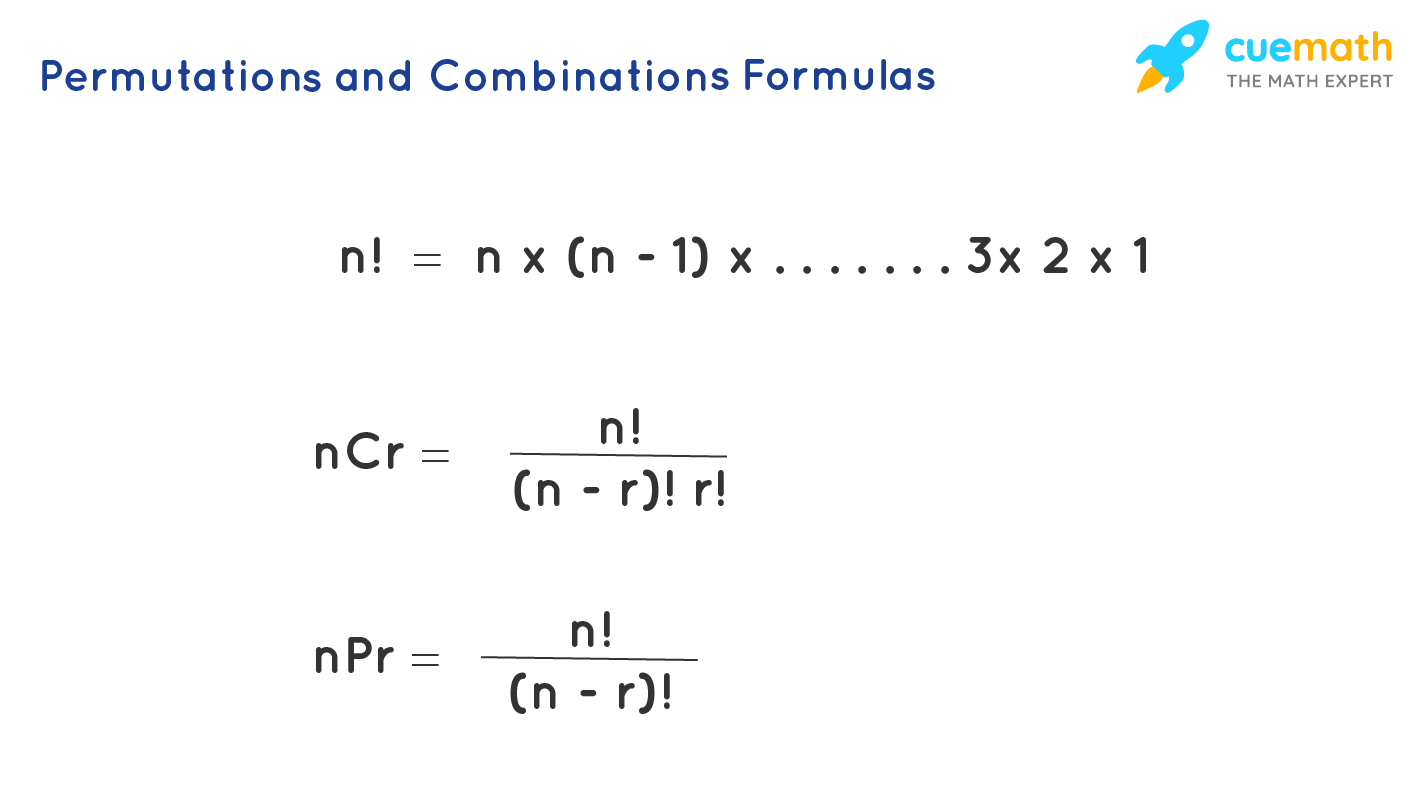
Permutation And Combination Formulas

The counting situation is analyzed to determine whether to employ permutations or combinations. Accordingly, the permutation and combination formulas are applied.

Formula 1: Factorial of a natural number n.

n! = 1 × 2 × 3 × 4 × .......× n

Formula 2: The number of distinct permutations of r objects which can me made from n distinct objects is

\(^nP_r = \dfrac{n!}{(n - r)!}\), where 0 ≤ r ≤ n.

Formula 3: The number of permutations of n different things, taken r at a time, where repetition is allowed, is n^r.

Formula 4: The number of permutations of n objects taken all at a time, where p\(_1\) objects are of the first kind, p\(_2\)

objects are of the second kind, ..., p\(_k\) objects are of the k^th kind and the rest if any, and if all are different is

\(\dfrac{n!}{p_1! p_2!....p_k!}\)

Formula 5: The number of combinations of n different objects taken r at a time is given by

\(^nC_r = \dfrac{n!}{r!.(n - r)!}\), where 0 ≤ r ≤ n.

This formula is sometimes also called as ncr formula.

Formula 6: The relationship between permutation and combination for r things taken from n things.

\(^nP_r = r! \times ^nC_r \)

The permutations formula: The total number of permutations of a set of n objects taken r at a time P(n,r) = n!/(n-r)!, where [n>= r]

The combination formula: The total number of combinations of a set of n objects taken r at a time C(n,r) = n!/[r !(n-r)!], where [n>= r]

Let us look into the derivation below for a deeper understanding using our knowledge in practical counting situations.

Derivation of Permutations Formula

Since a permutation involves selecting r distinct items without replacement from n items and order is important, by the fundamental counting principle, we have
P (n, r) = n . (n-1) . (n-2) . (n-3)…… (n-(r-1)) ways.
This can be written as:
P (n, r) = n.(n-1).(n-2). (n-3) …. (n-r+1)---------------> (1)
Multiplying and Dividing (1) by (n-r) (n-r-1) (n-r-2)........... 3. 2. 1, we get
P (n, r) =

\(\dfrac{n.(n-1).(n-2).…. (n-r+1)[(n-r) (n-r-1) (n-r-2)... 3. 2. 1]}{[(n-r) (n-r-1) (n-r-2)....3. 2. 1]}\)
P (n, r) = \(\dfrac{n!}{(n-r)!}\)

Derivation of Combinations Formula

Since combinations involve choosing r objects out of n objects where the order doesn't matter, we can determine that:

C(n,r) = the number of permutations /number of ways to arrange r objects. [Since by the fundamental counting principle, we know that the number of ways to arrange r objects in r ways = r!]

C(n,r) = P (n, r)/ r!

C(n,r) = \(\dfrac{\dfrac{n!}{(n-r)!}}{r!}\)

Thus we derive C(n,r) =\(\dfrac{n!}{r!.(n - r)!}\)

The difference between the permutation and combination can be understood through the following points.

• Permutations are used when order/sequence of arrangement is needed. Combinations are used when only the number of possible groups are to be found, and the order/sequence of arrangements is not needed.
• Permutations are used for things of a different kind. Combinations are used for things of a similar kind.
• The permutation of two things from three given things a, b, c is ab, ba, bc, cb, ac, ca. The combination of two things from three given things a, b, c is ab, bc, ca
• For different possible arrangement of things nPr=n!/(n-r)!. For different possible selection of things nCr =n!/r!(n-r)!
• For a given set of n and r values, the permutation answer is larger than the combination answer.

☛ Also Check:

• Examples - Permutations as Arrangements
• Factorial Calculator
• Introduction to Combinations
• Probability
• Probability and Statistics

Let us see the application of the permutation and combination formulas in the following solved examples.

What Are Permutation and Combination?

Permutation and combination are the principles of counting used in various situations. Permutations are the form of counting used in the arrangement of r distinct objects out of n distinct objects. Combinations are the form of counting used in the selection of r different objects taken from n different objects.

What Is the Difference Between Permutation and Combination?

The permutation is the number of different arrangements that can be made by picking r number of things from the available n things. The combination is the number of different groups of r objects each, which can be formed from the available n objects. Further permutations are used for creating passwords, for creating different words from the set of alphabets, and for different seating arrangements. And the combination is used for the selection of people, formation of teams or committees, a grouping of objects.

What Is the Formula for Permutation and Combination?

The formula for permutations is \(^nP_r =\frac{n!}{(n - r)!} \) and the formula for combinations is \(^nC_r =\frac{n!}{r!.(n - r)!} \). Before working and applying these formulas we need to understand n!. It is called n factorial and is the product of the consecutive numbers from 1 to n. Also we need to know that ⁿP₀ = 1, ⁿP₁ = n, ⁿP_n = n!, ⁿC₀ = 1, ⁿC₁= n, ⁿC_n = 1.

What Is the Relationship Between Permutation and Combination?

The formula for permutations is \(^nP_r =\frac{n!}{(n - r)!} \) and the formula for combinations is \(^nC_r =\frac{n!}{r!.(n - r)!} \). Combining both the formulas we can write \(^nC_r =\frac{^nP_r}{r!} \), or we have \(^nP_r =r!× ^nC_r \)

How Do You Find Factorial of a Number?

The factorial of a number is obtained by taking the product of all the numbers from 1 to n in sequence. Here we have n! = 1 × 2 × 3 × 4 × 5 × .......n. As an example let us find the value of 5! = 1 × 2 × 3 × 4 × 5 = 120. The formula of n! is used in the formulas of permutation and combination.

What Are the Examples of Permutation and Combination?

The examples of permutations are for different arrangements such as seating arrangements, formation of different passwords from the given set of digits and alphabets, arrangement of books on a shelf, flower arrangements. And the examples of combinations are the formation of teams from the set of eligible players, the formation of committees, picking a smaller group from the available large set of elements.

Which of the Two of Permutation and Combination Is of Greater Value?

The formulas of permutation and combination is ⁿP_r = n!/(n - r)! and ⁿC_r = n!/r!(n - r)!. For the given value of n and r the permutations are greater than the combinations since the number of arrangement are always more than the number of groups which can be formed. Mathematically observing n! is the same in both the formulas, but the denominator in combinations is larger, hence combination is lesser than permutations.

What Are the Areas in Mathematics Where Permutation and Combination Are Used?

The concepts of permutation and combination are prominently used in probability, sets and relations, functions. The different sequences or arrangements can be found with the help of permutations, and the different groups can be found with the help of combinations.

What is 0!?

The value of 0! = 1 is used very often in formulas of permutation and combination. Let us understand this with an example \(^nP_n =\frac{n!}{(n - n)!} =\frac{n!}{0!} =\frac{n!}{1}=n!\) , and \(^nC_n =\frac{n!}{n!.(n - n)!} =\frac{n!}{n!.0!} =\frac{n!}{n!.1}=\frac{n!}{n!} = 1\).

What Is The Formula For Permutation And Combination?

Permutation and combination are calculated using different formulas. Permutations are arranging r objects out of n objects. The permutations formula is given by \(^nP_r = \dfrac{n!}{(n - r)!}\).

Combinations are selecting r objects out of n objects. The combinations formula is given by \(^nC_r = \dfrac{n!}{r!.(n - r)!}\)

What Is The Relation Between Permutation and Combination?

The relation between permutation and combination is given by the formula: \(^nC_r = \dfrac{^nP_r}{r !}\).

How Are Permutation And Combination Formulas Used in Real-life?

For arranging the numbers, allocating the PIN codes, setting up passwords, and so on we use permutations formulas. For selecting the team members, choosing food menu, drawing lottery, and so on. we use combinations formulas.

What Is The Method of Calculating Permutation and Combination?

Identify whether the problem involves permutations or combinations. Understand if we are going to arrange r objects out of n objects or select r objects out of n objects. Determine the permutation and combination formula to be used. The permutations formula is \(^nP_r = \dfrac{n!}{(n - r)!}\) and the combinations formula is \(^nC_r = \dfrac{n!}{r!.(n - r)!}\). Apply n and r as required in the formula and arrive at the desired result.