LearnPracticeDownload
 

Prime Factorization

Prime factorization is a way of expressing a number as a product of its prime factors. A prime number is a number that has exactly two factors, 1 and the number itself. Let’s take an example of the number 30. We know that 30 is 5 × 6, but 6 is not a prime number. The number 6 is expressed as 2 × 3 since 3 and 2 are prime numbers. Therefore, the prime factorization of 30 is 2 × 3 × 5.

In this lesson, you will be learning prime factorization to solve various mathematical problems followed by solved examples and practice questions.

1. What is Prime Factorization?
2. Prime Factorization of a Number
3. What are Factors and Prime Factors?
4. Methods to Find the Prime Factorization
5. Applications of Prime Factorization
6. FAQs on Prime Factorization

What is Prime Factorization?

The process of writing a number as the product of prime numbers is prime factorization. Prime numbers are the numbers that have only two factors 1 and the number itself like 2, 3, 5, 7, 11, 13, 17, 19, and so on.

Prime factorization of any number means to represent that number as a product of prime numbers. For example, prime factorization of 40 is the representation of 40 as a product of prime numbers and can be done in the following way: 

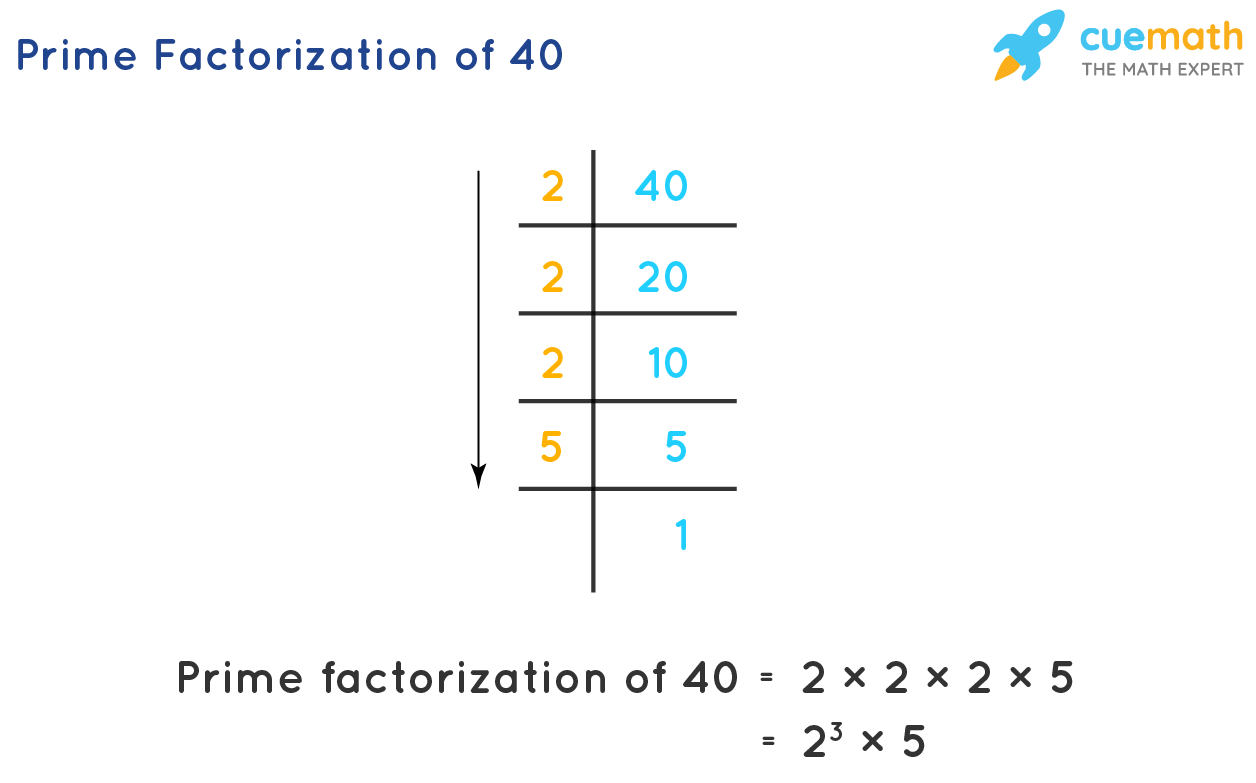
Prime Factorization of 40

 

 

 

 

 

 

 

Prime Factorization of a Number

Let us see the prime factorization chart of a few more numbers in the table given below:

Numbers Prime Factorization
36  22 × 32
24  23 × 3
60  22 × 3 × 5
18  2 × 32
72  23 × 32
45  32 × 5
40  23 × 5
50  2 × 52
48  24 × 3
30  2 × 3 × 5
42  2 × 3 × 7

What are Factors and Prime Factors?

Prime factorization is similar to factoring a number and considering only the prime numbers (2, 3, 5, 7, 11, 13, 17, 19, and so on) among all the factors. The factors are the numbers that divide the original number completely and can't be split into more factors are known as the prime factors.

Factors of a number are the numbers that are multiplied to get the original number. For example; 4 and 5 are the factors of 20, i.e. 4 × 5 = 20, whereas prime factors of a number are the prime numbers that are multiplied to get the original number. For example: 2, 2, and 5 are the prime factors of 20, i.e. 2 × 2 × 5 = 20.

Methods to find Prime Factorization

There are various methods to find the prime factorization of a number. The most common methods used to find the prime factorization are given below:

  • Prime factorization using factor tree method
  • Division method of prime factorization

Prime Factorization using Factor Tree Method

In the factor tree method, the factors of a number are found and then those numbers are further factorized until we reach the prime numbers. To evaluate the prime factorization of a number using the factor tree method, follow the steps given below:

  • Step 1: Consider the number as the root of the tree that is at the top of the factor tree.
  • Step 2: Then write down the corresponding pair of factors as the branches of the tree.
  • Step 3: Factorize the composite factors that are found in step 2, and write down the pair of factors as the next branches of the tree.
  • Step 4: Repeat step 3, until we get the prime factors of all the composite factors.

Example: Follow the diagram given below to understand the concept and find the prime factorization of 850.

Prime Factorization by Factor Tree Method

Division Method of Prime Factorization

The division method can also be used to find the prime factors of a large number by dividing the number by prime numbers. Follow the steps given below to find the prime factors of a number by using the division method:

  • Step 1: Divide the number by the smallest prime number such that the smallest prime number should divide the number completely.
  • Step 2: Again, divide the quotient of step 1 by the smallest prime number.
  • Step 3: Repeat step 2, until the quotient becomes 1.
  • Step 4: Finally, multiply all the prime factors that are the divisors of the division.

Applications of Prime Factorization

There is a wide range of properties of prime factorization. The two most important applications of the prime factorization are given below.

  • Cryptography and Prime Factorization
  • HCF and LCM Using Prime Factorization

Cryptography and Prime Factorization

Cryptography is a method of protecting information and communicating cryptography through the use of codes. Prime factorization plays an important role for the coders who want to create a unique code using numbers that is not too heavy for computers to store or process quickly.

HCF and LCM Using Prime Factorization

To find the HCF and LCM of two numbers, we use the prime factorization method. For this, we first find the prime factorization of both the numbers. Next, we consider the following:

  • HCF is the product of the smallest power of each common prime factor.
  • LCM is the product of the greatest power of each common prime factor.

Example: What is the HCF and LCM of 850 and 680?

Solution: We first find the prime factorizations of both the numbers. The prime factorization of 850 is shown below:

Prime Factorization by Factor Tree Method

The prime factorization of 680 is shown below:

Prime Factorization of 680

Thus: 850 = 21 × 52 × 171 , 680 = 23 × 51 × 171

HCF is the product of the smallest power of each common prime factor. Hence, HCF of (850, 680) = 21 × 51 × 171 = 170. LCM is the product of the greatest power of each common prime factor. Hence, LCM of (850, 680) = 23 × 52 × 171 = 3400. Thus,  HCF of (850, 680) = 17,   LCM of (850, 680) = 3400

Related Topics:

Prime Factorization Solved Examples 

  1. Example 1: Can you help Charlize to express 1080 as the product of prime factors? Also, can you tell if this factorization is unique?

    Solution:

    We will find the prime factorization of 1080:

    Prime Factorization of 1080

    Thus, 1080 = 23 × 33 × 51

    Therefore, the prime factorization of 1080 is 23 × 33 × 51

  2. Example 2: Jenifer was given a task by her teacher to find the lowest common multiple of 48 and 72 using prime factorization. Can you help her?

    Solution:

    We will find the prime factorizations of 48 and 72. The prime factorization of 48 is shown below:

    Prime Factorization of 48

    The prime factorization of 72 is shown below:

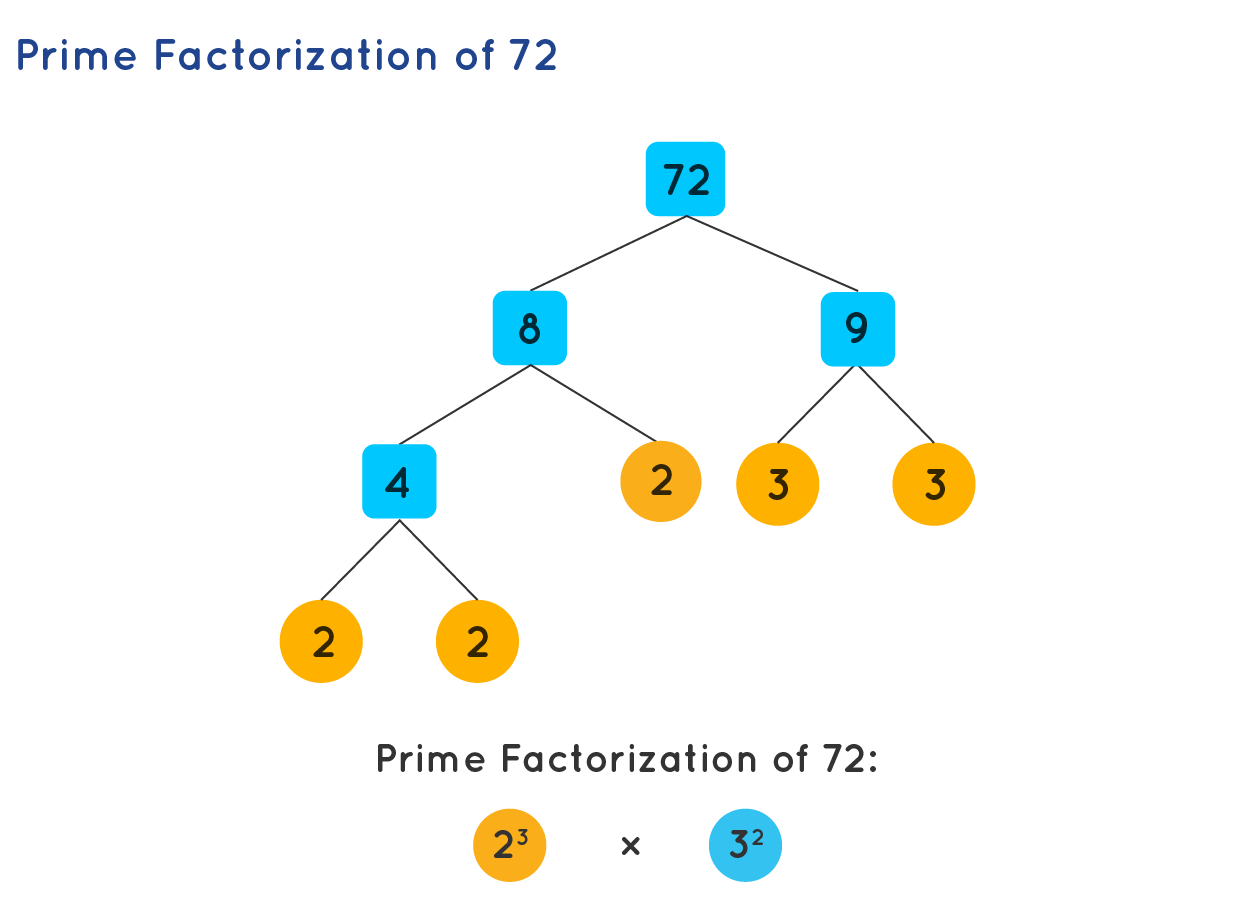
    Prime Factorization of 72

    The LCM or lowest common multiple of any 2 numbers is the product of the greatest power of the common prime factors. Hence,  LCM (48, 72) = 24 × 32 = 144

    Therefore, LCM (48, 72) = 24 × 32 = 144

  3. Example 3: Jane has to prove that the prime factorization of 40 will always remain the same. She is confused, help her prove it.

    Solution:

    Jane can use the division method and factor tree method to prove that the prime factorization of 40 will always remain the same. Jane knows that 40 can be factored as 5 and 8. The composite number 8 can further be broken down as a product of 2 and 4. The number 5 is a prime number already. Hence, she will show the division method and factor tree method in the following way: 

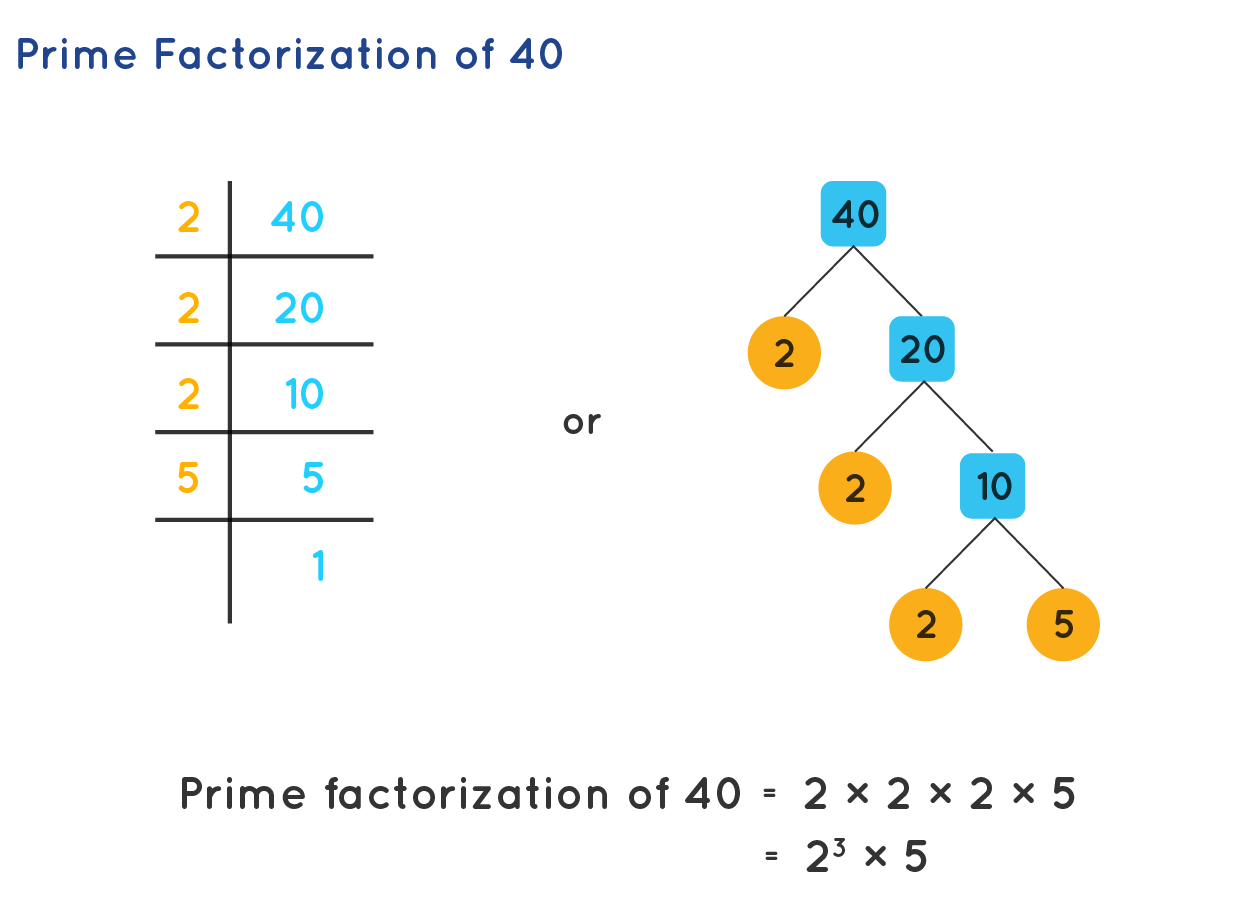
    Prime Factorization of 40

    Therefore, this shows that by any method of factorization, the prime factorization remains the same. The prime factorization for a number is unique.

     
Show Solution >

go to slidego to slidego to slide

 

 

 

FAQs on Prime Factorization 

What is Prime Factorization in Math?

Prime factorization of any number means to represent that number as a product of prime numbers. A prime number is a number that has exactly two factors 1 and the number itself.

How to Find Prime Factorization?

Prime factorization of any number can be calculated out by following two methods:

  • Method 1: Division method.
  • Method 2: Factor tree method.

What is the Prime Factorization of 72, 36, and 45?

Prime factorization is the way of writing a number as the multiple of their prime factors. The first few prime numbers are 2, 3, 5, 7, 11, 13, 17, 19, and so on. The prime factorization of 72, 36, and 45 are shown below.

  • Prime factorization of 72 = 23 × 32
  • Prime factorization of 36 = 22 × 32
  • Prime factorization of 45 = 32 × 5

How to Find LCM using Prime Factorization?

The abbreviation LCM stands for "Least Common Multiple". The least common multiple of a number is the smallest number that is the product of two or more numbers. LCM of two numbers can be found out by first finding out the prime factors of the numbers. Then the LCM is the product of the greatest power of each common prime factor.

How to Find HCF using Prime Factorization?

The abbreviation HCF stands for "Highest Common Factor". The Highest Common Factor (HCF) of two numbers is the highest possible number which divides both the numbers exactly. HCF of two numbers can be found out by first finding out the prime factors of the numbers. Then the HCF is the highest common factor from the prime factors of the two numbers.

Where is Prime Factorization Useful?

Prime factorization is useful in finding HCF and LCF of numbers. It is widely used in cryptography as the study of secret codes is known as cryptography. Prime numbers are used to form or decode those codes.

What is the Prime Factorization of 24?

The number 24 can be written as 4 × 6. Now the composite numbers 4 and 6 are also factorized as 4 = 2 × 2 and 6 = 2 × 3. Therefore, the prime factorization of 24 is 24 = 2 × 2 × 2 × 3 = 23 × 31

Download FREE Study Materials
Download Arithmetic Integers Worksheets
Pick your preferred
day & time: