Synthetic Division
In algebra, the synthetic division is one of the methods used to manually perform the Euclidean division of polynomials. The division of polynomials can also be done using the long division method. But, in comparison to the long division method of polynomials, the synthetic division requires lesser writing and fewer calculations. That means the synthetic division is the shorter method of the traditional long-division of a polynomial for the special cases when dividing by a linear factor.
Let us understand the method to perform the synthetic division of polynomials in detail using solved examples.
| 1. | What is Synthetic Division? |
| 2. | Synthetic Division Method |
| 3. | How to do Synthetic Division? |
| 4. | FAQs on Synthetic Division |
What is Synthetic Division?
Synthetic division is a method used to perform the division operation on polynomials when the divisor is a linear factor. One of the advantages of using this method over the traditional long method is that the synthetic division allows one to calculate without writing variables while performing the polynomial division, which also makes it an easier method in comparison to the long division.
We can represent the division of two polynomials in the form:
p(x)/q(x) = Q(x) + R/(q(x))
where,
- p(x) is the dividend
- q(x) is the linear divisor
- Q(x) is quotient
- R is remainder
Synthetic Division of Polynomials Definition
When we divide a polynomial p(x) by a linear factor (x - a) (which is a polynomial of degree 1), Q(x) is the quotient polynomial and R is the remainder.
p(x)/q(x) = p(x)/(x- a) = Quotient + (Remainder/(x - a))
p(x)/(x - a) = Q(x) + (R/(x - a))
The coefficients of p(x) are taken and divided by the zero of the linear factor.
We use synthetic division in the context of the evaluation of the polynomials by the remainder theorem, wherein we evaluate the value of p(x) at "a" while dividing (p(x)/(x - a)). That is, to find if "a" is the factor of the polynomial p(x), use the synthetic division to find the remainder quickly. Let us understand this better using the example given below.
Synthetic Division Example
Richard sells apples. The previous day, his profits were x, and today, his profits are ((x × x) - 2). If the number of apples he sold was (x + 2), what was the profit made per apple? We obtain the solution by modelling the equation as (x2 + x - 2) ÷ (x + 2).
Step 1: Write the coefficients of the dividend inside the box and zero of x + 2 as the divisor.
Step 2: Bring down the leading coefficient 1 to the bottom row.
Step 3: Multiply -2 by 1 and write the product -2 in the middle row.
Step 4: Add 1 and -2 in the second column and write the sum -1 in the bottom row.
Step 5: Now, multiply -2 by -1 (obtained in step 4) and write product 2 below -2.
Step 6: Add -2 and 2 in the third column and write the sum 0 in the bottom row.
Step 7: The bottom row gives the coefficient of the quotient. The degree of the quotient is one less than that of the dividend. So, the final answer is x - 1 + 0/(x + 2) = x - 1.
Please note that the last box in the bottom row gives the remainder.

The profit per apple is given by (x - 1).
Synthetic Division vs Long Division
Let us see how long division differs from the synthetic division of polynomials by comparing both methods. In the example given below, we will perform the division of the polynomial 4x2 - 5x - 21 by a linear polynomial x - 3.
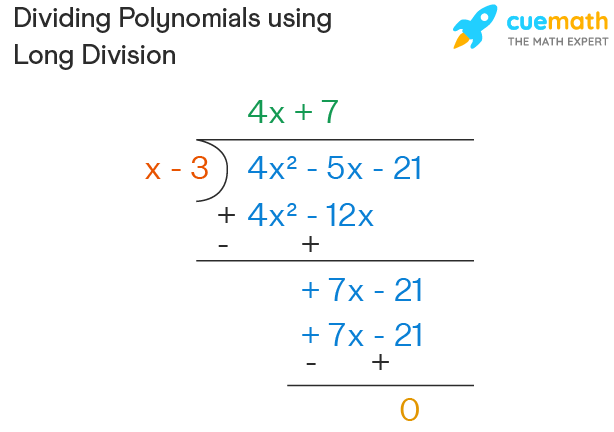
In the example given below, another polynomial 2x2 + 3x - 1 is divided by a linear polynomial x + 1. When a polynomial P(x) is to be divided by a linear factor, we write the coefficients alone, bring down the first coefficient, multiply, and add. Repeat the multiplication and addition until we reach the end term of the polynomial.

Using synthetic division, we can perform complex division and obtain the solutions easily.
Synthetic Division Method
The following are the steps while performing synthetic division and finding the quotient and the remainder. We will take the following expression as a reference to understand it better: (2x3 - 3x2 + 4x + 5)/(x + 2)
- Check whether the polynomial is in the standard form.
- Write the coefficients in the dividend's place and write the zero of the linear factor in the divisor's place.
- Bring the first coefficient down.

- Multiply it with the divisor and write it below the next coefficient.
- Add them and write the value below.

- Repeat the previous 2 steps until you reach the last term.

- Separate the last term thus obtained which is the remainder.
- Now group the coefficients with the variables to get the quotient.
Therefore, the result obtained after synthetic division of (2x3 - 3x2 + 4x + 5)/(x + 2) is 2x2 - 7x + 18 and remainder is -31
How to do Synthetic Division?
Synthetic division of polynomials uses numbers for calculation and avoids the usage of variables. In the place of division, we multiply, and in the place of subtraction, we add.
- Write the coefficients of the dividend and use the zero of the linear factor in the divisor's place.
- Bring the first coefficient down and multiply it with the divisor.
- Write the product below the 2nd coefficient and add the column.
- Repeat until the last coefficient. The last number is taken as the remainder.
- Take the coefficients and write the quotient.
- Note that the resultant polynomial is of one order less than the dividend polynomial.
Example:
1) Consider this division: (x3 - 2x3 - 8x - 35)/(x - 5). The polynomial is of order 3. The divisor is a linear factor. Let's use synthetic division to find the quotient. Thus, the quotient is one order less than the given polynomial. It is x2 + 3x + 7 and the remainder is 0. (x3 - 2x3 - 8x - 35)/(x - 5) = x2 + 3x + 7.

Tips and Tricks on Synthetic Division:
- Write down the coefficients and divide them using the zero of the linear factor to obtain the quotient and the remainder. (P(x)/(x - a) = Q(x) + (R/(x - a))
- When we do synthetic division by (bx + a), we should get (Q(x)/b) as the quotient.
- Perform synthetic division only when the divisor is a linear factor.
- Perform multiplication and addition in the place of division and subtraction that is used in the long division method.
☛Related Articles:
Synthetic Division Examples
-
Example 1: The distance covered by Steve in his car is given by the expression 9a2 - 39a - 30. The time taken by him to cover this distance is given by the expression (a - 5). Find the speed of the car.
Solution:
Speed is given as the ratio of the distance to the time.
Speed = (9a2 - 39a - 30)/(a - 5)

Speed = (9a + 6)
Answer: Speed is given by the expression 9a + 6.
-
Example 2: The volume of Sara's storage box is 8x3 + 12x2 - 2x - 3. She knows that the area of the box is 4x2 - 1. What could be the height of the box?
Solution:
Area (A) = length(l) × breadth(b)
Given A = 4x2 - 1. This is of the form a2 - b2 = (a + b)(a - b)
This can be expressed as, A = (2x + 1)(2x - 1)
V = l × b × h = A × h
h = (V/A) = (8x3 + 12x2 - 2x - 3)/[(2x + 1)(2x - 1)]
Let's solve this by the synthetic division twice.

Answer: Height of the box = 2x + 3.
-
Example 3: Perform synthetic division to solve the following expression: (6x2 + 7x - 20)/(2x + 5).
Solution:
Let us have a look at the steps shown below,

Answer: Quotient for the given division of polynomials = 3x - 4.

FAQs on Synthetic Division
What is Synthetic Division?
When a polynomial has to be divided by a linear factor, the synthetic division is the shortest method. It is an alternative to the traditional long division method used to solve the polynomial division.
How do you Divide Polynomials by Synthetic Division?
We can perform synthetic division using some general steps. Take the coefficients alone, bring the first down, multiply with the zero of the linear factor, and add with the next coefficient and repeat until the end.
What is the Importance of the Synthetic Division?
Synthetic division can be generalized and expanded to the division of any polynomial with any polynomial. It is an easier method in comparison to the long division method for performing division on polynomials with the linear divisor.
What are the Advantages of the Synthetic Division of Polynomials?
This method uses fewer calculations and is quicker than long division. It takes comparatively lesser space while computing the steps involved in the polynomial division.
What are the Disadvantages of Synthetic Division?
The synthetic division can be used only when the divisor is a linear polynomial. We have to follow the long division method for the other cases.
What are the Main Uses of Synthetic Division of Polynomials?
Synthetic division of polynomials helps in finding the zeros of the polynomial. It also reduces the complexity of the expression while dividing the polynomials by a linear factor.
What is the Quotient in Synthetic Division?
In synthetic division, the polynomial obtained is one power lesser than the power of the dividend polynomial. The result obtained can be arranged to form the quotient of the polynomial division.
visual curriculum