Long Division

Long Division is a method for dividing large numbers, which breaks the division problem into multiple steps following a sequence. Just like the regular division problems, the dividend is divided by the divisor which gives a result known as the quotient, and sometimes it gives a remainder too. Let us learn how to divide using the long division method, along with long division examples with answers, which include the long division steps in this article.

1.	What is Long Division Method?
2.	Parts of Long Division
3.	How to do Long Division?
4.	FAQs on Long Division

Long division is a method for dividing large numbers into steps or parts, breaking the division problem into a sequence of easier steps. It is the most common method used to solve problems based on division. Observe the following long division method to see how to divide step by step and check the divisor, the dividend, the quotient, and the remainder.

The above example also showed us how to do 2 digit by 1 digit division.

While performing long division, we need to know the important parts of long division. The basic parts of long division can be listed as follows:

• Dividend
• Divisor
• Quotient
• Remainder

The following table describes the parts of long division with reference to the example shown above.

Dividend	The number which has to be divided.	75
Divisor	The number which divides the dividend.	4
Quotient	The result of division.	18
Remainder	The leftover part or the number left after the division which cannot be divided further.	3

Division is one of the four basic mathematical operations, the other three being addition, subtraction, and multiplication. In arithmetic, long division is a standard division algorithm for dividing large numbers, breaking down a division problem into a series of easier steps. Let us learn about the steps that are followed in long division.

Long Division Steps

In order to perform division, we need to understand a few steps. The divisor is separated from the dividend by a right parenthesis 〈)〉 or vertical bar 〈|〉 and the dividend is separated from the quotient by a vinculum (an overbar). Now, let us follow the long division steps given below to understand the process.

• Step 1: Take the first digit of the dividend from the left. Check if this digit is greater than or equal to the divisor.
• Step 2: Then divide it by the divisor and write the answer on top as the quotient.
• Step 3: Subtract the result from the digit and write the difference below.
• Step 4: Bring down the next digit of the dividend (if present).
• Step 5: Repeat the same process.

Let us have a look at the examples given below for a better understanding of the concept. While performing long division, we may come across problems when there is no remainder, while some questions have remainders. So, first, let us learn division in which we get remainders.

Division with Remainders

Case 1: When the first digit of the dividend is equal to or greater than the divisor.

Example: Divide 435 ÷ 4

Solution: The steps of this long division are given below:

• Step 1: Here, the first digit of the dividend is 4 and it is equal to the divisor. So, 4 ÷ 4 = 1. So, 1 is written on top as the first digit of the quotient.
• Step 2: Subtract 4 - 4 = 0. Bring the second digit of the dividend down and place it beside 0.
• Step 3: Now, 3 < 4. Hence, we write 0 as the quotient and bring down the next digit of the dividend and place it beside 3.
• Step 4: So, we have 35 as the new dividend. We can see that 35 > 4 but 35 is not divisible by 4, so we look for the number just less than 35 in the table of 4. We know that 4 × 8 = 32 which is less than 35 so, we go for it.
• Step 5: Write 8 in the quotient. Subtract: 35 - 32 = 3.
• Step 6: Now, 3 < 4. Thus, 3 is the remainder and 108 is the quotient.

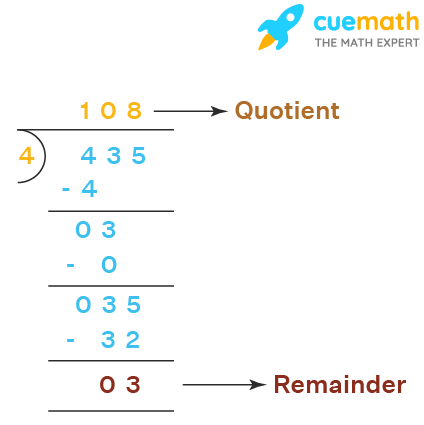
Case 2: When the first digit of the dividend is less than the divisor.

Example: Divide 735 ÷ 9

Solution: Let us divide this using the following steps.

• Step 1: Since the first digit of the dividend is less than the divisor, put zero as the quotient and bring down the next digit of the dividend. Now consider the first 2 digits to proceed with the division.
• Step 2: 73 is not divisible by 9 but we know that 9 × 8 = 72 so, we go for it.
• Step 3: Write 8 in the quotient and subtract 73 - 72 = 1.
• Step 4: Bring down 5. The number to be considered now is 15.
• Step 5: Since 15 is not divisible by 9 but we know that 9 × 1 = 9, so, we take 9.
• Step 6: Subtract: 15 - 9 = 6. Write 1 in the quotient.
• Step 7: Now, 6 < 9. Thus, remainder = 6 and quotient = 81.

Case 3: This is a case of long division without a remainder.

Division without Remainder

Example: Divide 900 ÷ 5

Solution: Let us see how to divide step by step.

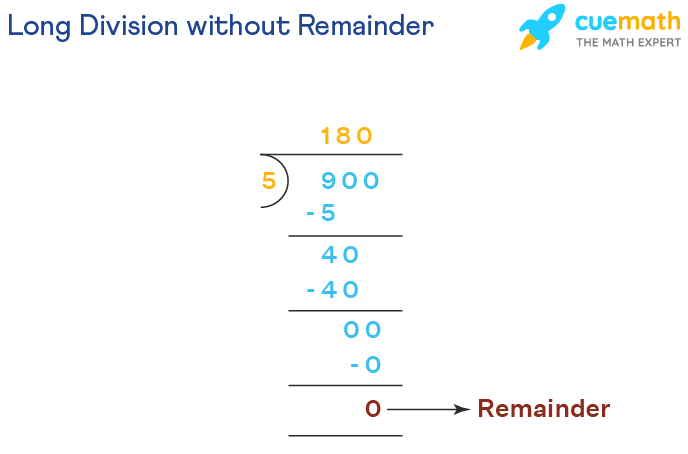
• Step 1: We will consider the first digit of the dividend and divide it by 5. Here it will be 9 ÷ 5.
• Step 2: Now, 9 is not divisible by 5 but 5 × 1 = 5, so, write 1 as the first digit in the quotient.
• Step 3: Write 5 below 9 and subtract 9 - 5 = 4.
• Step 4: Since 4 < 5, we will bring down 0 from the dividend to make it 40.
• Step 5: 40 is divisible by 5 and we know that 5 × 8 = 40, so, write 8 in the quotient.
• Step 6: Write 40 below 40 and subtract 40 - 40 = 0.
• Step 7: Bring down the next 0 from the dividend. Since 5 × 0 = 0, we write 0 as the remaining quotient.
• Step 9: Therefore, the quotient = 180 and there is no remainder left after the division, that is, remainder = 0.

Long division problems also include problems related to long division by a 2 digit number, long division polynomials and long division with decimals. Let us get an an idea about these in the following sections.

Long Division by a 2 Digit Number

Long division by a 2 digit number means dividing a number by a 2-digit number. For long division by a 2 digit number, we consider both the digits of the divisor and check for the divisibility of the first two digits of the dividend.

For example, if we need to divide 7248 by 24, we can do it using the long division steps. Let us see how to divide step by step.

• Step 1: Since it is a long division by a 2 digit number, we will check for the divisibility of the first two digits of the dividend. The first 2 digits of the dividend are 72 and it is greater than the divisor, so, we will proceed with the division.
• Step 2: Using the multiplication table of 24, we know that 24 × 3 = 72. So we write 3 in the quotient and 72 below the dividend to subtract these. Subtract 72 - 72 = 0.
• Step 3: Bring down the next number from the dividend, that is, 4. The number to be considered now is 4.
• Step 4: Since 4 is smaller than 24, we will put 0 as the next quotient, since 24 × 0 = 0 and write 0 below 4 to subtract 4 - 0 = 4
• Step 5: Bring down the next number from the dividend, that is, 8 and place it next to this 4. The number to be considered now is 48.
• Step 6: Using the multiplication table of 24, we know that 24 × 2 = 48. So we write 2 in the quotient and 48 below the dividend to subtract these. Subtract 48 - 48 = 0. Thus, remainder = 0 and quotient = 302. This means, 7248 ÷ 24 = 302.

Long Division of Polynomials

When there are no common factors between the numerator and the denominator, or if you can't find the factors, you can use the long division process to simplify the expression. For more details about long division polynomials, visit the Dividing Polynomials page.

Long Division with Decimals

Long division with decimals can be easily done just like the normal division. We just need to keep in mind the decimals and keep copying them as they come. For more details about long division with decimals, visit the Dividing Decimals page.

How to Divide Decimals by Whole Numbers?

When we need to divide decimals by whole numbers, we follow the same procedure of long division and place the decimal in the quotient whenever it comes. Let us understand this with the help of an example.

Example: Divide 36.9 ÷ 3

Solution: Let us see how to divide step by step.

• Step 1: Here, the first digit of the dividend is 3 and it is equal to the divisor. So, 3 ÷ 3 = 1. So, 1 is written on top as the first digit of the quotient and we write the product 3 below the dividend 3.
• Step 2: Subtract 3 - 3 = 0. Bring the second digit of the dividend down and place it beside 0, that is, 6
• Step 3: Using the multiplication table of 3, we know that 3 × 2 = 6. So we write 2 in the quotient and 6 below the dividend to subtract these. Subtract 6 - 6 = 0.
• Step 4: Now comes the decimal point in the dividend. So, place a decimal in the quotient after 12 and continue with the normal division.
• Step 5: Bring down the next number from the dividend, that is, 9. The number to be considered now is 9.
• Step 6: Using the multiplication table of 3, we know that 3 × 3 = 9. So we write 3 in the quotient and 9 below the dividend to subtract these. Subtract 9 - 9 = 0. Thus, remainder = 0 and quotient = 12.3. This means, 36.9 ÷ 3 = 12.3

Long Division Tips and Tricks:

Given below are a few important tips and tricks that would help you while working with long division:

• The remainder is always smaller than the divisor.
• For division, the divisor cannot be 0.
• The division is repeated subtraction, so we can check our quotient by repeated subtractions as well.
• We can verify the quotient and the remainder of the division using the division formula: Dividend = (Divisor × Quotient) + Remainder.
• If the remainder is 0, then we can check our quotient by multiplying it with the divisor. If the product is equal to the dividend, then the quotient is correct.

☛ Related Articles

• Long Division Formula
• Long Division of Polynomials
• Long Division with Remainders Worksheets
• Long Division Without Remainders Worksheets
• Long Division with 2-digit Divisors Worksheets
• Long Division Calculator

Cuemath is one of the world's leading math learning platforms that offers LIVE 1-to-1 online math classes for grades K-12. Our mission is to transform the way children learn math, to help them excel in school and competitive exams. Our expert tutors conduct 2 or more live classes per week, at a pace that matches the child's learning needs.

 

What is Long Division in Math?

Long division is a process to divide large numbers in a convenient way. The number which is divided into smaller groups is known as a dividend, the number by which we divide it is called the divisor, the value received after doing the division is the quotient, and the number left after the division is called the remainder.

How to do Long Division?

The following steps explain the process of long division. This procedure is explained with examples above on this page.

• Write the dividend and the divisor in their respective positions.
• Take the first digit of the dividend from the left.
• If this digit is greater than or equal to the divisor, then divide it by the divisor and write the answer on top as the quotient.
• Write the product below the dividend and subtract the result from the dividend to get the difference. If this difference is less than the divisor, and there are no numbers left in the dividend, then this is considered to be the remainder and the division is done. However, if there are more digits in the dividend to be carried down, we continue with the same process until there are no more digits left in the dividend.

What are the Steps of Long Division?

Given below are the 5 main steps of long division. For example, let us see how we divide 52 by 2.

• Step 1: Consider the first digit of the dividend which is 5 in this example. Here, 5 > 2. We know that 5 is not divisible by 2.
• Step 2: We know that 2 × 2 = 4, so, we write 2 as the quotient.
• Step 3: 5 - 4 = 1 and 1 < 2 (After writing the product 4 below the dividend, we subtract them).
• Step 4: 1 < 2, so we bring down 2 from the dividend and we get 12 as the new dividend now.
• Step 5: Repeat the process till the time you get a remainder less than the divisor. 12 is divisible by 2 as 2 × 6 = 12, so we write 6 in the quotient, and 12 - 12 = 0 (remainder).

Therefore, the quotient is 26 and the remainder is 0.

How to do Long Division with 2 Digits?

For long division with 2 digits, we consider both the digits of the divisor and check for the divisibility of the first two digits of the dividend. If the first 2 digits of the dividend are less than the divisor, then consider the first three digits of the dividend. Proceed with the division in the same way as we divide regular numbers. This procedure is explained with examples above on this page under the heading of 'Long Division by a 2 Digit Number'.

What is the Long Division of Polynomials?

In algebra, the long division of polynomials is an algorithm to divide a polynomial by another polynomial of the same or the lower degree. For example, (4x² - 5x - 21) is a polynomial that can be divided by (x - 3) following some defined rules, which will result in 4x + 7 as the quotient.

How to do Long Division with Decimals?

The long division with decimals is performed in the same way as the normal division. This procedure is explained with examples above on this page under the heading of 'How to Divide Decimals by Whole Numbers'? For more details, visit the page about dividing decimals. The basic steps of long division with decimals are given below.

• Write the division in the standard form.
• Start by dividing the whole number part by the divisor.
• Place the decimal point in the quotient above the decimal point of the dividend.
• Bring down the digits on the tenths place, i.e., the digit after the decimal.
• Divide and bring down the other digit in sequence.
• Divide until all the digits of the dividend are over and a number less than the divisor or 0 is obtained in the remainder.