Rational Expression

A rational expression is an expression in which both numerator and denominator are polynomials. Simplifying rational expressions is done by converting the numerator and denominator to their lowest form. A rational expression is also known as an algebraic fraction.

Let us learn more about rational expression along with operations on rational expressions. Also, we will see how to simplify rational expressions.

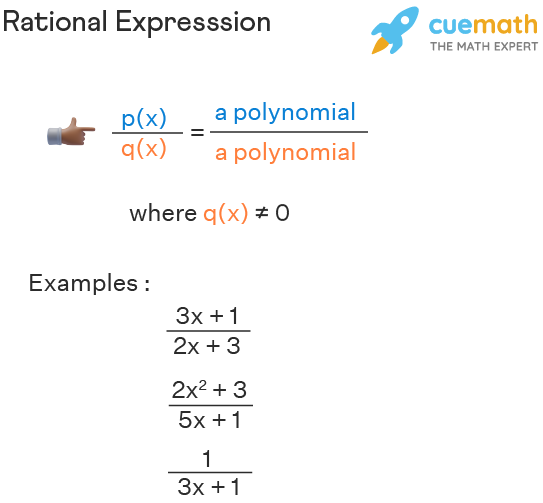
Rational expressions are fractions with variables. In a rational expression, both numerator and denominator are polynomials. i.e., it is of the form p(x)/q(x), where q(x) ≠ 0 and p(x) and q(x) are polynomials. Since rational expressions are nothing but fractions, we operate on them just the way we operate the fractions.

Here are some examples of rational expressions: (x + 1) / (x² - 5), (x³ + 3x² - 5) / (4x - 2), etc. Note that if one of the numerator and denominator is NOT a polynomial, then the fraction is NOT called as a rational expression.

Restrictions of Rational Expression

Dividing a number by 0 is not possible. Check what is 1/0 using your calculator, it will throw an error. Thus, a rational expression (as it is a fraction) is NOT defined for the value(s) of the variable for which the denominator is equal to 0. For example, in the expression x / (x + 2), the denominator becomes zero when x = -2 and hence it is known as the restriction of the rational expression x / (x + 2). In other words, we say x / (x + 2) takes all values for x but x ≠ -2. Thus, to find the restriction(s) of a rational expression just set the denominator equal to 0 and solve for the variable.

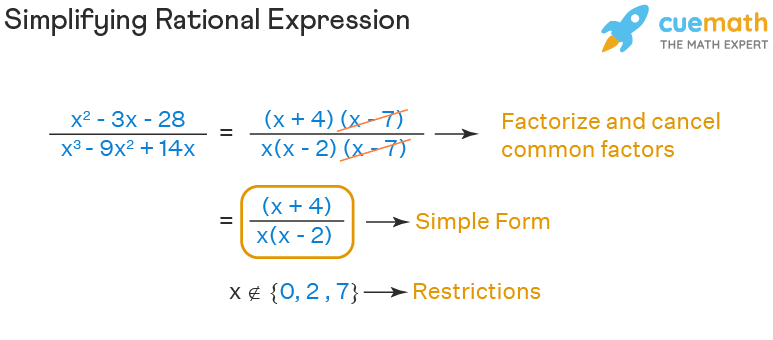
Simplifying rational expressions means reducing the value of a rational expression to its lowest terms or simplified form. The simplification of a rational expression is the same as how we simplify fractions. In fractions, when the numerator and denominator of a rational number have no common factor other than 1, we consider that it is its simplified form. The same thing works for simplifying rational expressions as well but the only difference is of having polynomials in the fraction. Let us look at the steps to be followed for simplifying rational expressions.

• Step 1: Factorize each of the numerator and the denominator by taking the common factors out.
• Step 2: Cancel the common factors.
• Step 3: Write the remaining terms in the numerator and denominator.
• Step 4: Mention the restricted values if any. Note that the restrictions are any values that make the denominator equal to 0, including any canceled terms while simplifying.

Here is another example.

Example: Simplify the rational expression (3y² + 6y) / (6y² + 9y).

Solution:

To simplify the given expression, we just factorize the numerator and denominator and cancel the common terms.

(3y² + 6y) / (6y² + 9y) = [3y (y + 2) ] / [3y (2y + 3)]

= (y + 2) / (2y + 3)

To find the restrictions, set the original denominator ≠ 0 and solve. Note that we shouldn't use the denominator of the simplest form while calculating the restrictions.

3y (2y + 3) ≠ 0

y ≠ 0 and y ≠ -3/2

Thus, the simplest form is (y + 2) / (2y + 3), where y ≠ 0, -3/2.

The process of performing operations on rational expression is as same as that of fractions. i.e., we can add/subtract/multiply/divide the algebraic fractions in the same way as numeric fractions. But while writing the restrictions, the denominator of each rational expression involved in the operation should be taken care of. Let us see how to do each of the following operations on rational expressions:

• Addition
• Subtraction
• Multiplication
• Division

To add/subtract rational expressions, first rewrite the fractions with a common denominator. If the denominators of two rational expressions are already the same, then we can add/subtract their numerators directly. In general, to add/subtract two rational expressions:

• Find the LCD (LCM) of their denominators.
• Multiply and divide each rational expression by some expression/number such that all the denominators are same as LCD found in the above step.
• Now, we can add/subtract all the numerators as now the denominators are the same.

Example: Add the rational expressions (3 / x) + (1 / (x² + x)).

Solution:

The given rational expressions can be written as (3 / x) + [1 / (x(x + 1) ]

Here, the LCD (leat common denominator) is x (x + 1). To make the denominators the same, we multiply and divide the first fraction by (x + 1). Then we get

(3 / x) · (x + 1) / (x + 1) + [1 / (x(x + 1) ]

= (3(x+1)) / (x(x+1)) + 1/(x(x+1))

Now, we can add the numerators as the denominators are the same.

= (3x + 3 + 1) / (x(x+1))

= (3x + 4) / (x(x+1)) (OR) (3x + 4) / (x² + x).

Here, the restrictions are x ≠ 0, -1.

To multiply two rational expressions, we multiply their numerators and their denominators separately. But before this, following these steps is more helpful:

• Simplify each of the expressions if it is not in the simplest form.
• Factorize the numerator and denominator of each expression.
• Cancel the common terms (while cancelation, one term should be in the numerator and the other should be in the denominator).
• Simplify the remaining terms.

Example: Multiply the rational expressions (x² - 3x - 10) / (x² + x - 2) × (x² + 2x - 3) / (x² + x - 6).

Solution:

Factorizing each polynomial,

[(x - 5) (x + 2)] / [(x + 2) (x - 1)] × [(x + 3) (x - 1)] / [(x + 3) (x - 2)]

Now, cancel the terms present in both numerator and denominator.

= [(x - 5) ̶(̶x̶ ̶+̶ ̶2̶)̶] / [ ̶(̶x̶ ̶+̶ ̶2̶)̶ ̶(̶x̶ ̶-̶ ̶1̶)̶] × [ ̶(̶x̶ ̶+̶ ̶3̶)̶ ̶(̶x̶ ̶-̶ ̶1̶)̶] / [ ̶(̶x̶ ̶+̶ ̶3̶)̶ (x - 2)]

= (x - 5) / (x - 2)

Restrictions are x ≠ -2, 1, -3, 2.

Recall to divide one fraction by another fraction, we multiply the first fraction by the reciprocal of the second fraction. We follow the same procedure for dividing rational expressions as well. To divide a rational expression by another:

• Factorize each polynomial in each rational expression.
• To divide by a fraction, multiply by its reciprocal (multiplicative inverse).
• Cancel the common factors and simplify.
• While writing the restricted values, take care of the denominators of the original fractions along with the denominator of the reciprocal fraction that we considered along the way.

Example: Divide the rational expressions [(x² - 9) / (x² + 6x + 9)] ÷ [(x² - 8x + 16) / (x² - 7x + 12)].

Solution:

We will factorize each polynomial first.

= [(x - 3)(x + 3) / (x + 3) (x + 3)] ÷ [(x - 4) (x - 4) / (x - 3) (x - 4)]

Now we will change the "÷" sign into "×" sign by flipping over the second fraction. Note that we have to keep the first fraction as it is.

= [(x - 3)(x + 3) / (x + 3) (x + 3)] × [(x - 3) (x - 4) / (x - 4) (x - 4)]

Now, the division is converted into multiplication. Hence, cancel the possible terms as we do in multiplication.

= [(x - 3) ̶(̶x̶ ̶+̶ ̶3̶)̶ / ̶(̶x̶ ̶+̶ ̶3̶)̶ (x + 3)] × [(x - 3) ̶(̶x̶ ̶-̶ ̶4̶)̶ / ̶(̶x̶ ̶-̶ ̶4̶)̶ (x - 4)]

= (x - 3)² / [(x + 3) (x - 4)]

Restrictions are: x ≠ -3, 3, 4.

Important Notes on Rational Expressions:

• A rational expression must include all values of x except the values that make the denominator to zero.
• To simplify rational expressions, they should be factorized and common factors should be canceled.
• Any arithmetic operation on rational expression is done just as the way the operations are applied on fractions.
• While doing any operation on rational expressions, the denominators of all original expressions and also the expressions that come along the way (especially in division) should be considered while writing restrictions.

☛ Related Topics:

• Rational Expressions Calculator
• Simplifying Rational Expressions Calculator
• Adding and Subtracting Rational Expressions Calculator
• Multiplying And Dividing Rational Expressions Calculator

What is a Rational Expression in Algebra?

A rational expression is a fraction in which the numerator and denominator are polynomials. Every rational expression has at least one restriction. A restriction is a value of the variable that makes the denominator of the rational expression to be zero. For example, x / (x - 1) is not defined when x = 1 and it is the restriction for the given expression.

What are Examples of Rational Expressions?

In a rational expression, the numerator and denominator must be the polynomials. For example, 2x / (x + 3), (x³ - 5) / (x + 2), etc are rational expressions.

How to Simplify a Rational Expression?

To simplify a rational expression, just factorize the numerator and denominator and then cancel the common factors. While taking down the restrictions, the factors that are canceled out also have to be considered.

What is the Process of Adding Rational Expressions?

The process of adding fractions is followed for adding rational expressions as well because rational expressions are nothing but fractions. So to add rational expressions just make the common denominators and add the numerators.

How do You Reduce Rational Expressions to the Lowest Terms?

Rational expressions are reduced to the lowest terms by taking out the common factor from the numerator and denominator.

What is the Difference Between Multiplying and Dividing Rational Expressions?

• For multiplying rational expressions factor out every expression involved and cancel the factors which lie in both numerator and denominator and simplify the rest of the factors.
• For dividing a rational expression (dividend) by another rational expression (divisor), keep the dividend as it is, flip the divisor expression for converting the division symbol into the multiplication symbol and then perform the multiplication.

In multiplication, only the denominators of the original expressions are considered to write the restrictions whereas in the division, along with the denominators of the original expressions, the numerator of the second fraction should also be considered because when the second fraction is flipped, the numerator becomes the denominator.

What is NOT a Rational Expression?

A fraction is called a rational expression if both its numerator and denominator are polynomials. If at least one of them is not a polynomial, then the fraction is NOT a rational expression. For example, √x / (x + 1) is NOT a rational expression as the square root of x is NOT a polynomial.

How to Simplify Rational Expressions With Exponents?

To simplify rational expressions with exponents, we have to follow rules of exponents and powers and then cancel the common factor from the numerator and denominator.

How to Find the Restrictions of Rational Expressions?

To find the restriction of a rational expression, just set each factor of its denominator to zero and solve the equation. This is because a fraction is not defined when its denominator is equal to 0.