Equation
An equation is a mathematical statement with an 'equal to' symbol between two expressions that have equal values. For example, 3x + 5 = 15. There are different types of equations like linear, quadratic, cubic, etc. Let us learn more about equations in math in this article.
| 1. | What are Equations? |
| 2. | Parts of an Equation |
| 3. | How to Solve an Equation? |
| 4. | Types of Equations |
| 5. | Equation vs Expression |
| 6. | FAQs on Equations |
What are Equations?
Equations are mathematical statements containing two algebraic expressions on both sides of an 'equal to (=)' sign. It shows the relationship of equality between the expression written on the left side with the expression written on the right side. In every equation in math, we have, L.H.S = R.H.S (left hand side = right hand side). Equations can be solved to find the value of an unknown variable representing an unknown quantity. If there is no 'equal to' symbol in the statement, it means it is not an equation. It will be considered as an expression. You will learn the difference between equation and expression in the later section of this article.
Look at the following examples. These will give you an idea of the meaning of an equation in math.
| Equations | Is it an equation? | |
|---|---|---|
| 1. | y = 8x - 9 | Yes |
| 2. | y + x2 - 7 | No, because there is no 'equal to' symbol. |
| 3. | 7 + 2 = 10 - 1 | Yes |
Now, let us move forward and learn about parts of an equation in math.
Parts of an Equation
There are different parts of an equation which include coefficients, variables, operators, constants, terms, expressions, and an equal to sign. When we write an equation, it is mandatory to have an "=" sign, and terms on both sides. Both sides should be equal to each other. An equation doesn't need to have multiple terms on either of the sides, having variables, and operators. An equation can be formed without these as well, for example, 5 + 10 = 15. This is an arithmetic equation with no variables. As opposed to this, an equation with variables is an algebraic equation. Look at the image below to understand the parts of an equation.
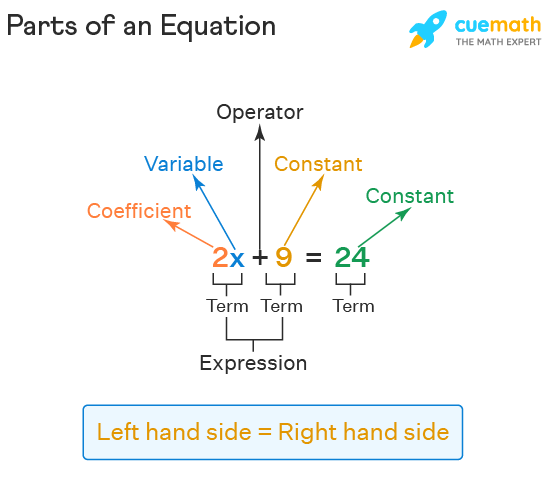
How to Solve an Equation?
An equation is like a weighing balance with equal weights on both sides. If we add or subtract the same number from both sides of an equation, it still holds. Similarly, if we multiply or divide the same number into both sides of an equation, it still holds. Consider the equation of a line, 3x − 2 = 4. We will perform mathematical operations on the LHS and the RHS so that the balance is not disturbed. Let's add 2 on both sides to reduce the LHS to 3x. This will not disturb the balance. The new LHS is 3x − 2 + 2 = 3x and the new RHS is 4 + 2 = 6. So, the equation becomes 3x = 6. Now, let's divide both sides by 3 to reduce the LHS to x. Thus, the solution of the given equation of a line is x = 2.
The steps to solve a basic equation with one variable (linear) are given below:
- Step 1: Bring all the terms with variables on one side and all the constants on the other side of the equation by applying arithmetic operations on both sides.
- Step 2: Combine all like terms (terms containing the same variable with the same exponent) by adding/subtracting them.
- Step 3: Simplify it and get the answer.
Let us take one more example of a basic equation: 3x - 20 = 7. To bring all the constants on RHS, we have to add 20 to both sides. This implies, 3x - 20 + 20 = 7 + 20, which can be simplified as 3x = 27. Now, divide both sides by 3. This will get you x = 9 which is the required solution of the equation.
Types of Equations
Based on the degree, equations can be classified into three types. Following are the three types of equations in math:
- Linear Equations
- Quadratic Equations
- Cubic Equations
Linear Equation
Equations with 1 as the degree are known as linear equations in math. In such equations, 1 is the highest exponent of terms. These can be further classified into linear equations in one variable, two-variable linear equations, with three variables, etc. The standard form of a linear equation with variables X and Y are aX + bY - c = 0, where a and b are the coefficients of X and Y respectively and c is the constant.
Quadratic Equation
Equations with degree 2 are known as quadratic equations. The standard form of a quadratic equation with variable x is ax2 + bx + c = 0, where a ≠ 0. These equations can be solved by splitting the middle term, completing the square, or by the discriminant method.
Cubic Equations
Equations with degree 3 are known as cubic equations. Here, 3 is the highest exponent of at least one of the terms. The standard form of a cubic equation with variable x is ax3 + bx2 + cx + d = 0, where a ≠ 0.
Equation vs Expression
Expressions and equations in math are used simultaneously in algebra, but there is a major difference between the two terms. When 2x + 4 is an expression, 2x + 4 = 0 is considered an equation. Let us understand the basic difference between equation and expression through the table given below:
| Equation | Expression |
|---|---|
| When two expressions are equal in value and written together with an 'equal to' sign in between, it is known as an equation in math. | It is a mathematical statement having at least one term or multiple terms connected through operators in between. |
| It has an equal to "=" sign. | An expression does not contain an equal to "=" sign. |
| It can be solved to find the value of the unknown quantity. | It can be simplified to the lowest form. |
| Example: x - 8 = 16, 6y = 33, 3z - 7y = 9, etc. | Example: x - 8, 6y, 3z - 7y - 9, etc. |
Important Notes on Equations in Math:
- The values of the variable that makes an equation true are called the solution or root of the equation.
- The solution of an equation is unaffected if the same number is added, subtracted, multiplied, or divided into both sides of the equation.
- The graph of a linear equation in one or two variables is a straight line.
- The curve of the quadratic equation is in the form of a parabola.
☛ Related Topics:
Check these interesting articles related to the concept of equations in math.
Equation Examples
-
Example 1: Meghan goes to a shop to purchase some pens and notebooks and realizes that the cost of a notebook is $2 more than twice the cost of the pen. Represent this information using equations in two variables.
Solution: Let's assume the following:
The cost of a pen = $x
The cost of a notebook = $y
Answer: According to the given information, y = 2x + 2. This is the required equation.
-
Example 2: Solve the following equation: 2x = 3x - 20.
Solution: The given equation '2x = 3x - 20' can be solved using the following steps:
- Add 20 to both sides of the equation. It will result to 2x + 20 = 3x - 20 + 20, which will be simplified to 2x + 20 = 3x.
- Subtract 2x from both sides. This implies, 2x - 2x + 20 = 3x - 2x which is equal to 20 = x.
Answer: Therefore, the value of x is 20.
-
Example 3: Hailey knows that the total sum in her piggy bank is $100 and it has 3 times as many coins of $5 as $10 in it. She wants to know the exact number of coins of $5 and $10 in her piggy bank.
Can you help her find the count?
Solution:
Let the number of coins of $10 be x. Then the number of $5 coins will be 3x. Therefore, total amount = (10 × x) + (5 × 3x) = 10x + 15x = 25x. According to the information we have,
25x = 100
x = 4
Answer: Therefore, she has 4 $10 and (3 × 4) = 12 $5 coins.

FAQs on Equation
What is an Equation in Math?
An equation in math is an equality relationship between two expressions written on both sides of the equal to sign. For example, 3y = 16 is an equation.
What is a Linear Equation?
A linear equation is an equation with degree 1. It means the highest exponent of any term could be 1. An example of a linear equation in math is x + y = 24.
What is a Quadratic Equation?
A quadratic equation is an equation with degree 2. It can have any number of variables but the highest power of terms could be only 2. The standard form of a quadratic equation with variable y is ay2 + by + c = 0, where a ≠ 0.
How Equations are Used in Real Life?
In real life, there are many situations in which equations can be used. Whenever an unknown quantity has to be found, an equation can be formed and solved. For example, if the cost of 1 pencil is $1.2, and the total money spent by you on pencils is $9.6, the number of pencils bought can be found by forming an equation based on the given information. Let the number of pencils bought be x. Then, the equation will be 1.2x = 9.6, which can be solved as x = 8.
How to Solve Quadratic Equations?
Quadratic equations in one variable can be solved using the following methods:
What are the 3 Types of Equation?
Based on the degree, equations can be classified into the following three types:
- Linear equation
- Quadratic equation
- Cubic equation
What Equation Has No Solution?
Equations of two parallel lines have no solutions as they do not intersect at any point. To identify the equations of parallel lines, we have to compare the coefficients of both the variables in the given two linear equations in two variables. If the ratio of coefficients is the same and unequal to the ratio of constants, it means those equations have no solution. For two equations ax + by + c = 0 and px + qy + r = 0, it will have no solution when a/p = b/q ≠ c/r.
What is the Equation of a Circle?
The equation of a circle with radius r and center (x1, y1) is (x − x1)2 + (y − y1)2 = r2.
visual curriculum