Quadratic Equation

Quadratic equations are second-degree algebraic expressions and are of the form ax² + bx + c = 0. The term "quadratic" comes from the Latin word "quadratus" meaning square, which refers to the fact that the variable x is squared in the equation. In other words, a quadratic equation is an “equation of degree 2.” There are many scenarios where a quadratic equation is used. Did you know that when a rocket is launched, its path is described by a quadratic equation? Further, a quadratic equation has numerous applications in physics, engineering, astronomy, etc.

Quadratic equations have maximum of two solutions, which can be real or complex numbers. These two solutions (values of x) are also called the roots of the quadratic equations and are designated as (α, β). We shall learn more about the roots of a quadratic equation in the below content.

A quadratic equation is an algebraic equation of the second degree in x. The quadratic equation in its standard form is ax² + bx + c = 0, where a and b are the coefficients, x is the variable, and c is the constant term. The important condition for an equation to be a quadratic equation is the coefficient of x² is a non-zero term (a ≠ 0). For writing a quadratic equation in standard form, the x² term is written first, followed by the x term, and finally, the constant term is written.

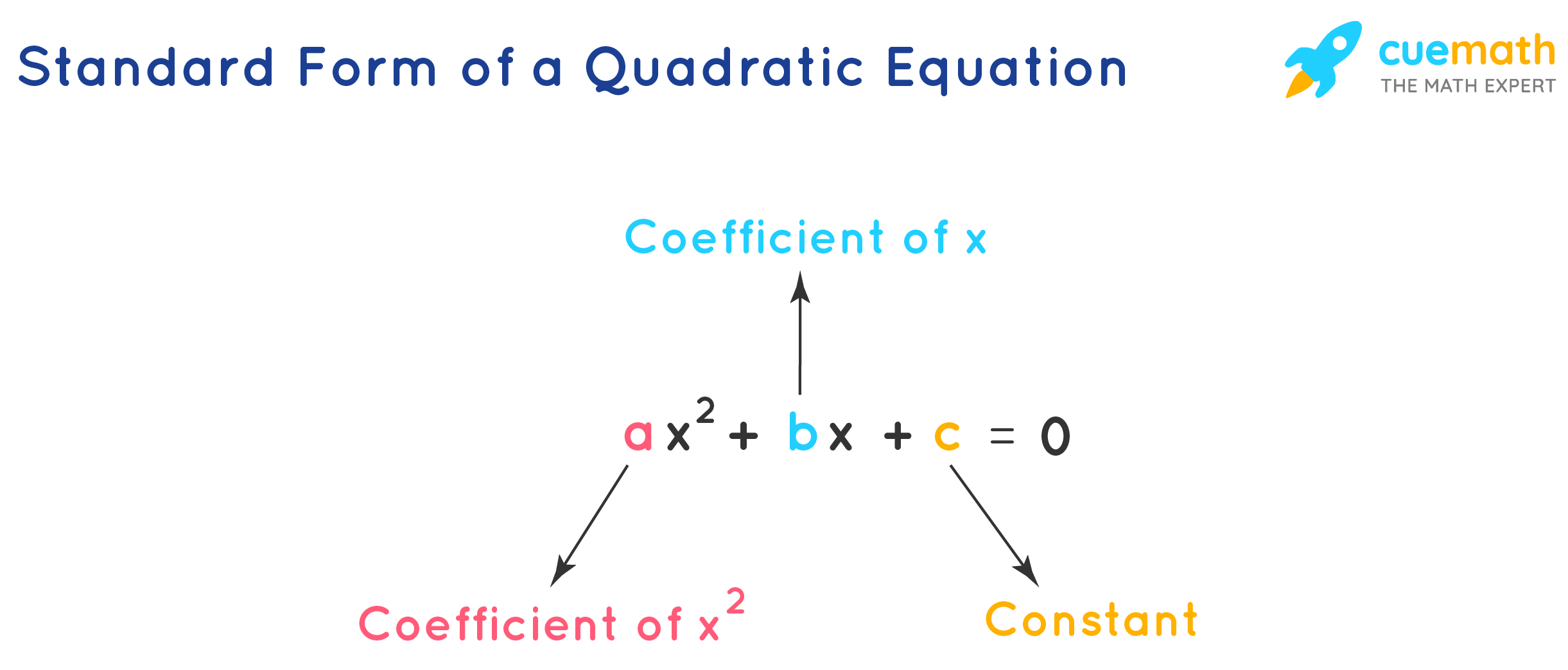
Further, in real math problems the quadratic equations are presented in different forms: (x - 1)(x + 2) = 0, -x² = -3x + 1, 5x(x + 3) = 12x, x³ = x(x² + x - 3). All of these equations need to be transformed into standard form of the quadratic equation before performing further operations.

The roots of a quadratic equation are the two values of x, which are obtained by solving the quadratic equation. These roots of the quadratic equation are also called the zeros of the equation. For example, the roots of the equation x² - 3x - 4 = 0 are x = -1 and x = 4 because each of them satisfies the equation. i.e.,

• At x = -1, (-1)² - 3(-1) - 4 = 1 + 3 - 4 = 0
• At x = 4, (4)² - 3(4) - 4 = 16 - 12 - 4 = 0

There are various methods to find the roots of a quadratic equation. The usage of the quadratic formula is one of them.

Quadratic formula is the simplest way to find the roots of a quadratic equation. There are certain quadratic equations that cannot be easily factorized, and here we can conveniently use this quadratic formula to find the roots in the quickest possible way. The two roots in the quadratic formula are presented as a single expression. The positive sign and the negative sign can be alternatively used to obtain the two distinct roots of the equation.

Quadratic Formula: The roots of a quadratic equation ax² + bx + c = 0 are given by x = [-b ± √(b² - 4ac)]/2a.

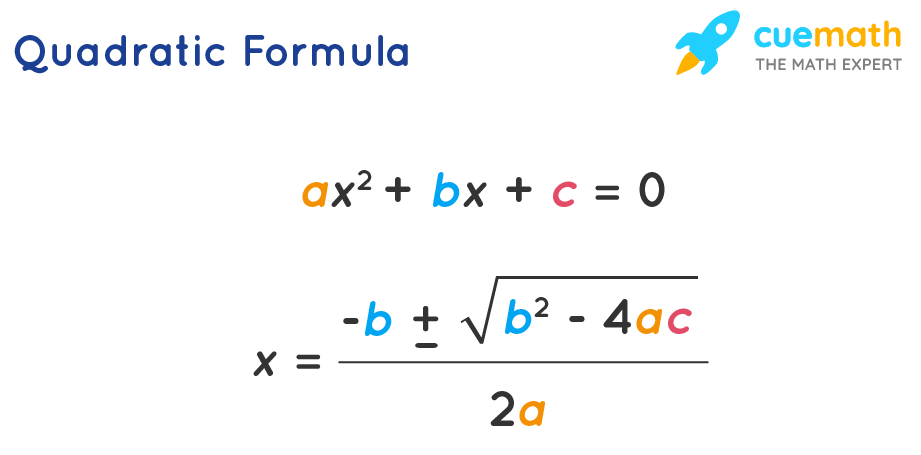
This formula is also known as the Sridharacharya formula.

Example: Let us find the roots of the same equation that was mentioned in the earlier section x² - 3x - 4 = 0 using the quadratic formula.

a = 1, b = -3, and c = -4.

x = [-b ± √(b² - 4ac)]/2a
= [-(-3) ± √((-3)² - 4(1)(-4))]/2(1)
= [3 ± √25] / 2
= [3 ± 5] / 2
= (3 + 5)/2 or (3 - 5)/2
= 8/2 or -2/2
= 4 or -1 are the roots.

Proof of Quadratic Formula

Consider an arbitrary quadratic equation: ax² + bx + c = 0, a ≠ 0

To determine the roots of this equation, we proceed as follows:

ax² + bx = -c ⇒ x² + bx/a = -c/a

Now, we express the left-hand side as a perfect square, by introducing a new term (b/2a)² on both sides:

x²+ bx/a + (b/2a)² = -c/a + (b/2a)²

The left-hand side is now a perfect square:

(x + b/2a)² = -c/a + b²/4a² ⇒ (x + b/2a)² = (b² - 4ac)/4a²

This is good for us, because now we can take square roots to obtain:

x + b/2a = ±√(b² - 4ac)/2a

x = (-b ± √(b² - 4ac))/2a

Thus, by completing the squares, we were able to isolate x and obtain the two roots of the equation.

The roots of a quadratic equation are usually represented to by the symbols alpha (α), and beta (β). Here we shall learn more about how to find the nature of roots of a quadratic equation without actually finding the roots of the equation.

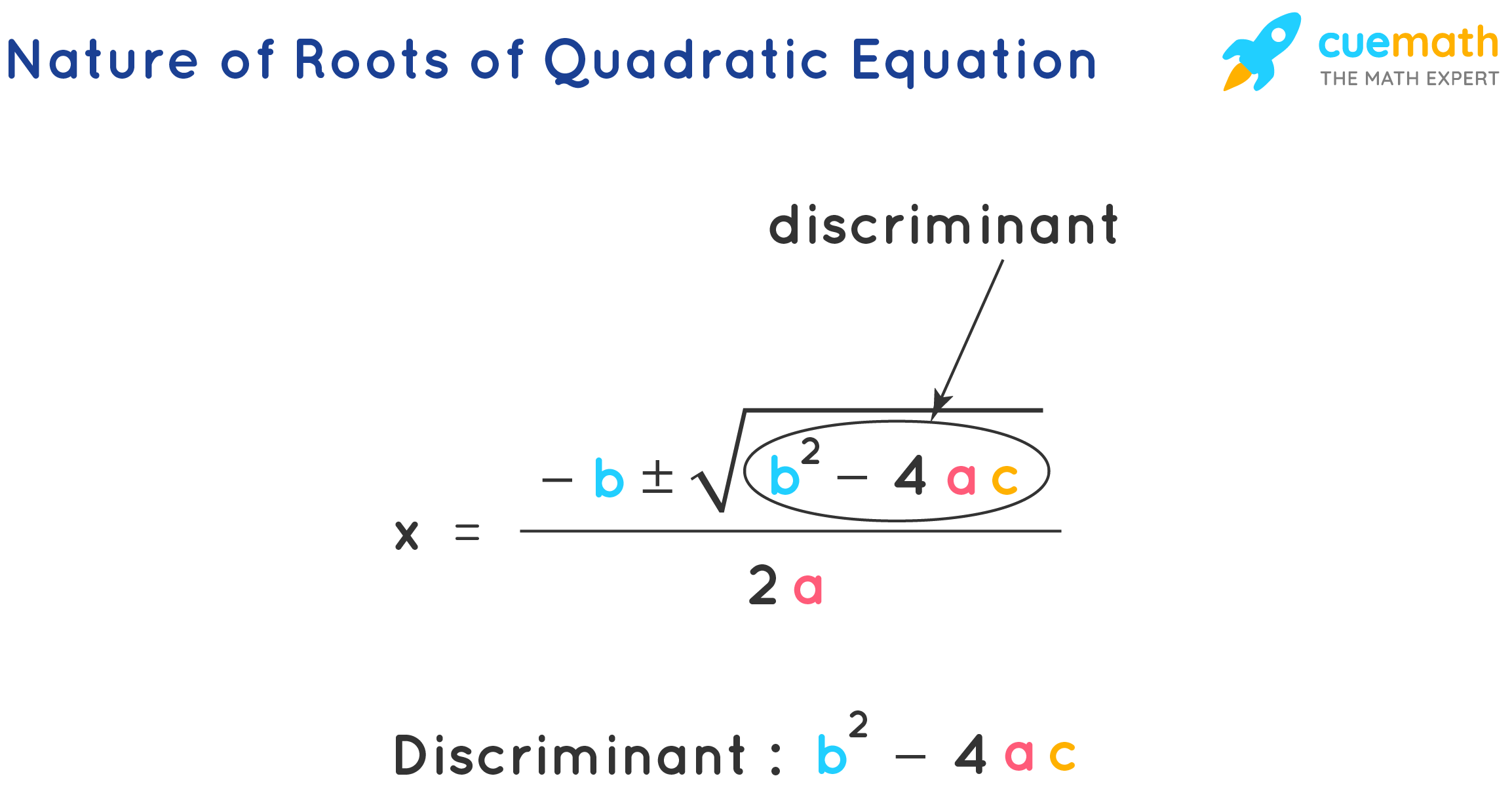
The nature of roots of a quadratic equation can be found without actually finding the roots (α, β) of the equation. This is possible by taking the discriminant value, which is part of the formula to solve the quadratic equation. The value b² - 4ac is called the discriminant of a quadratic equation and is designated as 'D'. Based on the discriminant value the nature of the roots of the quadratic equation can be predicted.

Discriminant: D = b² - 4ac

• D > 0, the roots are real and distinct
• D = 0, the roots are real and equal.
• D < 0, the roots do not exist or the roots are imaginary.

Now, check out the formulas to find the sum and the product of the roots of the equation.

Sum and Product of Roots of Quadratic Equation

The coefficient of x², x term, and the constant term of the quadratic equation ax² + bx + c = 0 are useful in determining the sum and product of the roots of the quadratic equation. The sum and product of the roots of a quadratic equation can be directly calculated from the equation, without actually finding the roots of the quadratic equation. For a quadratic equation ax² + bx + c = 0, the sum and product of the roots are as follows.

• Sum of the Roots: α + β = -b/a = - Coefficient of x/ Coefficient of x²
• Product of the Roots: αβ = c/a = Constant term/ Coefficient of x²

Writing Quadratic Equations Using Roots

The quadratic equation can also be formed for the given roots of the equation. If α, β, are the roots of the quadratic equation, then the quadratic equation is as follows.

x² - (α + β)x + αβ = 0

Example: What is the quadratic equation whose roots are 4 and -1?

Solution: It is given that α = 4 and β = -1. The corresponding quadratic equation is found by:

x² - (α + β)x + αβ = 0
x² - (α + β)x + αβ = 0
x² - (4 - 1)x + (4)(-1) = 0
x² - 3x - 4 = 0

The following list of important formulas is helpful to solve quadratic equations.

• The quadratic equation in its standard form is ax² + bx + c = 0
• The discriminant of the quadratic equation is D = b² - 4ac
  • For D > 0 the roots are real and distinct.
  • For D = 0 the roots are real and equal.
  • For D < 0 the real roots do not exist, or the roots are imaginary.
• The formula to find the roots of the quadratic equation is x = [-b ± √(b² - 4ac)]/2a.
• The sum of the roots of a quadratic equation is α + β = -b/a.
• The product of the Root of the quadratic equation is αβ = c/a.
• The quadratic equation whose roots are α, β, is x² - (α + β)x + αβ = 0.
• The condition for the quadratic equations a₁x² + b₁x + c₁ = 0, and a₂x² + b₂x + c₂ = 0 having the same roots is (a₁b₂ - a₂b₁) (b₁c₂ - b₂c₁) = (a₂c₁ - a₁c₂)².
• When a > 0, the quadratic expression f(x) = ax² + bx + c has a minimum value at x = -b/2a.
• When a < 0, the quadratic expression f(x) = ax² + bx + c has a maximum value at x = -b/2a.
• The domain of any quadratic function is the set of all real numbers.

A quadratic equation can be solved to obtain two values of x or the two roots of the equation. There are four different methods to find the roots of the quadratic equation. The four methods of solving the quadratic equations are as follows.

• Factorizing of Quadratic Equation
• Using quadratic formula (which we have seen already)
• Method of Completing the Square
• Graphing Method to Find the Roots

Let us look in detail at each of the above methods to understand how to use these methods, their applications, and their uses.

Factorization of quadratic equation follows a sequence of steps. For a general form of the quadratic equation ax² + bx + c = 0, we need to first split the middle term into two terms, such that the product of the terms is equal to the constant term. Further, we can take the common terms from the available term, to finally obtain the required factors as follows:

• x² + (a + b)x + ab = 0
• x² + ax + bx + ab = 0
• x(x + a) + b(x + a)
• (x + a)(x + b) = 0

Here is an example to understand the factorization process.

• x² + 5x + 6 = 0
• x² + 2x + 3x + 6 = 0
• x(x + 2) + 3(x + 2) = 0
• (x + 2)(x + 3) = 0

Thus the two obtained factors of the quadratic equation are (x + 2) and (x + 3). To find its roots, just set each factor to zero and solve for x. i.e., x + 2 = 0 and x + 3 = 0 which gives x = -2 and x = -3. Thus, x = -2 and x = -3 are the roots of x² + 5x + 6 = 0.

Further, there is another important method of solving a quadratic equation. The method of completing the square for a quadratic equation is also useful to find the roots of the equation.

The method of completing the square in a quadratic equation is to algebraically square and simplify, to obtain the required roots of the equation. Consider a quadratic equation ax² + bx + c = 0, a ≠ 0. To determine the roots of this equation, we simplify it as follows:

• ax² + bx + c = 0
• ax² + bx = -c
• x² + bx/a = -c/a

Now, we express the left-hand side as a perfect square, by introducing a new term (b/2a)² on both sides:

• x² + bx/a + (b/2a)² = -c/a + (b/2a)²
• (x + b/2a)² = -c/a + b²/4a²
• (x + b/2a)² = (b² - 4ac)/4a²
• x + b/2a = +√(b²- 4ac)/2a
• x = - b/2a +√(b²- 4ac)/2a
• x = [-b ± √(b² - 4ac)]/2a

Here the '+' sign gives one root and the '-' sign gives another root of the quadratic equation. Generally, this detailed method is avoided, and only the quadratic formula is used to obtain the required roots.

The graph of the quadratic equation ax² + bx + c = 0 can be obtained by representing the quadratic equation as a function y = ax² + bx + c. Further by solving and substituting values for x, we can obtain values of y, we can obtain numerous points. These points can be presented in the coordinate axis to obtain a parabola-shaped graph for the quadratic equation. For detailed information about graphing a quadratic function, click here.

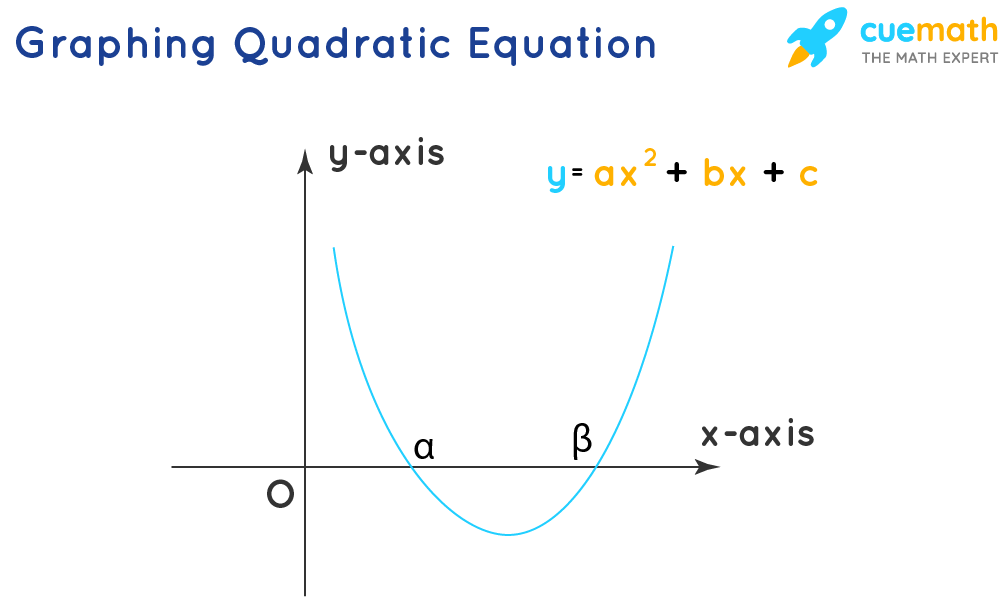
The point(s) where the graph cuts the horizontal x-axis (typically the x-intercepts) is the solution of the quadratic equation. These points can also be algebraically obtained by equalizing the y value to 0 in the function y = ax² + bx + c and solving for x.

Consider two quadratic equations having common roots a₁x² + b₁x + c₁ = 0, and a₂x² + b₂x + c₂ = 0. Let us solve these two equations to find the conditions for which these equations have a common root. The two equations are solved for x² and x respectively.

(x²)(b₁c₂ - b₂c₁) = (-x)/(a₁c₂ - a₂c₁) = 1/(a₁b₂ - a₂b₁)

x² = (b₁c₂ - b₂c₁) / (a₁b₂ - a₂b₁)

x = (a₂c₁ - a₁c₂) / (a₁b₂ - a₂b₁)

Hence, by simplifying the above two expressions we have the following condition for the two equations having the common root.

(a₁b₂ - a₂b₁) (b₁c₂ - b₂c₁) = (a₂c₁ - a₁c₂)²

The maximum and minimum values for the quadratic function F(x) = ax² + bx + c can be observed in the below graphs. For positive values of a (a > 0), the quadratic expression has a minimum value at x = -b/2a, and for negative value of a (a < 0), the quadratic expression has a maximum value at x = -b/2a. x = -b/2a is the x-coordinate of the vertex of the parabola.

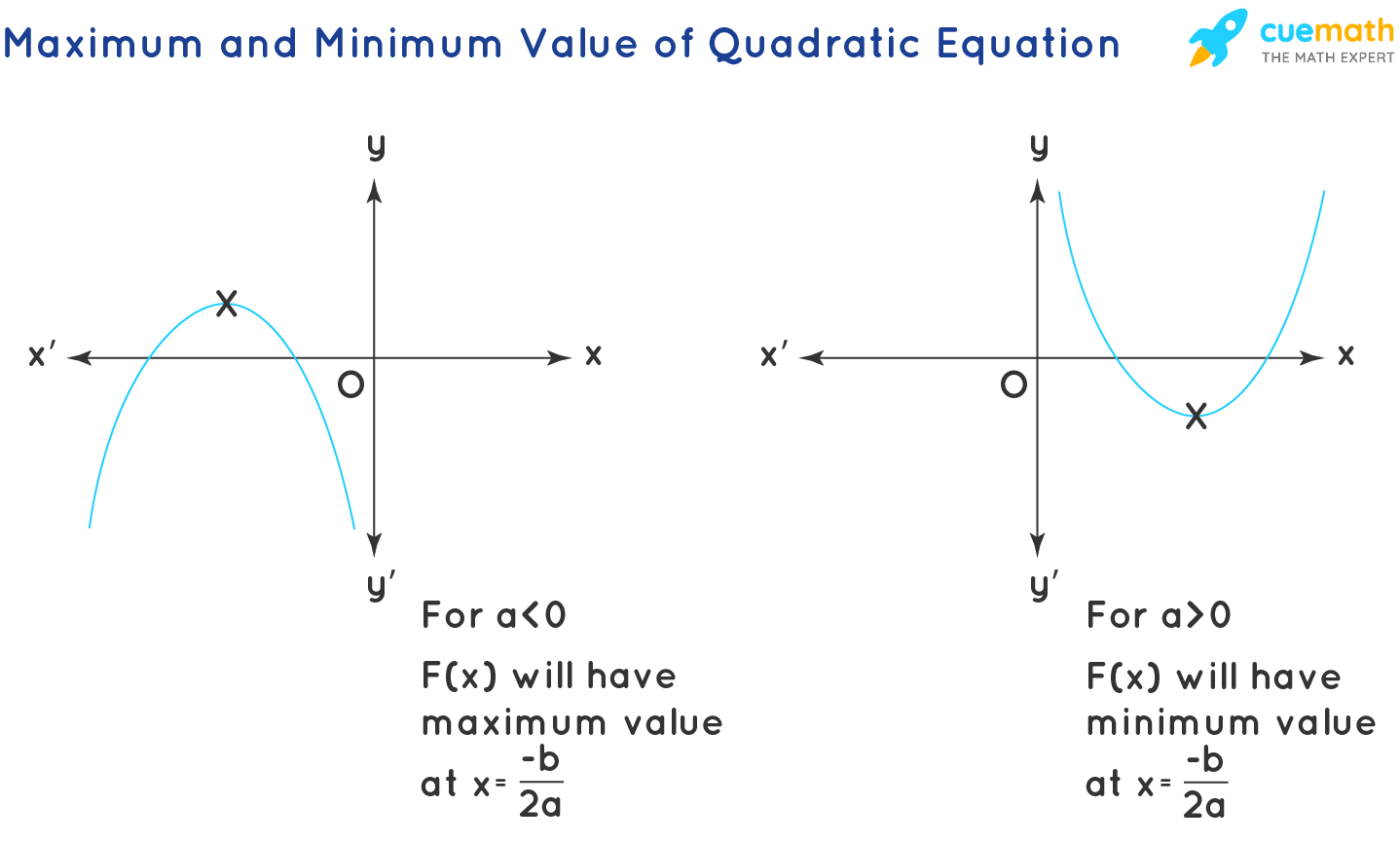
The maximum and minimum values of the quadratic expressions are of further help to find the range of the quadratic expression: The range of the quadratic expressions also depends on the value of a. For positive values of a( a > 0), the range is [ F(-b/2a), ∞), and for negative values of a ( a < 0), the range is (-∞, F(-b/2a)].

• For a > 0, Range: [ f(-b/2a), ∞)
• For a < 0, Range: (-∞, f(-b/2a)]

Note that the domain of a quadratic function is the set of all real numbers, i.e., (-∞, ∞).

Tips and Tricks on Quadratic Equation:

Some of the below-given tips and tricks on quadratic equations are helpful to more easily solve quadratic equations.

• The quadratic equations are generally solved through factorization. But in instances when it cannot be solved by factorization, the quadratic formula is used.
• The roots of a quadratic equation are also called the zeroes of the equation.
• For quadratic equations having negative discriminant values, the roots are represented by complex numbers.
• The sum and product of the roots of a quadratic equation can be used to find higher algebraic expressions involving these roots.

☛Related Topics:

• Roots Calculator
• Quadratic Factoring Calculator
• Roots of Quadratic Equation Calculator

Cuemath is one of the world's leading math learning platforms that offers LIVE 1-to-1 online math classes for grades K-12. Our mission is to transform the way children learn math, to help them excel in school and competitive exams. Our expert tutors conduct 2 or more live classes per week, at a pace that matches the child's learning needs.

What is the Definition of a Quadratic Equation?

A quadratic equation in math is a second-degree equation of the form ax² + bx + c = 0. Here a and b are the coefficients, c is the constant term, and x is the variable. Since the variable x is of the second degree, there are two roots or answers for this quadratic equation. The roots of the quadratic equation can be found by either solving by factorizing or through the use of the quadratic formula.

What is the Quadratic Formula?

The quadratic equation formula to solve the equation ax² + bx + c = 0 is x = [-b ± √(b² - 4ac)]/2a. Here we obtain the two values of x, by applying the plus and minus symbols in this formula. Hence the two possible values of x are [-b + √(b² - 4ac)]/2a, and [-b - √(b² - 4ac)]/2a.

How do You Solve a Quadratic Equation?

There are several methods to solve quadratic equations, but the most common ones are factoring, using the quadratic formula, and completing the square.

• Factoring involves finding two numbers that multiply to equal the constant term, c, and add up to the coefficient of x, b.
• The quadratic formula is used when factoring is not possible, and it is given by x = [-b ± √(b² - 4ac)]/2a.
• Completing the square involves rewriting the quadratic equation in a different form that allows you to easily solve for x.

What is Determinant in Quadratic Formula?

The value b² - 4ac is called the discriminant and is designated as D. The discriminant is part of the quadratic formula. The discriminants help us to find the nature of the roots of the quadratic equation, without actually finding the roots of the quadratic equation.

What are Some Real-Life Applications of Quadratic Equations?

Quadratic equations are used to find the zeroes of the parabola and its axis of symmetry. There are many real-world applications of quadratic equations.

• They can be used in running time problems to evaluate the speed, distance or time while traveling by car, train or plane.
• Quadratic equations describe the relationship between quantity and the price of a commodity.
• Similarly, demand and cost calculations are also considered quadratic equation problems.
• It can also be noted that a satellite dish or a reflecting telescope has a shape that is defined by a quadratic equation.

How are Quadratic Equations Different From Linear Equations?

A linear degree is an equation of a single degree and one variable, and a quadratic equation is an equation in two degrees and a single variable. A linear equation is of the the form ax + b = 0 and a quadratic equation is of the form ax² + bx + c = 0. A linear equation has a single root and a quadratic equation has two roots or two answers. Also, a quadratic equation is a product of two linear equations.

What Are the 4 Ways To Solve A Quadratic Equation?

The four ways of solving a quadratic equation are as follows.

• Factorizing method
• Roots of Quadratic Equation Formula Method
• Method of Completing Squares
• Graphing Method

How to Solve a Quadratic Equation by Completing the Square?

The quadratic equation is solved by the method of completing the square and it uses the formula (a + b)^2 = a² + 2ab + b² (or) (a - b)^2 = a² - 2ab + b².

How to Find the Value of the Discriminant?

The value of the discriminant in a quadratic equation can be found from the variables and constant terms of the standard form of the quadratic equation ax² + bx + c = 0. The value of the discriminant is D = b² - 4ac, and it helps to predict the nature of roots of the quadratic equation, without actually finding the roots of the equation.

How Do You Solve Quadratic Equations With Graphing?

The quadratic equation can be solved similarly to a linear equal by graphing. Let us take the quadratic equation ax² + bx + c = 0 as y = ax² + bx + c . Here we take the set of values of x and y and plot the graph. The two points where this graph meets the x-axis, are the solutions of this quadratic equation.

How Important Is the Discriminant of a Quadratic Equation?

The discriminant is very much needed to easily find the nature of the roots of the quadratic equation. Without the discriminant, finding the nature of the roots of the equation is a long process, as we first need to solve the equation to find both the roots. Hence the discriminant is an important and needed quantity, which helps to easily find the nature of the roots of the quadratic equation.

Where Can I Find Quadratic Equation Solver?

To get the quadratic equation solver, click here. Here, we can enter the values of a, b, and c for the quadratic equation ax² + bx + c = 0, then it will give you the roots along with a step-by-step procedure.

What is the Use of Discriminants in Quadratic Formula?

The discriminant (D = b² - 4ac) is useful to predict the nature of the roots of the quadratic equation. For D > 0, the roots are real and distinct, for D = 0 the roots are real and equal, and for D < 0, the roots do not exist or the roots are imaginary complex numbers. With the help of this discriminant and with the least calculations, we can find the nature of the roots of the quadratic equation.

How do you Solve a Quadratic Equation without Using the Quadratic Formula?

There are two alternative methods to the quadratic formula. One method is to solve the quadratic equation through factorization, and another method is by completing the squares. In total there are three methods to find the roots of a quadratic equation.

How to Derive Quadratic Formula?

The algebra formula (a + b)² = a² + 2ab + b² is used to solve the quadratic equation and derive the quadratic formula. This algebraic formula is used to manipulate the quadratic equation and derive the quadratic formula to find the roots of the equation.