Set Builder Notation

Set builder notation is one of the ways of representing sets in the most convenient and concise way. Here, the common property of all elements of the set is used to define the set. We may need to describe the set of all natural numbers divisible by 3, set of all prime numbers, set of 2x2 matrices, etc. The set builder notation is very useful in all such instances.

Let us learn more about the symbols used in set-builder notation, the domain, and range, and the uses of set-builder notation, with the help of examples, and FAQs.

Set-builder notation is defined as a representation or a notation that can be used to describe a set that is defined by a logical formula that simplifies to be true for every element of the set. It includes one or more than one variables. It also defines a rule about the elements which belong to the set.

There are two methods that can be used to represent a set: Roster form and Set builder form.

The roster form or listing the individual elements of the sets, and the set builder form of representing the elements with a statement or an equation or an inequality. The two methods are as follows.

Roster Form (Listing the Elements)

In this method, we list down all the elements of a set, and they are represented inside curly brackets. Each of the elements is written only once and is separated by commas. For example, the set of letters in the word, "California" is written as A = {c, a, l, i, f, o, r, n}.

Set Builder Form (Defining a Rule For the Elements)

Set builder form uses a statement or a math equation to represent all the elements of a set. In this method, we do not list the elements; instead, we will write the representative element using a variable followed by a vertical line or colon and write the general property of the same representative element. For example, the same set above (that denotes the set of letters in the word, "California") in set builder form can be written as

• A = {x | x is a letter of the word "California"} (or)

• A = {x : x is a letter of the word "California"}.

Here is another example of writing the set of odd positive integers below 11 in both forms.

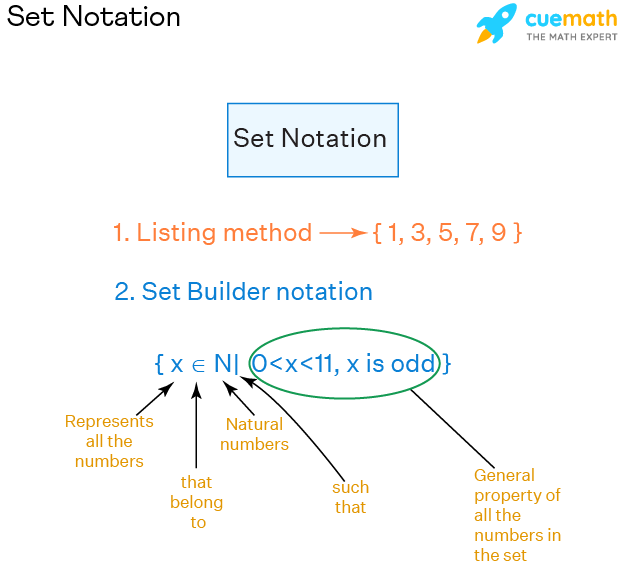
Here are some more set builder form examples.

Example 1:

A = {x | x ∈ ℕ, 5 < x < 10} and is read as "set A is the set of all ‘x’ such that ‘x’ is a natural number between 5 and 10."

The symbol ∈ ("belongs to") means “is an element of” and denotes membership of an element in a set.

Example 2:

B = { x | x is a two-digit odd number from 11 to 20} which means set B contains all the odd numbers from 11 to 20. By using the roster method, set B can be written as B = {11, 13, 15, 17, 19}.

Example 3:

Q is the set of rational numbers that can be written in set builder form as Q = {p/q | p, q ∈ ℤ, q≠0}. The above is read as ‘Q’ is the set of all numbers in the form q/p such that p and q are integers where q is not equal to zero.’

The set builder form uses various symbols to represent the elements of the set. A few of the symbols are listed as follows.

• | is read as "such that" and we usually write it immediately after the variable in the set builder form and after this symbol, the condition of the set is written.
• ∈ is read as "belongs to" and it means "is an element of".
• ∉ is read as "does not belong to" and it means "is not an element of".
• ℕ represents natural numbers or all positive integers.
• W represents whole numbers.
• ℤ indicates integers.
• ℚ represents rational numbers or any number that can be expressed as a fraction of integers.
• ℝ represents real numbers or any number that isn't imaginary.

Set builder notation is used when there are numerous elements and we are not able to easily represent the elements of the set by using the roster form. Let us understand this with the help of an example. If you have to list a set of integers between 1 and 8 inclusive, one can simply use roster notation to write {1, 2, 3, 4, 5, 6, 7, 8}. But the problem arises when we have to list all the real numbers. Using roster notation would not be practical in this case. {..., 1, 1.1, 1.01, 1.001, 1.0001, ... }. But using the set-builder notation would be better in this scenario. The set builder form of real numbers is {x | x is a real number} (or) {x | x is rational or irrational number}.

Set-builder notation comes in handy to write sets, especially for sets with an infinite number of elements. Numbers such as integers, real numbers, and natural numbers can be expressed using set-builder notation. A set with an interval or an equation can also be expressed using this method.

Set builder notation is very useful for writing the domain and range of a function. In its simplest form, the domain is the set of all the values that go into a function. For Example: For the rational function, f(x) = 2/(x-1) the domain would be all real numbers, except 1. This is because the function f(x) would be undefined when x = 1. Thus, the domain for the above function can be expressed as {x ∈ R | x ≠ 1}. Similarly, we can represent the range of a function as well using the set builder notation.

Set builder notation is represented using interval notation, and it is a way to define a set of numbers between a lower limit and an upper limit using end-point values. The upper and lower limits may or may not be included in the set. The end-point values are written between brackets or parentheses. A square bracket denotes inclusion in the set, while a parenthesis denotes exclusion from the set. For example, (4,12]. This interval notation denotes that this set includes all real numbers between 4 and 12 and 12 is included (as it has a square bracket at 12) in the set while 4 (as it has parenthesis at 4) is not a part of the set. Suppose we want to express the set of real numbers {x |-2 < x < 5} using an interval. This can be expressed as interval notation (-2, 5) and it is shown on the number line below:

The set of real numbers can be expressed as the interval (-∞, ∞) as follows:

Important Notes on Set Builder Form:

• To represent the sets with many/infinite numbers of elements, the set builder form is used.
• Take care of the brackets/parenthesis at the endpoints while using intervals in the set builder form.
• Interval is another way of writing an inequality.

☛Related Articles:

• Operations on Sets
• Venn Diagrams
• Subset
• Universal Set

What is the Definition of Set-Builder Notation in Math?

Set builder notation is a mathematical notation for describing a set by representing its elements or explaining the properties that its members must satisfy. For example, C = {2,4,5} denotes a set of three numbers: 2, 4, and 5, and D ={(2,4),(−1,5)} denotes a set of two ordered pairs of numbers. Another option is to use the set-builder notation: F = {n³: n is an integer with 1≤n≤100} is the set of cubes of the first 100 positive integers.

What is the Difference Between Roster Form and Set Builder Form?

• The roster form of a set is just listing the elements of the set inside the curly brackets and is useful when the set has less number of elements. Example: {1, 2, 3, 4, 5}.
• The set builder form of a set is defining the elements of a set by a common characteristic of the elements. Example: The above set in the set-builder notation is {x ∈ ℕ | x ≤ 5}. This form is mainly used to define the larger sets.

What is Interval Notation and Set Builder Notation Form?

• In the Interval notation, the end-point values are written between brackets or parentheses. A square bracket represents that an element is included in the set, whereas a parenthesis denotes exclusion from the set.
  For example, (8,12]. This interval notation denotes that this set includes all real numbers between 8 and 12 where 8 is excluded and 12 is included.
• The set-builder notation is a mathematical notation for describing a set by representing its elements or explaining the properties that its members must satisfy.
  For example, For the given set A = {..., -3, -2, -1, 0, 1, 2, 3, 4}, the set builder notation is A = {x ∈ ℤ | x ≤ 4 }.

What is Set Builder Notation Example?

A set-builder notation describes the elements of a set instead of listing the elements. For example, the set {5, 6, 7, 8, 9} lists the elements. We read the set {x is a counting number between 4 and 10} as the set of all x such that x is a number greater than 4 and less than 10. Technically, the same set in the set builder form can be {x | x ∈ N and 4 < x < 10} (or) {x | x ∈ N and 5 ≤ x ≤ 9}.

How do you Write a Domain in Set Builder Notation?

We can write the domain of f(x) = 1/x in set builder notation as, {x ∈ ℝ | x ≠ 0}. If the domain of a function is all real numbers we can state the domain as, 'all real numbers,'. Also, we can use the interval (-∞, ∞) to represent all real numbers.

How do you Write Inequalities in Set Builder Notation?

The inequalities in sets builder notation is written using >, <, ≥, ≤, symbols. For example, the set { x | x ∈ ℝ, x ≥ 2 and x ≤ 6 } indicates all the real numbers between 2 and 6 both inclusive.

How do you Express Intervals in Set Builder Form?

We can use the intervals while writing the set builder form depending on the situation. Example For the given set A = {..., -3, -2, -1, 0, 1, 2, 3, 4}. A = {x ∈ ℤ | x ≤ 4 }.